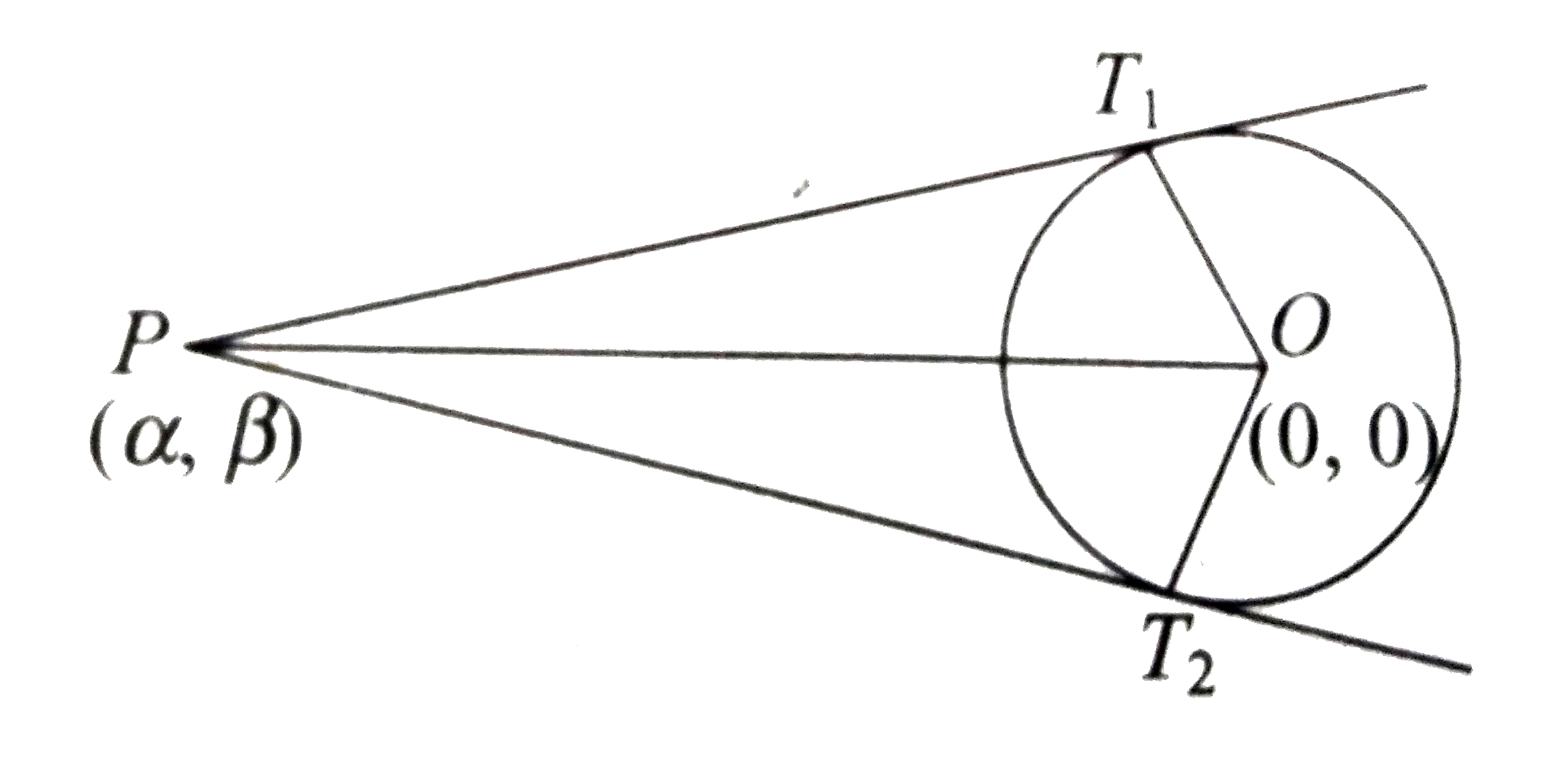
Tangents PT1,andPT2, are drawn from a point P to the circle x2+y2=a2. If the point P lies on the line Px+qy+r=0, then the locus of the centre of circumcircle of the triangle PT1T2 is Solution in Bengali
A. px+qy=r
B. (x−p)2+(y−q)2=r2
C. px+qy=r2
D. 2px+2qy+r=0
The correct Answer is:D
P,T2,Q,T1 are concylic points with PO as diameter.
Thus, the circumcentre of ΔPT1T2 is (α2,β2).
New (α,β) lies on px+qy+r=0
i.e, locus of (α2,β2) is 2px+2qy+r=0.
Topper's Solved these Questions
- CIRCLESBOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLESEXERCISE - Multiple Correct Answers Type
9 Videos
- CIRCLESBOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLESEXERCISE - Comprehension Type
8 Videos
- CIRCLE BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - For problems 3 and 4
2 Videos
- COMPLEX NUMBERSBOOK - CENGAGE PUBLICATIONCHAPTER - COMPLEX NUMBERSEXERCISE - MULTIPLE CORRECT ANSWER TYPE
6 Videos
Similar Questions
The line 3x+6y=k intersects the curve 2x2+3y2=1 at points AandB . The circle on AB as diameter passes through the origin. Then the value of k2 is__________
View Solution
In the diagram as shown, a circle is drawn with centre C(1,1) and radius 1 and a line L. The line L is tangent to the circle at Q. Further L meets the y-axis at R and the x-axis at P in such a way that the angle OPQ equals θ where 0<θ<π2.
Coordinate of Q is
View SolutionPARAGRAPH X Let S be the circle in the xy -plane defined by the equation x2+y2=4. (For Ques. No 15 and 16) Let E1E2 and F1F2 be the chords of S passing through the point P0(1, 1) and parallel to the x-axis and the y-axis, respectively. Let G1G2 be the chord of S passing through P0 and having slope −1 . Let the tangents to S at E1 and E2 meet at E3 , the tangents to S at F1 and F2 meet at F3 , and the tangents to S at G1 and G2 meet at G3 . Then, the points E3, F3 and G3 lie on the curve x+y=4 (b) (x−4)2+(y−4)2=16 (c) (x−4)(y−4)=4 (d) xy=4
View SolutionLet z1 and z2 be two distinct complex numbers and z=(1−t)z1+tz2, for some real number t with 0<t<1 and i=√−1. If arg(w) denotes the principal argument of a non-zero compolex number w, then
View SolutionTangents PT1,andPT2, are drawn from a point P to the circle x2+y2=a2. If the point P line Px+qy+r=0, then the locus of the centre of circumcircle of the triangle PT1T2 is
View SolutionTangents are drawn from P(3,0) to the circle x^(2)+y^(2)=1 touches the circle at points A and B.The equation of locus of the point whose distances from the point P and the line AB are equal is
View SolutionTangents PA and PB are drawn to the circle (x+3)^(2)+(y-4)^(2)=1 from a variable point P on y=sin x. Find the locus of the centre of the circle circumscribing triangle PAB.
View SolutionIf the line px+qy=1 touches the circle x^(2)+y^(2)=r^(2), prove that the point (p,q) lies on the circle x^(2)+y^(2)=r^(-2)
View SolutionTangents from point P are drawn one to each of the circles x2+y2−4x−8y+11=0 and x2+y2−4x−8y+15=0 if the tangents are perpendicular then the locus of P is
View SolutionEquation of any circle passing through the point(s) of intersection of circle S=0 and line L=0 is S+kL=0. Let P(x1,y1) be a point outside the circle x2+y2=a2 and PAandPB be two tangents drawn to this circle from P touching the circle at AandB. On the basis of the above information : If P lies on the px+qy=r, then locus of circumcentre of ΔPAB is : (A) 2px+2qy=r (B) px+qy=r (C) px−qy=r (D) 2px−2py=r
View SolutionA part of the locus of a point P, which is equidstant from two intersecting line as+by+c=0andpx+qy+r=0
View SolutionP is a variable point on the line L=0 . Tangents are drawn to the circles x2+y2=4 from P to touch it at Q and R. The parallelogram PQSR is completed.
If P≡(6,8), then the area of ΔQRS is
View SolutionTangents drawn from the point P(1,8) to the circle x2+y2−6x−4y−11=0 touch the circle at the points A and B. The equation of the circumcircle of the triangle PAB is:
View SolutionTangents drawn from the point P (1, 8) to the circle x2+y2−6x−4y−11=0 touch the circle at the points A and B. The equation of the circumcircle of the triangle PAB is:
View SolutionThe variable point P at the line L = 0 is the circle from the point P x^(2) + y^(2) = 4 The tangent lines drawn at Q touch the circle at Q and R. PQSR is a parallelogram. If P -= (2, 3) If so Delta QRS Is the center of the circumcircle of
View Solution
