P(a,5a) and Q(4a,a) are two points. Two circles are drawn through these points touching the axis of y.
Centre of these circles are at Solution in Bengali
(a,a),(2a,3a)
(205a18,29a3),(5a2,3a)
(3a,29a3),(205a9,29a18)
None of these
The correct Answer is:B
Equation of any circle through the given points is:
(x−a)(x−4a)+(y−5a)(y−a)+λ(4x+3y−19a)=0, for some λ∈R.
As it touches the y-axis, putting x=0 and comparing discriminant of resulting quadratic equation in x to 0, we get
∴(−3a+3λ2)2=9a2−19λa
∴λ=0,−40a9.
∴ The required circles are
x2+y2−5ax−6ay+9a2=0
x2+y2−5ax−6ay+9a2−40a9(4x+3y−19a)=0
Hence centres are (5a2,3a) and (205a18,29a3)
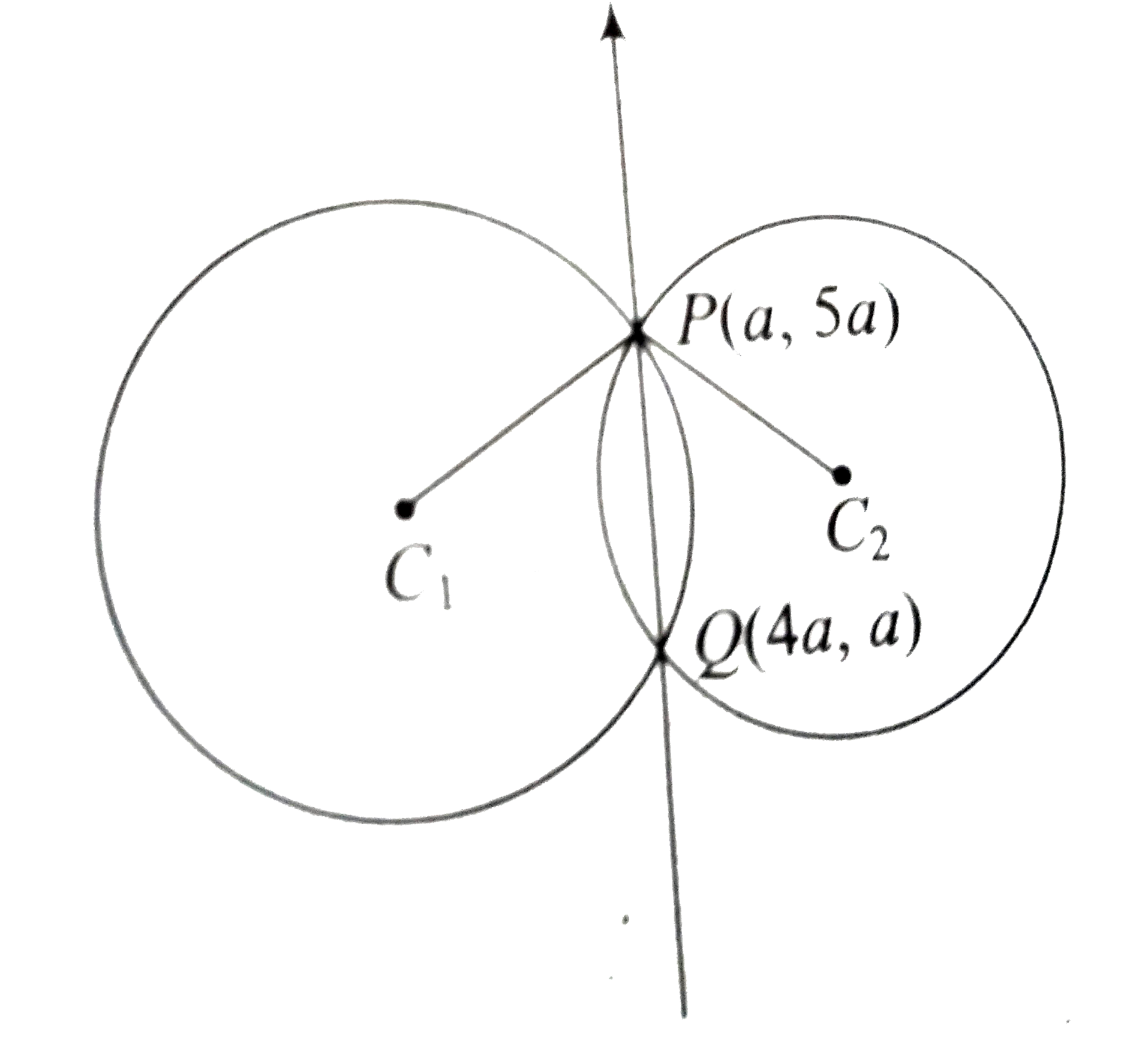
The centres of the given circles are:
C1(205a18,29a3) and C2(5a2,3a)
Now, the angle of intersection θ of these two circles is the angle between the radius vectors a the common point P to the two circles.
i.e., ∠C1PC2=θ
and slope of C2P=5a−3aa−5a2=−43
So, tanθ=84187+431−84187×43=252+748561−336=409
⇒θ=tan−1(409)
Topper's Solved these Questions
- CIRCLESBOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLESEXERCISE - Multiple Correct Answers Type
9 Videos
- CIRCLE BOOK - CENGAGE PUBLICATIONCHAPTER - CIRCLE EXERCISE - For problems 3 and 4
2 Videos
- COMPLEX NUMBERSBOOK - CENGAGE PUBLICATIONCHAPTER - COMPLEX NUMBERSEXERCISE - MULTIPLE CORRECT ANSWER TYPE
6 Videos
Similar Questions
The line 3x+6y=k intersects the curve 2x2+3y2=1 at points AandB . The circle on AB as diameter passes through the origin. Then the value of k2 is__________
View SolutionPARAGRAPH X Let S be the circle in the xy -plane defined by the equation x2+y2=4. (For Ques. No 15 and 16) Let E1E2 and F1F2 be the chords of S passing through the point P0(1, 1) and parallel to the x-axis and the y-axis, respectively. Let G1G2 be the chord of S passing through P0 and having slope −1 . Let the tangents to S at E1 and E2 meet at E3 , the tangents to S at F1 and F2 meet at F3 , and the tangents to S at G1 and G2 meet at G3 . Then, the points E3, F3 and G3 lie on the curve x+y=4 (b) (x−4)2+(y−4)2=16 (c) (x−4)(y−4)=4 (d) xy=4
View SolutionLet z1 and z2 be two distinct complex numbers and z=(1−t)z1+tz2, for some real number t with 0<t<1 and i=√−1. If arg(w) denotes the principal argument of a non-zero compolex number w, then
View SolutionLet S1 and S2 be two circles. S1 is tangent to x-axis and S2 is tangent to y axis and the straight line y = mx touches both circle at their common point. If centre of the circle S1 is (3,1), then radius of circle S2 is p/q, Find the least value of (p+q)
View SolutionTwo circles are drawn through the points (a,5a) and (4a,a) to touch the y-axis.Prove that they intersect at angle tan ^(-1)((40)/(9))
View SolutionA circle is drawn touching the x-axis and centre at the point which is the reflection of (a,b) to theline y-x=0. The equation of the circle is
View Solutionमाना कि केंद्र बिन्दु O वाले वृत्त पर P और Q दो बिन्दू है । यदि वृत्त के दो बिन्दू P और Q से होकर खींची गई स्पर्श रेखाएं A पर मिलती है, तो ∠PAQ=48∘,then ∠APQ कितना होगा ?
View SolutionP(a,5a) and Q(4a,a) are two points. Two circles are drawn through these points touching the axis of y.
Centre of these circles are at
View SolutionIn the adjoining figure, two tangents drawn from external point C to a circle with centre O touches the circel at the point P and Q respectively. A tangent drawn at another point R of the circle intersects CP and CQ at the points A and B respectively. If CP = 7 cm and BC =11 cm, then determine the length of BR.
View SolutionTwo circles touch each other at the point R. PQ is a common tangent to both the circle which touches the circle at the points P and Q. Then ∠PRQ=
View SolutionAB is a diameter of a circle with centre O, P is any point on the circle, the tangent drawn through the point P intersects the two tangents drawn through the points A and B at the points Q and R respectively. If the radius of the circle be r, then prove that PQ.PR=r2.
View Solutionएक चर वृत्त एक स्थिर बिन्दु A(p,q) से गुजरता है तथा x- अक्ष को स्पर्श करता है । इस वृत्त के बिन्दु A से गुजरने वाले व्यास के दूसरे सिरे का बिन्दुपथ है :
View SolutionP and Q are two points on circle with centre O. The point R is on the minor arcPQ. The tangents from P and Q meet at S. If ∠PSQ=20∘ them ∠PRQ is-
View SolutionThe angle between the tangents drawn from point A to a circle 60^(0) And if the circle of two tangents touches at points P, Q Delta APQ
View SolutionTwo circles are drawn through the points (1,5) and (4,1) to touch the axis of y. Find the angle at which they intersect.
View SolutionP(a,5a) and Q(4a,a) are two points. Two circles are drawn through these points touching the axis of y.
Centre of these circles are at
View Solution
