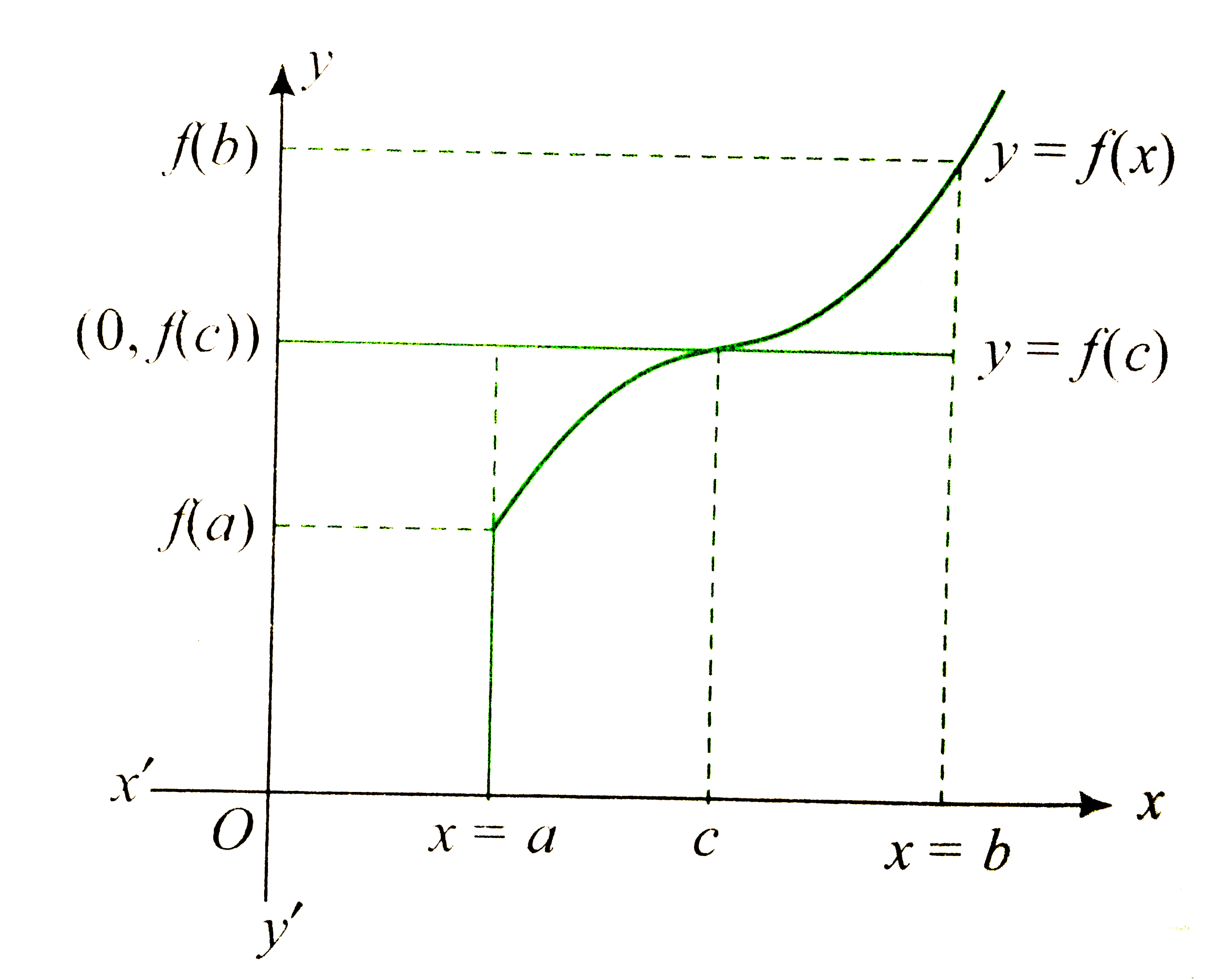
[Bengali] If y=f(x) is a monotonic function in (a,b), then the area bo

Match the following lists :
ocr_image

The area enclosed by the curve $c : y = x \sqrt{9 - x^{2}} (x \geq 0)$ and the x-axis is________

Let $A (k)$ be the area bounded by the curves $y = x^{2} - 3$ and $y = k x + 2$ The range of $A (k)$ is $(\frac{10 \sqrt{5}}{3}, \infty)$ The range of $A (k)$ is $(\frac{20 \sqrt{5}}{3}, \infty)$ If function $k \to A (k)$ is defined for $k \in [- 2, \infty$ ), then $A (k)$ is many-one function. The value of $k$ for which area is minimum is $1 .$

View Solution

Prove that for $λ > 1$ , the equation $x log x + x = λ$ has least one solution in $[1, λ]$ .

View Solution

Consider a $G . P .$ with first term ${(1 + x)}^{n}$ , $| x | < 1$ , common ratio $\frac{1 + x}{2}$ and number of terms $(n + 1)$ . Let $' S'$ be sum of all the terms of the $G . P .$ , then
The coefficient of $x^{n}$ is $' S'$ is

View Solution

Let the definite integral be defined by the formula $\int_{a}^{b} f (x) d x = \frac{b - a}{2} (f (a) + f (b))$ . For more accurate result, for $c ε (a, b),$ we can use $\int_{a}^{b} f (x) d x = \int_{a}^{c} f (x) d x + \int_{c}^{b} f (x) d x = F (c)$ so that for $c = \frac{a + b}{2}$ we get $\int_{a}^{b} f (x) d x = \frac{b - a}{4} (f (a) + f (b) + 2 f (c))$ .
If $f'' (x) < 0 \forall x ε (a, b)$ and $c$ is $a$ point such that $a < c < b$ , and $(c, f (c))$ is the point lying on the curve for which $F (c)$ is maximum then $f' (c)$ is equal to

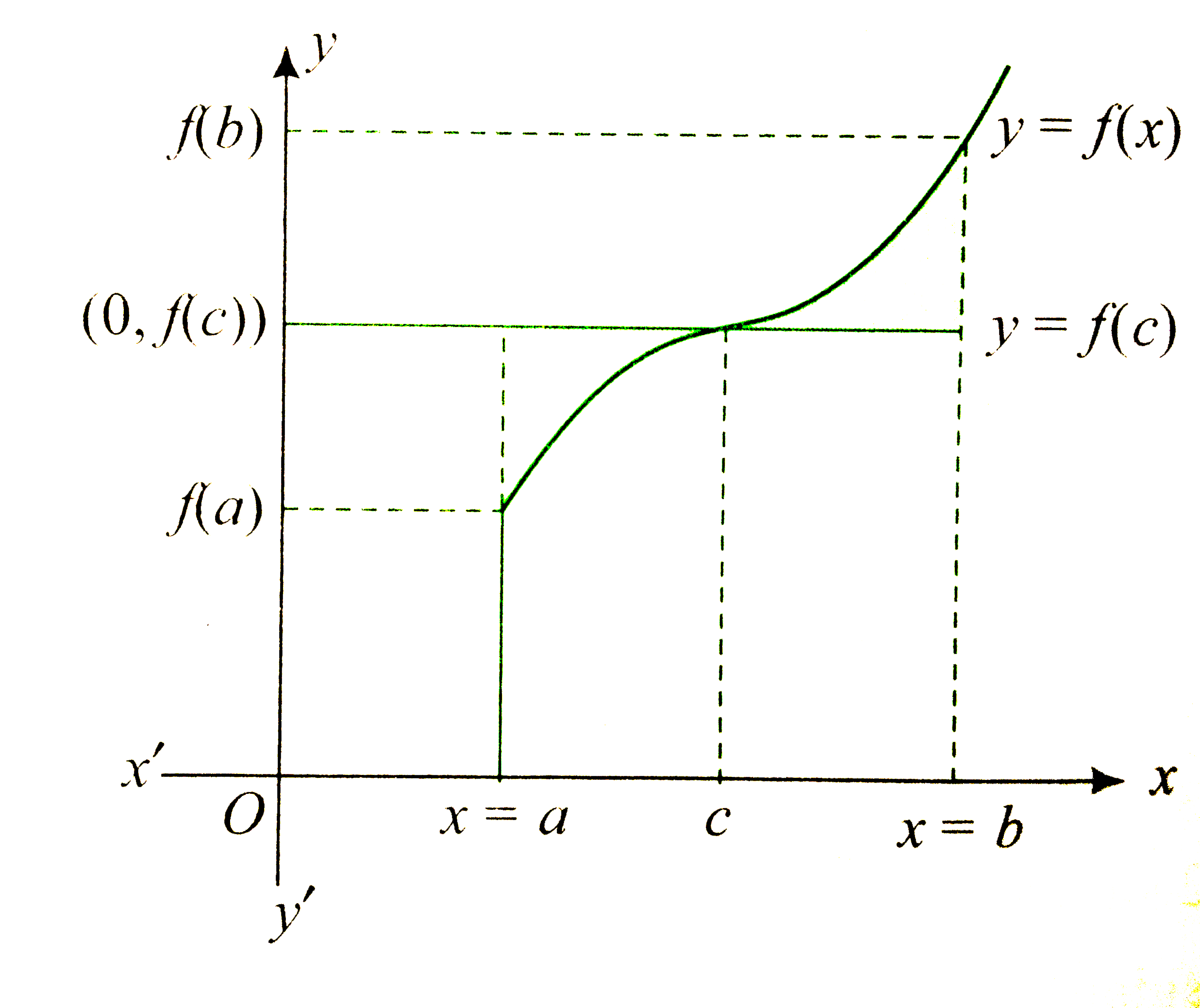
View Solution

If y=f(x) is a monotonic function in (a,b), then the area bounded by the ordinates at $x = a, x = b, y = f (x) and y = f (c) (where c \in (a, b)) is minimum when c = \frac{a + b}{2}$ .
$Proof : A = \int_{a}^{c} (f (c) - f (x)) d x + \int_{c}^{b} (f (c)) d x$
$= f (c) (c - a) - \int_{a}^{c} (f (x)) d x + \int_{a}^{b} (f (x)) d x - f (c) (b - c)$
$\Rightarrow A = [2 c - (a + b)] f (c) + \int_{c}^{b} (f (x)) d x - \int_{a}^{c} (f (x)) d x$
ocr_image
Differentiating w.r.t. c, we get
$\frac{d A}{d c} = [2 c - (a + b)] f' (c) + 2 f (c) + 0 - f (c) - (f (c) - 0)$
For maxima and minima , $\frac{d A}{d c} = 0$
$\Rightarrow f' (c) [2 c - (a + b)] = 0 (a s f' (c) \neq 0)$
Hence, $c = \frac{a + b}{2}$
$Also for c < \frac{a + b}{2}, \frac{d A}{d c} < 0 and for c > \frac{a + b}{2}, \frac{d A}{d c} > 0$
Hence, A is minimum when $c = \frac{a + b}{2}$ .
If the area bounded by $f (x) = \frac{x^{3}}{3} - x^{2} + a$ and the straight lines x=0, x=2, and the x-axis is minimum, then the value of a is

View Solution

If y=f(x) is a monotonic function in (a,b), then the area bounded by the ordinates at $x = a, x = b, y = f (x) and y = f (c) (where c \in (a, b)) is minimum when c = \frac{a + b}{2}$ .
$Proof : A = \int_{a}^{c} (f (c) - f (x)) d x + \int_{c}^{b} (f (c)) d x$
$= f (c) (c - a) - \int_{a}^{c} (f (x)) d x + \int_{a}^{b} (f (x)) d x - f (c) (b - c)$
$\Rightarrow A = [2 c - (a + b)] f (c) + \int_{c}^{b} (f (x)) d x - \int_{a}^{c} (f (x)) d x$
ocr_image
Differentiating w.r.t. c, we get
$\frac{d A}{d c} = [2 c - (a + b)] f' (c) + 2 f (c) + 0 - f (c) - (f (c) - 0)$
For maxima and minima , $\frac{d A}{d c} = 0$
$\Rightarrow f' (c) [2 c - (a + b)] = 0 (a s f' (c) \neq 0)$
Hence, $c = \frac{a + b}{2}$
$Also for c < \frac{a + b}{2}, \frac{d A}{d c} < 0 and for c > \frac{a + b}{2}, \frac{d A}{d c} > 0$
Hence, A is minimum when $c = \frac{a + b}{2}$ .
The value of the parameter a for which the area of the figure bounded by the abscissa axis, the graph of the function $y = x^{3} + 3 x^{2} + x + a$ , and the straight lines, which are parallel to the axis of ordinates and cut the abscissa axis at the point of extremum of the function, which is the least, is

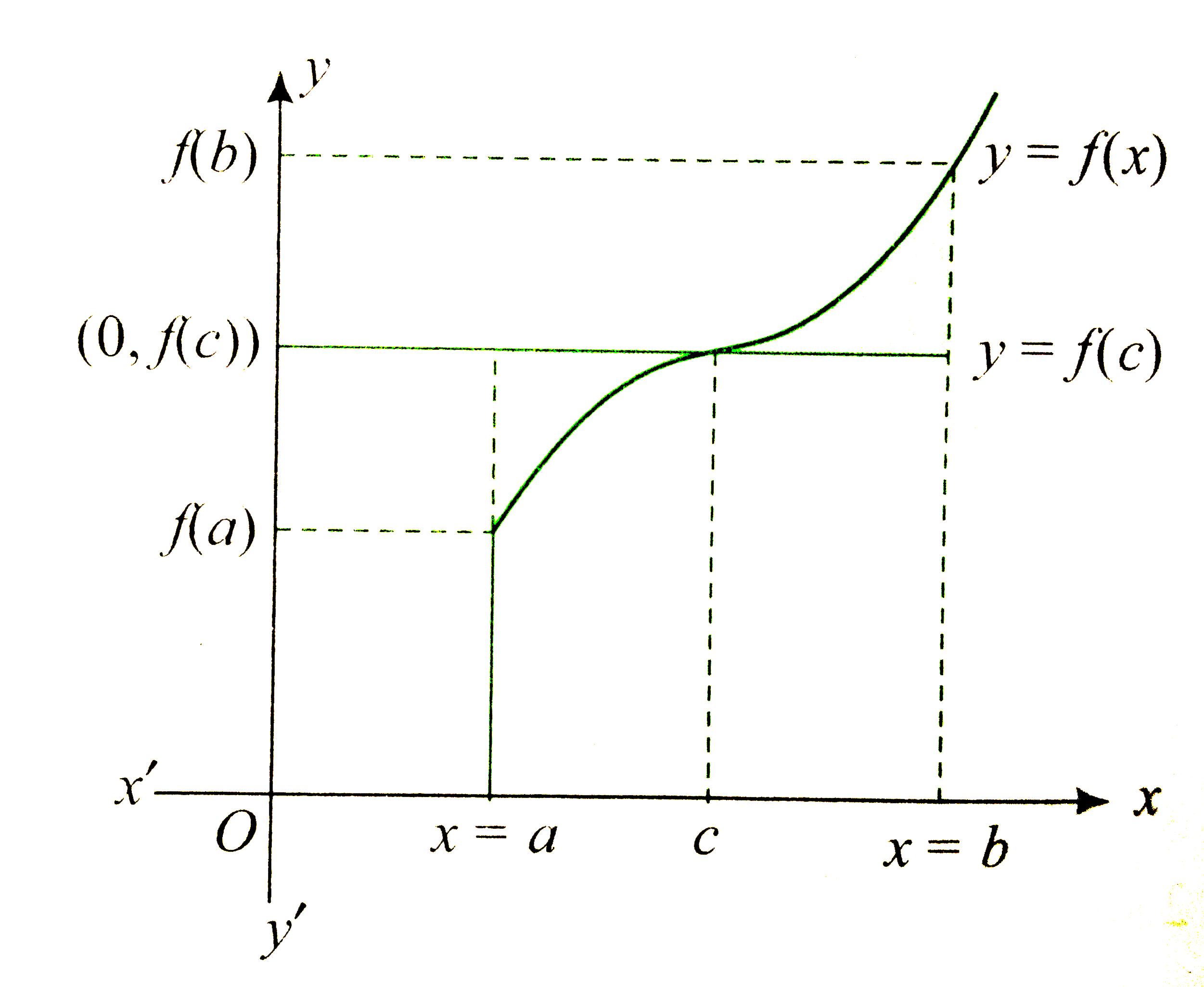
View Solution

If y=f(x) is a monotonic function in (a,b), then the area bounded by the ordinates at $x = a, x = b, y = f (x) and y = f (c) (where c \in (a, b)) is minimum when c = \frac{a + b}{2}$ .
$Proof : A = \int_{a}^{c} (f (c) - f (x)) d x + \int_{c}^{b} (f (c)) d x$
$= f (c) (c - a) - \int_{a}^{c} (f (x)) d x + \int_{a}^{b} (f (x)) d x - f (c) (b - c)$
$\Rightarrow A = [2 c - (a + b)] f (c) + \int_{c}^{b} (f (x)) d x - \int_{a}^{c} (f (x)) d x$
ocr_image
Differentiating w.r.t. c, we get
$\frac{d A}{d c} = [2 c - (a + b)] f' (c) + 2 f (c) + 0 - f (c) - (f (c) - 0)$
For maxima and minima , $\frac{d A}{d c} = 0$
$\Rightarrow f' (c) [2 c - (a + b)] = 0 (a s f' (c) \neq 0)$
Hence, $c = \frac{a + b}{2}$
$Also for c < \frac{a + b}{2}, \frac{d A}{d c} < 0 and for c > \frac{a + b}{2}, \frac{d A}{d c} > 0$
Hence, A is minimum when $c = \frac{a + b}{2}$ .
If the area enclosed by $f (x) = sin x + cos x, y = a$ between two consecutive points of extremum is minimum, then the value of a is

View Solution

If $\int_{0}^{b - c} f (x + c) d x = a \int_{b}^{c} f (x) d x$ , then a =

View Solution

If $\int_{0}^{b - c} f (x + c) d x = k \int_{b}^{c} f (x) d x$ then $k =$

View Solution

Let the definite integral be defined by the formula $\int_{a}^{b} f (x) d x = \frac{b - a}{2} (f (a) + f (b))$ . For more accurate result, for $c ε (a, b),$ we can use $\int_{a}^{b} f (x) d x = \int_{a}^{c} f (x) d x + \int_{c}^{b} f (x) d x = F (c)$ so that for $c = \frac{a + b}{2}$ we get $\int_{a}^{b} f (x) d x = \frac{b - a}{4} (f (a) + f (b) + 2 f (c))$ .
If $f'' (x) < 0 \forall x ε (a, b)$ and $c$ is $a$ point such that $a < c < b$ , and $(c, f (c))$ is the point lying on the curve for which $F (c)$ is maximum then $f' (c)$ is equal to

View Solution

If y=f(x) is a monotonic function in (a,b), then the area bounded by the ordinates at $x = a, x = b, y = f (x) and y = f (c) (where c \in (a, b)) is minimum when c = \frac{a + b}{2}$ .
$Proof : A = \int_{a}^{c} (f (c) - f (x)) d x + \int_{c}^{b} (f (c)) d x$
$= f (c) (c - a) - \int_{a}^{c} (f (x)) d x + \int_{a}^{b} (f (x)) d x - f (c) (b - c)$
$\Rightarrow A = [2 c - (a + b)] f (c) + \int_{c}^{b} (f (x)) d x - \int_{a}^{c} (f (x)) d x$
ocr_image
Differentiating w.r.t. c, we get
$\frac{d A}{d c} = [2 c - (a + b)] f' (c) + 2 f (c) + 0 - f (c) - (f (c) - 0)$
For maxima and minima , $\frac{d A}{d c} = 0$
$\Rightarrow f' (c) [2 c - (a + b)] = 0 (a s f' (c) \neq 0)$
Hence, $c = \frac{a + b}{2}$
$Also for c < \frac{a + b}{2}, \frac{d A}{d c} < 0 and for c > \frac{a + b}{2}, \frac{d A}{d c} > 0$
Hence, A is minimum when $c = \frac{a + b}{2}$ .
If the area bounded by $f (x) = \frac{x^{3}}{3} - x^{2} + a$ and the straight lines x=0, x=2, and the x-axis is minimum, then the value of a is

View Solution

If y=f(x) is a monotonic function in (a,b), then the area bounded by the ordinates at $x = a, x = b, y = f (x) and y = f (c) (where c \in (a, b)) i s min i μ m w h e n$
$c = \frac{a + b}{2}$ .
$Proof : A = \int_{a}^{c} (f (c) - f (x)) d x + \int_{c}^{b} (f (c)) d x$
$= f (c) (c - a) - \int_{a}^{c} (f (x)) d x + \int_{a}^{b} (f (x)) d x - f (c) (b - c)$
$\Rightarrow A = [2 c - (a + b)] f (c) + \int_{c}^{b} (f (x)) d x - \int_{a}^{c} (f (x)) d x$
ocr_image
Differentiating w.r.t. c, we get
$\frac{d A}{d c} = [2 c - (a + b)] f' (c) + 2 f (c) + 0 - f (c) - (f (c) - 0)$
For maxima and minima , $\frac{d A}{d c} = 0$
$\Rightarrow f' (c) [2 c - (a + b)] = 0 (a s f' (c) \neq 0)$
Hence, $c = \frac{a + b}{2}$
$Also for c < \frac{a + b}{2}, \frac{d A}{d c} < 0 and for c > \frac{a + b}{2}, \frac{d A}{d c} > 0$
Hence, A is minimum when $c = \frac{a + b}{2}$ .
The value of the parameter a for which the area of the figure bounded by the abscissa axis, the graph of the function $y = x^{3} + 3 x^{2} + x + a$ , and the straight lines, which are parallel to the axis of ordinates and cut the abscissa axis at the point of extremum of the function, which is the least, is

View Solution

If y=f(x) is a monotonic function in (a,b), then the area bounded by the ordinates at $x = a, x = b, y = f (x) and y = f (c) (where c \in (a, b)) is minimum when c = \frac{a + b}{2}$ .
$Proof : A = \int_{a}^{c} (f (c) - f (x)) d x + \int_{c}^{b} (f (c)) d x$
$= f (c) (c - a) - \int_{a}^{c} (f (x)) d x + \int_{a}^{b} (f (x)) d x - f (c) (b - c)$
$\Rightarrow A = [2 c - (a + b)] f (c) + \int_{c}^{b} (f (x)) d x - \int_{a}^{c} (f (x)) d x$
ocr_image
Differentiating w.r.t. c, we get
$\frac{d A}{d c} = [2 c - (a + b)] f' (c) + 2 f (c) + 0 - f (c) - (f (c) - 0)$
For maxima and minima , $\frac{d A}{d c} = 0$
$\Rightarrow f' (c) [2 c - (a + b)] = 0 (a s f' (c) \neq 0)$
Hence, $c = \frac{a + b}{2}$
$Also for c < \frac{a + b}{2}, \frac{d A}{d c} < 0 and for c > \frac{a + b}{2}, \frac{d A}{d c} > 0$
Hence, A is minimum when $c = \frac{a + b}{2}$ .
If the area enclosed by $f (x) = sin x + cos x, y = a$ between two consecutive points of extremum is minimum, then the value of a is

View Solution

Prove that $\int_{a}^{b} f (x) d x = \int_{a}^{c} f (x) d x + \int_{c}^{b} f (x) d x$ , $a < c < b$

View Solution

The correct Answer is:C

$f (x) = x^{3} + 3 x^{2} + x + a$
$f' (x) = 3 x^{2} + 6 x + 1 = 0$
$\Rightarrow x = - 1 \pm \frac{\sqrt{6}}{3} .$
Hence, f(x) cuts the x-axis at
$\frac{1}{2} [(- 1 + \frac{\sqrt{6}}{3}) + (- 1 - \frac{\sqrt{6}}{3})] = - 1 .$
$f (- 1) = - 1 + 3 - 1 + a = 0$
$a = - 1 .$

Topper's Solved these Questions

Similar Questions

CENGAGE PUBLICATION-AREA-Linkded Comprehension Type

The correct Answer is:C

f(x)=x3+3x2+x+a f'(x)=3x2+6x+1=0 ⇒ x=−1±√63. Hence, f(x) cuts the x-axis at 12[(−1+√63)+(−1−√63)]=−1. f(−1)=−1+3−1+a=0 a=−1.

Topper's Solved these Questions

Similar Questions

CENGAGE PUBLICATION-AREA-Linkded Comprehension Type

$f (x) = x^{3} + 3 x^{2} + x + a$
$f' (x) = 3 x^{2} + 6 x + 1 = 0$
$\Rightarrow x = - 1 \pm \frac{\sqrt{6}}{3} .$
Hence, f(x) cuts the x-axis at
$\frac{1}{2} [(- 1 + \frac{\sqrt{6}}{3}) + (- 1 - \frac{\sqrt{6}}{3})] = - 1 .$
$f (- 1) = - 1 + 3 - 1 + a = 0$
$a = - 1 .$