The area of the closed figure bounded by y=x22−2x+2 and the tangents to it at (1,12)and(4,2) is 98sq.units (b) 38sq.units 32sq.units (d) 94sq.unitsSolution in Bengali
9/8 sq. units
3/8 sq. units
3/2 sq. units
9/4 sq. units
The correct Answer is:A
y=x22−2x+2=(x−2)22,
dydx=x−2,(dydx)x=1=−1,(dydx)x=4=2
Thus, tangent at (1,1/2) is y−1/2=−1(x−1)or2x+2y−3=0
Tangent at (4,2) is y−2=2(x−4)or2x−y−6=0
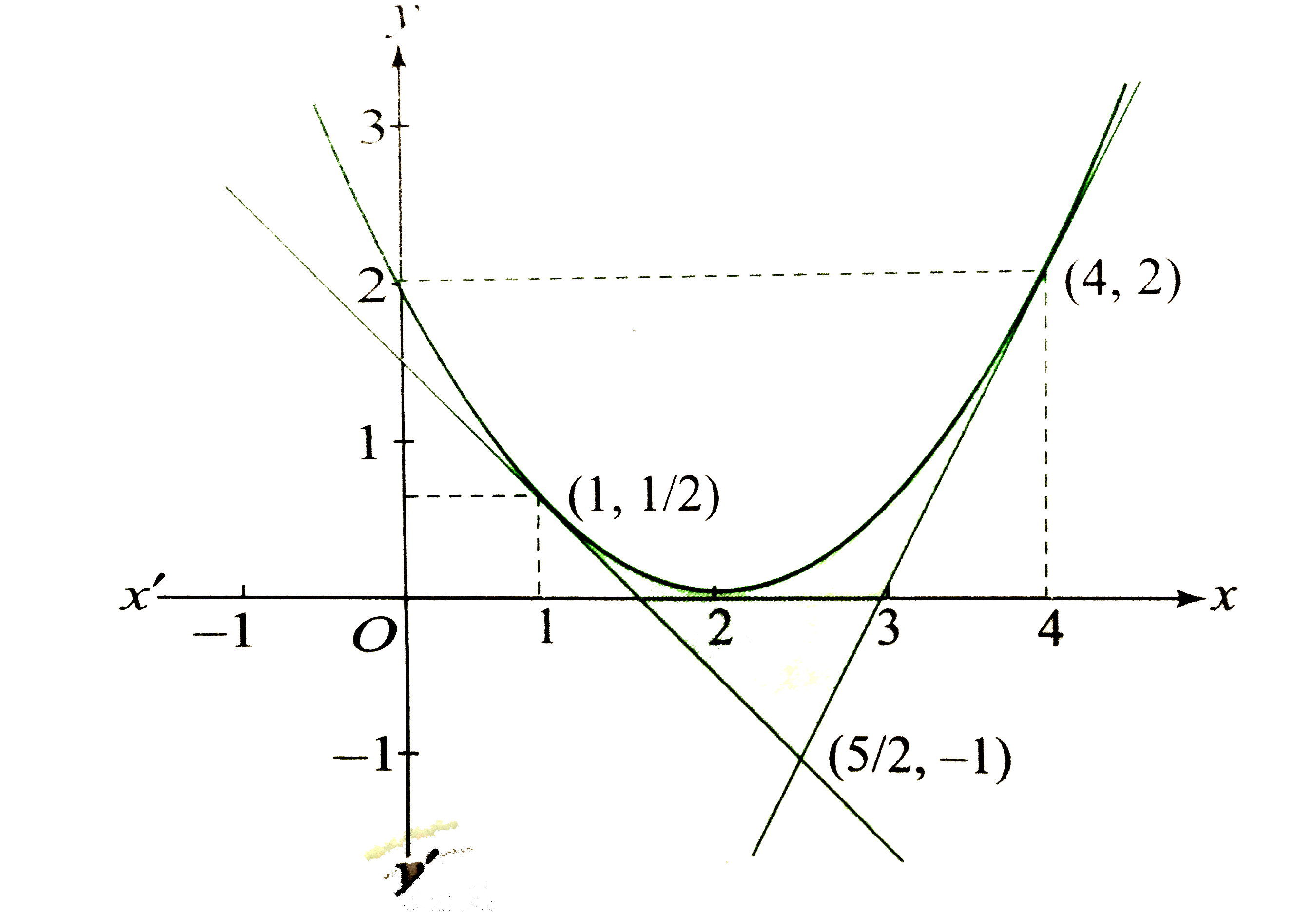
Hence, A=5/2∫1(x22−2x+2−3−2x2)dx+4∫5/2(x22−2x+2−(2x−6))dx
=4∫1(x22−2x+2)dx−5/2∫1(3−2x2)dx−4∫5/2(2x−6)dx
=(x36−x2+2x)41−12(3x−x2)5/21−(x2−6x)45/2
=(633−15+6)−12(3×32−(254−1))−((16−254)−6(4−52))
=32−12(92−214)−(394−6(32))
=98 sq. units
Topper's Solved these Questions
- AREABOOK - CENGAGE PUBLICATIONCHAPTER - AREAEXERCISE - Multiple Correct Answers Type
13 Videos
- AREABOOK - CENGAGE PUBLICATIONCHAPTER - AREAEXERCISE - Linkded Comprehension Type
21 Videos
- AREABOOK - CENGAGE PUBLICATIONCHAPTER - AREAEXERCISE - Concept Application Exercise 9.3
7 Videos
- APPLICATIONS OF DERIVATIVESBOOK - CENGAGE PUBLICATIONCHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Subjective Type
2 Videos
- BINOMIAL THEOREMBOOK - CENGAGE PUBLICATIONCHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension
11 Videos
Similar Questions
Let A(k) be the area bounded by the curves y=x2−3 and y=kx+2 The range of A(k) is (10√53,∞) The range of A(k) is (20√53,∞) If function k→A(k) is defined for k∈[−2,∞ ), then A(k) is many-one function. The value of k for which area is minimum is 1.
View SolutionLet Ar be the area of the region bounded between the curves y2=(e−kr)x(where k>0,r∈N) and the line y=mx(where m≠0), k and m are some constants
A1,A2,A3,… are in G.P. with common ratio
View SolutionFind the continuous function f where (x4−4x2)≤f(x)≤(2x2−x3) such that the area bounded by y=f(x),y=x4−4x2. then y-axis, and the line x=t, where (0≤t≤2) is k times the area bounded by y=f(x),y=2x2−x3,y-axis , and line x=t(where0≤t≤2).
View SolutionProve that for λ>1, the equation xlogx+x=λ has least one solution in [1,λ].
View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is
View SolutionThe area enclosed between the curves y=(log)e(x+e),x=(log)e(1y), and the x-axis is 2sq.units (b) 1sq.units 4sq.units (d) none of these
View SolutionThe area of the closed figure bounded by x=−1,x=2,and y={−x2+2,x≤12x−1,x>1andtheabscissaaξsis 163sq.units (b) 103sq.units 133sq.units (d) 73sq.units
View SolutionThe area enclosed between the curves y=(log)e(x+e),x=(log)e(1y), and the x-axis is (a) 2sq.units (b) 1sq.units (c) 4sq.units (d) none of these
View SolutionThe area bounded by the loop of the curve 4y2=x2(4−x2) is (a)7/3 sq. units (b) 83sq.unites 113sq.units (d) 163sq.units
View SolutionA particle of mass m is moving anticlockwise, in a circle of radius R in x−y plane with centre at (R,0) with a constant speed v2. If is located at point (2R,0) at time t=0. A man starts moving with a velocity v1 along the positive y-axis from origin at t=0. Calculate the linear momentum of the particle w.r.t. man as a function of time.
View SolutionThe area of the closed figure bounded by x=−1,x=2,and y={−x2+2,x≤12x−1,x>1and the ascissa axis is (a)163sq.units (b) 103sq.units (c)133sq.units (d) 73sq.units
View SolutionArea of the triangle formed by the line x+y=3 and the angle bisectors of the pairs of straight lines x2−y2+2y=1 is 2sq.units (b) 4sq.units 6sq.units (d) 8sq.units
View SolutionArea of the triangle formed by the line x+y=3 and angle bisectors of the pair of straight lines x2−y2+2y=1 is 2sq.units b. 4sq.units c. 6sq.units d. 8sq.units
View SolutionThe area of the closed figure bounded by y=x22−2x+2 and the tangents to it at (1,12)and(4,2) is 98sq.units (b) 38sq.units 32sq.units (d) 94sq.units
View SolutionThe area of the loop of the curve ay2=x2(a−x) is 4a2sq.units (b) 8a215sq.units 16a29sq.units (d) None of these
View SolutionThe area bounded by the y=2−x2and x+y=0 is (a)72sq.units b. 92 sq.units c. 9 sq.units d. none of these
View Solution
