x3+5x2+px+q=0 and x3+7x2+px+r=0 have two roos in common. If their third roots are γ1 and γ2 , respectively, then |γ1+γ2|= ?Solution in Bengali
10
12
13
42
The correct Answer is:B
(b) Let the roots of x3+5x2+px+q=0 are α1, β1, γ1……..(i)
Then roots of the x3+7x2+px+r=0 is α1, β1, γ2……..(ii)
From (i)-(ii), −2x2+q−r=0
This equation has roots α1, β1
⇒α1+β1=0
Now from (i)α1+β1+γ1=−5⇒γ1=−5
From (ii)α1+β1+γ2=−7⇒γ2=−7
∴γ1+γ2=−12
∴|γ1+γ2|=12
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - Multiple Correct Answer6videos
THEORY OF EQUATIONS
- BOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - ILLUSTRATION121videos
THEORY OF EQUATIONS
- BOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )1videos
THEORY OF EQUATIONS
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - ARCHIVES (NUMERICAL VALUE TYPE)1videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - All Questions291videos
THREE DIMENSIONAL GEOMETRY
Similar Questions
If c≠0 and the equation p/(2x)=a/(x+c)+b/(x−c) has two equal roots, then p can be a. (√a−√b)2 b. (√a+√b)2 c. a+b d. a−b
03:33View Solutionf(x) be a quadratic polynomial f(x)=(x−1)(ax+b) satisfying f(2)+f(4)=0.
If unity is one root of f(x) = 0 then find the other root.01:30View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
02:29View SolutionFor a point P in the plane, let d1(P)andd2(P) be the distances of the point P from the lines x−y=0andx+y=0 respectively. The area of the region R consisting of all points P lying in the first quadrant of the plane and satisfying 2≤d1(P)+d2(P)≤4, is
06:36View SolutionFind the angle between the line whose direction cosines are given by l+m+n=0andl2+m2−n2=0.
03:23View Solutionx^(3)+5x^(2)+px+q=0 and x^(3)+7x^(2)+px+r=0 have two roots in common.If their third roots are gamma_(1) and gamma_(2) respectively,then | gamma_(1)+gamma_(2)|=
06:05View SolutionThe coefficient of real expansion of liquid is gamma_(R) and the coefficient of appareent expansion of the liquid is gamma_(A) . The coefficient of cubical expansion of the vessel is gamma . If gamma_(R) : gamma_(A) = 4:1 then gamma_(A) : gamma is
06:05View SolutionOrder of no. of revolutions/sec gamma_(1),gamma_(2),gamma_(3) and gamma_(4) for I, II, III and IV orbits is:
View Solutionx3+5x2+px+q=0 and x3+7x2+px+r=0 have two roos in common. If their third roots are γ1 and γ2 , respectively, then |γ1−γ2|=
03:39View SolutionIF the equations x3+5x2+px+q=0andx3+7x2+px+r=0 have two roots in common, then the product of two non-common roots of two equations, is
03:28View SolutionLet α,β,γ be the roots of x3+x+10=0 and α1=α+βγ2,β1=β+γα2,γ1=γ+αβ2. Then, the value of (α31+β31+γ31)−110(α21+β21+γ21) is
View SolutionLet α,β,γ be the roots of x3+x+10=0 and α1=α+βγ2,β1=β+γα2,γ1=γ+αβ2. Then, the value of (α31+β31+γ31)−110(α21+β21+γ21) is
01:36View SolutionLet α,β,γ be the roots of x3+x+10=0. Write α1=α+βα2,β1=β+γα2,γ1=γ+αβ2. Then the value of (α31+β31+γ31)−110(α21+β21+γ21) is
07:13View SolutionThe following graph represents the variation of photo current with anode potential for a metal surface . Here l1,l2 and l3 represents intensities and γ1,γ2, γ3 respectively , them .
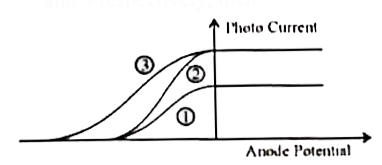 07:25View Solution
07:25View Solutionx3+5x2+px+q=0 and x3+7x2+px+r=0 have two roos in common. If their third roots are γ1 and γ2 , respectively, then |γ1+γ2|=
03:41View SolutionγA of liquid is 7/8 of γR of liquid. αgof vessel is
05:04View Solution
