All the values of 'a' for which the quadratic expression ax2+(a−2)x−2 is negative for exactly two integral values of x may lie in (a) [1,32] (b) [32,2) (c) [1,2) (d) [−1,2)Solution in Bengali
[−1,1]
[1,2)
[−1,1]
[-2,-1])
The correct Answer is:B
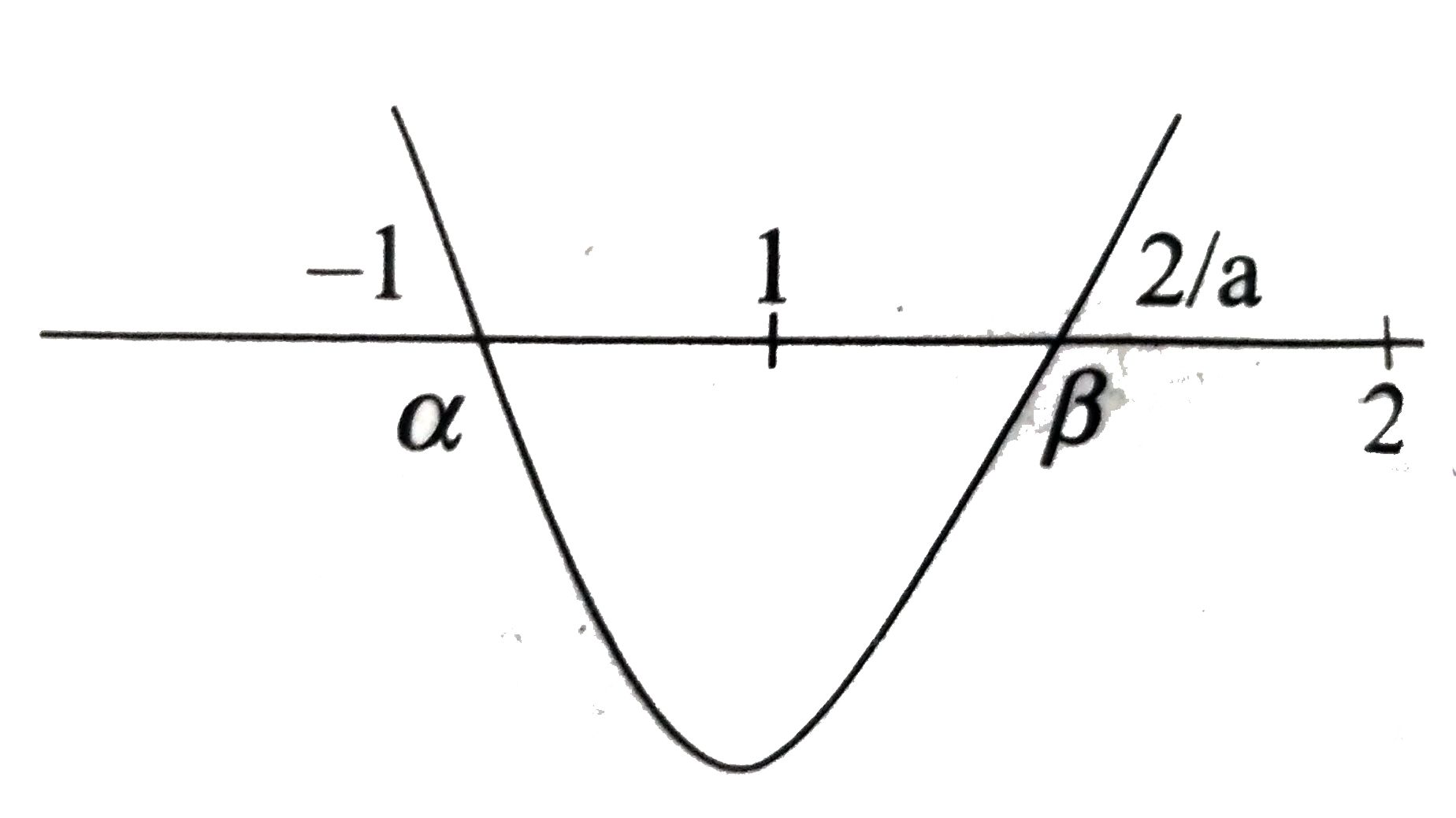
(b) Let f(x)=ax2+(a−2)x−2
f(x) is negative for two integral values of x, so graph should be vertically upward parabola
i.e., a>0
Let two roots of f(x)=0 are α and β, then
α, β=−(a−2)±(a+2)2a
⇒a=−1, β=2a
⇒1<β≤2
⇒1<2a≤2
⇒a∈[1,2)
Topper's Solved these Questions
- THEORY OF EQUATIONSBOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - Multiple Correct Answer
6 Videos
- THEORY OF EQUATIONSBOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - ILLUSTRATION
121 Videos
- THEORY OF EQUATIONSBOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )
1 Videos
- STRAIGHT LINESBOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - ARCHIVES (NUMERICAL VALUE TYPE)
1 Videos
- THREE DIMENSIONAL GEOMETRYBOOK - CENGAGE PUBLICATIONCHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - All Questions
291 Videos
Similar Questions
If c≠0 and the equation p/(2x)=a/(x+c)+b/(x−c) has two equal roots, then p can be a. (√a−√b)2 b. (√a+√b)2 c. a+b d. a−b
View Solutionf(x) be a quadratic polynomial f(x)=(x−1)(ax+b) satisfying f(2)+f(4)=0.
If unity is one root of f(x) = 0 then find the other root.
View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
View SolutionFor a point P in the plane, let d1(P)andd2(P) be the distances of the point P from the lines x−y=0andx+y=0 respectively. The area of the region R consisting of all points P lying in the first quadrant of the plane and satisfying 2≤d1(P)+d2(P)≤4, is
View SolutionFind the angle between the line whose direction cosines are given by l+m+n=0andl2+m2−n2=0.
View SolutionAll the values of 'a' for which the quadratic expression ax2+(a−2)x−2 is negative for exactly two integral values of x may lie in [1,32] (b) [32,2) [1,2) (d) [−1,2)
View SolutionThe interval [a,b] such that the value of ∫ba(2+x−x2)dx is maximum is a.[−1,2] b. [−2,1] c. [−2,−1] d. [1,2]
View SolutionThe values of 'a' for which the quadraic expression ax2+(a−2)x−2 is negative for exactly two integral values of x, belongs to
View SolutionThe value of 'a' for which the quadratic expression ax^(2)+|2a-3|x-6 is positive for exactly two integral values of x is
View SolutionAll the values of 'a' for which the quadratic expression ax2+(a−2)x−2 is negative for exactly two integral values of x may lie in (a) [1,32] (b) [32,2) (c) [1,2) (d) [−1,2)
View SolutionIf set of values a for which f(x)=ax2−(3+2a)x+6,a≠0 is positive for exactly three distinct negative integral values of x is (c,d] , then the value of (c2+4|d|) is equal to ________.
View SolutionThe values of 'a' for which the quadraic expression ax2+(a−2)x−2 is negative for exactly two integral values of x, belongs to
View Solutiona' के वे सभी मान, जिनके लिए द्विघात व्यंजक ax2+(a−2)x−2,x के ठीक दो पूर्णाक मानों के लिए ऋणात्मक होगा, निम्न अंतराल में हो सकते है
View Solutiona' के वे सभी मान जिसके लिए दिघात व्यंजक ax2+(a−2)x−2,x के ठीक दो पूर्णाक मानो के लिए ऋणात्मक होगा निम्न अंतराल में हो सकते है
View SolutionThe values of 'a' for which the quadraic expression ax2+(a−2)x−2 is negative for exactly two integral values of x, belongs to
View SolutionFind the values of a, for which the quadratic expression ax2+(a−2)x−2 is negative for exactly two integral values of x.
View Solution
