Consider triangle AOB in the x−y plane, where A≡(1,0,0),B≡(0,2,0)andO≡(0,0,0). The new position of O, when triangle is rotated about side AB by 900 can be a. (45,35,2√5) b. (−35,√25,2√5) c. (45,25,2√5) d. (45,25,1√5)Solution in Bengali
(45,35,2√5)
(−35,√25,2√5)
(45,25,2√5)
(45,25,1√5)
The correct Answer is:c
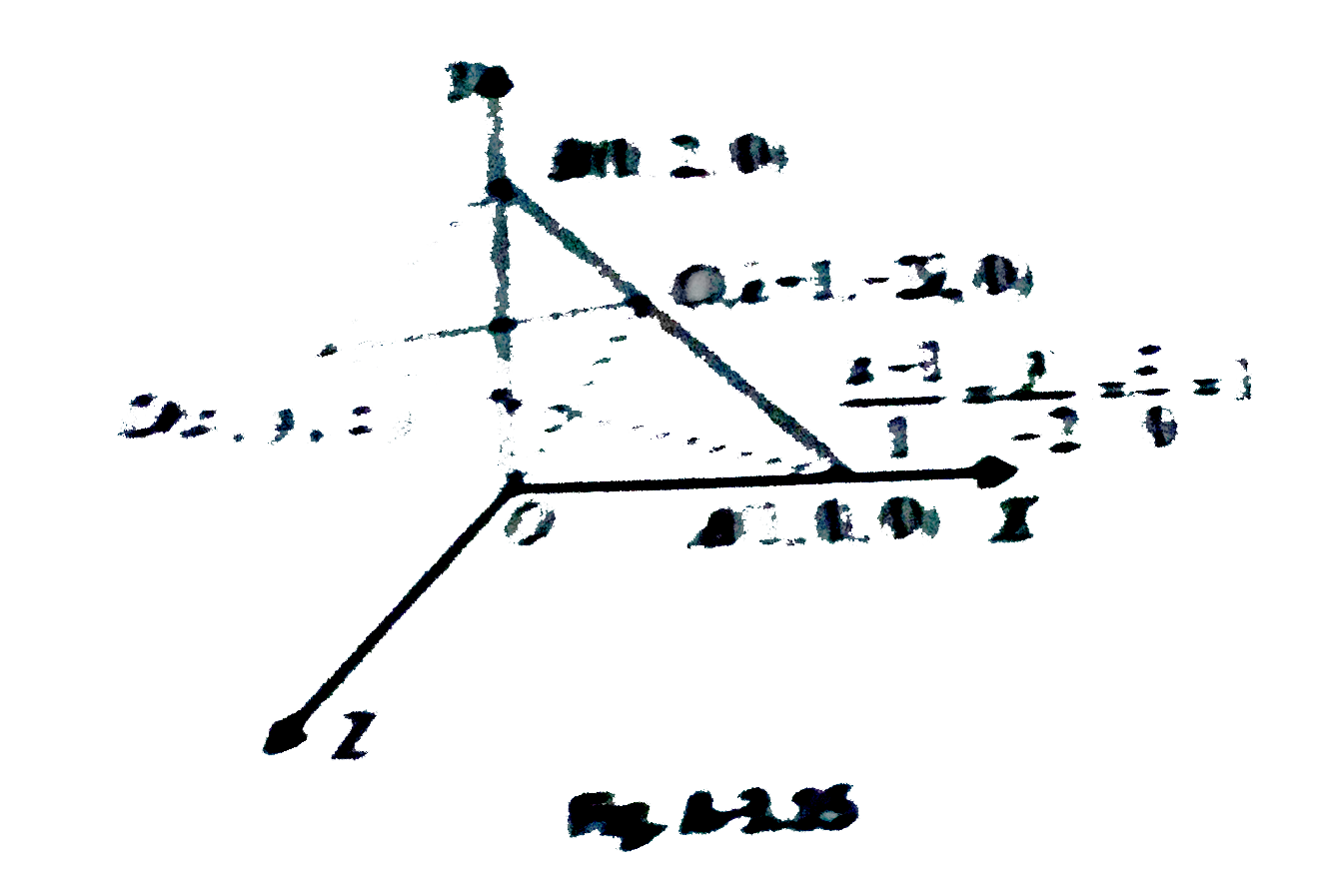
Equation of line AB is x−11=y−2=z0=λ
Now AB⊥OC⇒1(λ+1)+(−2λ)(−2)=0
or 5λ=−1orλ=−15
⇒ C is (45,25,0). Now
x21+(y1−2)2+z21=4 (i)
and (x1−1)2+y21+z21=1 (ii)
Now OC⊥CD
⇒ (x1−45)45+(y1−25)25+(z1−0)0=0 (iii)
From (i) and (ii), we get
−4y1+2x1=0orx1=2y1
From (iii), putting x1=2y1⇒2y1=45ory1=25⇒x1=(4)(5). Putting this value of x1andy1 in (i), we get
z1=±2√5
Topper's Solved these Questions
- THREE-DIMENSIONAL GEOMETRY BOOK - CENGAGE PUBLICATIONCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - MULTIPLE CORRECT ANSWER TYPE
17 Videos
- THREE-DIMENSIONAL GEOMETRY BOOK - CENGAGE PUBLICATIONCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - REASONING TYPE
10 Videos
- THREE-DIMENSIONAL GEOMETRY BOOK - CENGAGE PUBLICATIONCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - SUBJECTIVE TYPE
17 Videos
- THREE DIMENSIONAL GEOMETRYBOOK - CENGAGE PUBLICATIONCHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - All Questions
291 Videos
- TRIGONOMETRIC EQUATIONSBOOK - CENGAGE PUBLICATIONCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Numerical value type)
4 Videos
Similar Questions
Let PM be the perpendicular from the point P(1,2,3) to the x−y plane. If →OP makes an angle θ with the positive direction of the z− axis and→OM makes an angle φ with the positive direction of x− axis, whereO is the origin and θandφ are acute angels, then a. cosθcosφ=1/√14 b. sinθsinφ=2/√14 c. tanφ=2 d. tanθ=√5/3
View SolutionStatement 1: Lines →r=ˆi+ˆj−ˆk+λ(3ˆi−ˆj)and→r=4ˆi−ˆk+μ(2ˆi+3ˆk) intersect. Statement 2: →b×→d=0 , then lines →r=→a+λ→band→r=→c+λ→d do not intersect.
View SolutionIf the direction cosines of a variable line in two adjacent points be l,m,nandl+δl,m+δm,n+δn the small angle δθas between the two positions is given by
View SolutionFind the angle between the line whose direction cosines are given by l+m+n=0andl2+m2−n2=0.
View SolutionThe number of all possible values of θ, where 0<θ<π, for which the system of equations (y+z)cos3θ=(xyz)sin3θ,xsin3θ=2cos3θy+2sin3θzand(xyz)sin3θ=(y+2z)cos3θ+ysin3θ have a solution (x0,y0,z0) wiith y0z0≠0 is
View SolutionConsider triangle AOB in the x−y plane, where A≡(1,0,0),B≡(0,2,0)andO≡(0,0,0). The new position of O, when triangle is rotated about side AB by 900 can be a. (45,35,2√5) b. (−35,√25,2√5) c. (45,25,2√5) d. (45,25,1√5)
View SolutionLet a, b, c, d be real numbers between −5 and 5 such that |a|=√4−√5−a,|b|=√4+√5−b,
|c|=√4−√5+c,|d|=√4+√5+d,.
Then the product abcd is
View SolutionFind the area of the triangle whose vertices are: (-5,-1), (3,-5), (5,2)
View SolutionConsider triangle AOB in the x−y plane, where A≡(1,0,0),B≡(0,2,0)andO≡(0,0,0). The new position of O, when triangle is rotated about side AB by 900 can be a. (45,35,2√5) b. (−35,√25,2√5) c. (45,25,2√5) d. (45,25,1√5)
View SolutionConsider triangle AOB in the x−y plane, where A≡(1,0,0),B≡(0,2,0)andO≡(0,0,0). The new position of O, when triangle is rotated about side AB by 900 can be a. (45,35,2√5) b. (−35,√25,2√5) c. (45,25,2√5) d. (45,25,1√5)
View SolutionIn ABC , the coordinates of the vertex A are (4,−1) , and lines x−y−1=0 and 2x−y=3 are the internal bisectors of angles BandC . Then, the radius of the encircle of triangle ABC is (a) 4√5 (b) 3√5 (c) 6√5 (d) 7√5
View Solutionਜੇਕਰ A = [[2/3,1,5/3],[1/3,2/3,4/3],[7/3,2,2/3]] ਅਤੇ B = [[2/5,3/5,1],[1/5,2/5,4/5],[7/5,6/5,2/5]], ਤਾਂ 3A−5B ਪਤਾ ਕਰੋ।
View SolutionIf A=⎡⎢ ⎢ ⎢ ⎢⎣2315313234373223⎤⎥ ⎥ ⎥ ⎥⎦andB=⎡⎢ ⎢ ⎢ ⎢⎣25351152545756525⎤⎥ ⎥ ⎥ ⎥⎦, then compute 3A−5B
View SolutionIf the vertices of a triangle are (√5,0) , (√3,√2) , and (2,1) , then the orthocentre of the triangle is (√5,0) (b) (0,0) (c)(√5+√3+2,√2+1) (d) none of these
View SolutionConsider triangle AOB in the x−y plane, where A≡(1,0,0),B≡(0,2,0)andO≡(0,0,0). The new position of O, when triangle is rotated about side AB by 900 can be a. (45,35,2√5) b. (−35,√25,2√5) c. (45,25,2√5) d. (45,25,1√5)
View SolutionConsider triangle A O B in the x-y plane, where A-=(1,0,0),B-=(0,2,0)a n d O-=(0,0,0)dot The new position of O , when triangle is rotated about side A B by 90^0 can be
View Solution
