In the following figure (not to scale ), two chords XY and PQ are intersecting at the point A . The line segment joining X and P is a diameter of the circle , ∠XAP=120∘andXU=PQ=18cm . Find the distance between the centre of the circle and the point A .
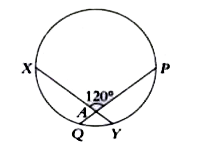
3 cm
4 cm
8 cm
6 cm
The correct Answer is:D
Step by step video, text & image solution for In the following figure (not to scale ), two chords XY and PQ are intersecting at the point A . The line segment joining X and P is a diameter of the circle , angleXAP=120^(@)andXU=PQ=18cm . Find the distance between the centre of the circle and the point A . by Maths experts to help you in doubts & scoring excellent marks in Class 8 exams.
Updated on:21/07/2023Topper's Solved these Questions
- GEOMETRY BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GEOMETRY EXERCISE - TEST YOUR CONCEPTS (ESSAY TYPE QUESTIONS)
5 Videos
- FormulaeBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - FormulaeEXERCISE - Level 3
7 Videos
- INDICESBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - INDICESEXERCISE - CONCEPT APPLICATION (Level 3)
7 Videos
Similar Questions
The length of common chord of two circles is 30 cm. if the diameters of circles are 50 cm and 34 cm, then find the distance between these centres.
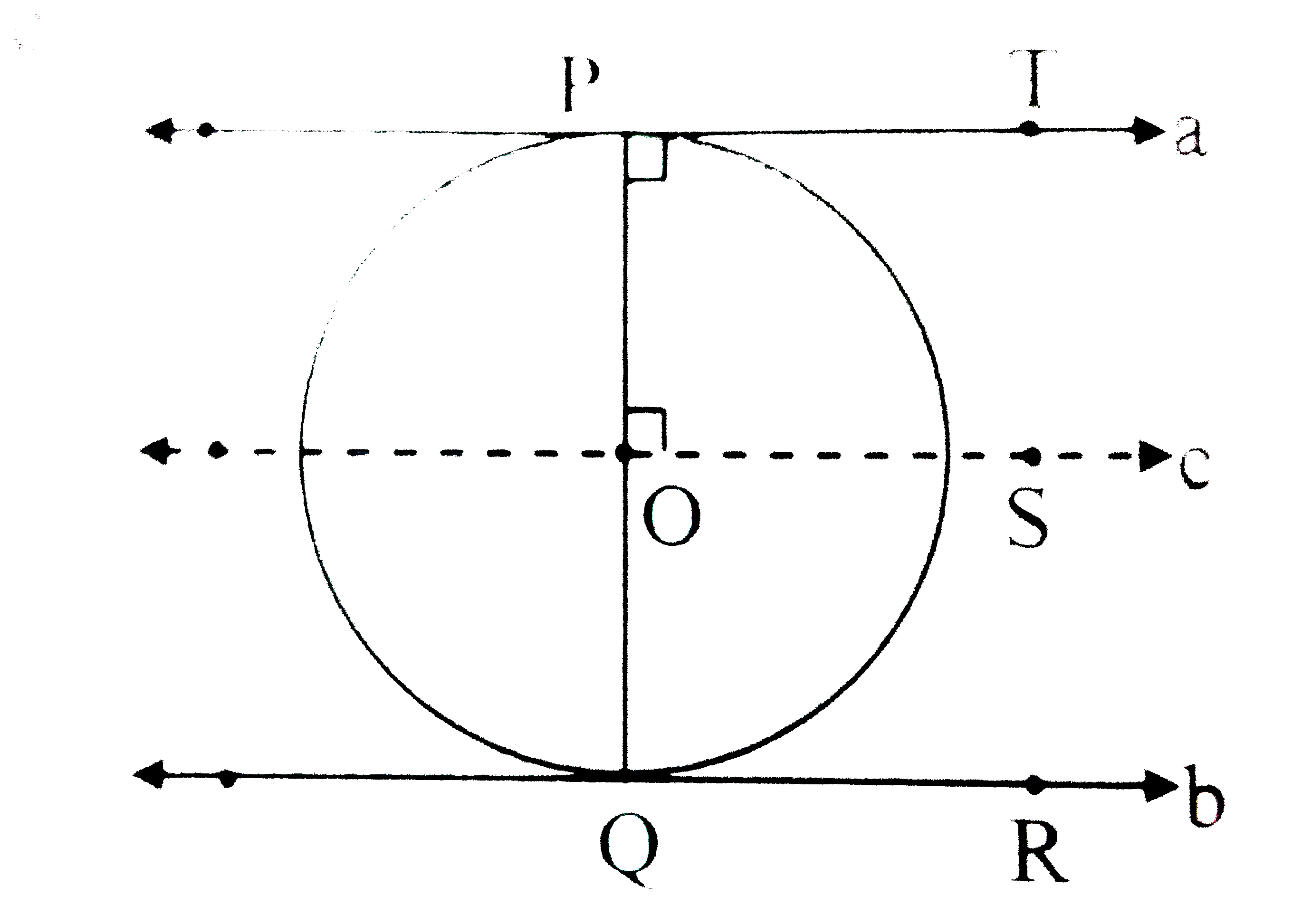
View SolutionPoint O is the centre of a circle . Line a and line b are parallel tangents to the circle at P and Q. Prove that segment PQ is a diameter of the circle.
View Solution
Knowledge Check
PQ is tangent at a point R of the circle with centre O. If ST is a diameter of the circle and ∠TRQ=30∘ find ∠PRS
A30∘B60∘C45∘D120∘The length of the common chord of two intersecting circles is 24 cm. If the diameter of the circles are 30 cm and 26 cm, then the distance between the centre (in cm) is
A13B14C15D16The line segment joining the points (4,7) and (-2,-1) is a diameter of a circle. If the circle intersects the X-axis at A and B, then AB is equal
A4B5C6D8
In the following figure,PQ is the diameter of the circle with radius 5 cm. if AT is the tangent and equal to the radius of the circle, then find the lenth of AR.
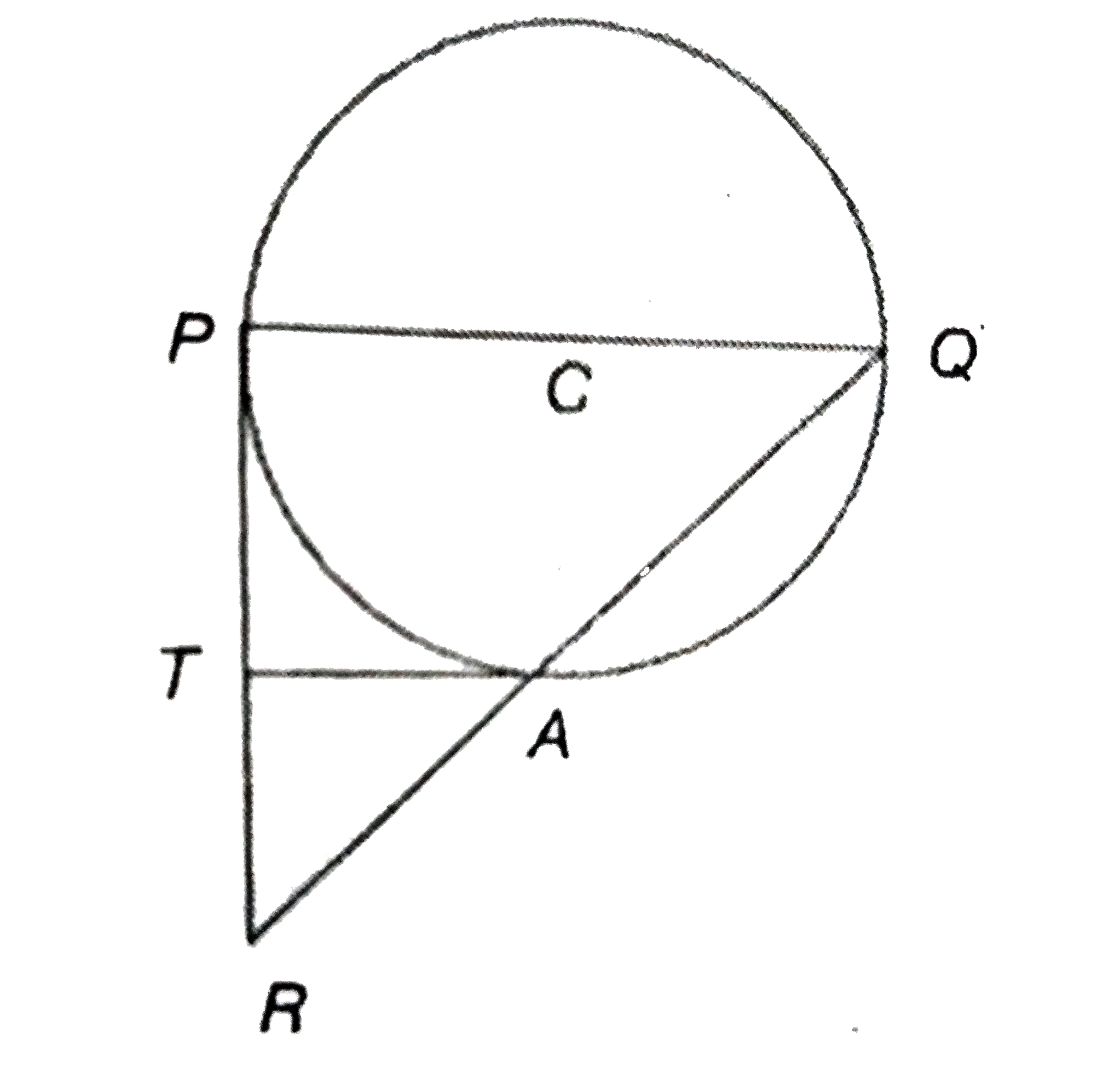
View SolutionIn the given figure, a diameter PQ of a circle bisects the chord RS at the point O. If PS is parallel to RQ, prove that RS is also a diameter of the circle.
View SolutionIf the two equal chords of a circle intersect :
(i) inside
(ii) on
(iii) outside
the circle, then show that the line segment joining the point of intersection to the centre of the circle will bisect the angle between the chords.
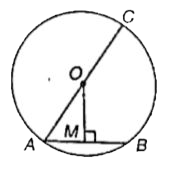
View SolutionIn the adjoining figure, O is the centre of circle and diameter AC=26cm. If chord AB=10cm, then the distance between chord AB and centre O of the circle is :
View SolutionTangents to a circle at points P and Q on the circle intersect at a point R. If PQ= 6 and PR= 5 then the radius of the circle is
View Solution
