A test tube of uniform cross-section is floated vertically in a liquid 'A' (density ρA) upto a mark on it when it is filled with 'x' ml of a liquid 'B' (density ρB). To make the test tube float in liquid B upto the same mark it is filled with y ml of the liquid A. Find the mass of the test tube.
What is law of floatation? Is mass of the test tube (float) changed, when it is immersed in different liquids? Then. can we say that it is a constant immersion hydrometer? Assume the mass of the test tube = M. When immersed in liquid .A.. the float is filled with .x. ml of liquid B.Then find the weight of the float. Is the volume of liquid .B. in the float = x ml. Then, weight of the liquid = vdg
Now, the weight of the float = mg+xpBg
Similarly, fmd the weight of the float when immersed in liquid .B..
ls the weight of the float = mg+yPAg
But, weight of the float = weight of the displaced liquid. The volume of the displaced liquids (both A and B) are equal Then get the volume of displ.iced liquids from above two cases in fonn of equations and equate them. From this, obtain the mass of the test tube.
(ii) 51680 Pa
Topper's Solved these Questions
- HYDROSTATICSBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - HYDROSTATICSEXERCISE - CONCEPT APPLICATION (LEVEL 2)
20 Videos
- HEATBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - HEATEXERCISE - CONCEPT APPLICATION Level 3
10 Videos
- KINEMATICS BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - KINEMATICS EXERCISE - CONCEPT APPLICATION (Level 3) (Application Based Questions )
5 Videos
Similar Questions
A test tube of uniform cross-section is floated vertically in a liquid 'A' (density ρA) upto a mark on it when it is filled with 'x' ml of a liquid 'B' (density ρB). To make the test tube float in liquid B upto the same mark it is filled with y ml of the liquid A. Find the mass of the test tube.
View SolutionA test tube floats to a depth 4 cm in water and to a depth 8 cm in a liquid. The relative density of the liquid is _____.
View Solution
Knowledge Check
A body of uniform cross-sectional area floats in a liquid of density thrice its value. The portion of exposed height will be :
A2/3B5/6C1/6D1/3A test tube of length l and area of cross-section A has some iron fillings of mass M. The test tube floats normally in a liquid of density ρ with length x dipped in the liquid. A distribuing force makes the tube oscillate in the liquid. The time period of osciallation is given by (neglect the mass of the test tube)
A(2π√MρAgB2π√xgC2π√lgD2π√MgA beaker is filled with a liquid of density ρ upto a height h If the beaker is at rest, the mean pressure at the walls is:
A0BhρgChρg3D2hρg
A circular tube of uniform cross section is filled with two liquids densities ρ1 and ρ2 such that half of each liquid occupies a quarter to volume of the tube. If the line joining the free surfaces of the liquid makes an angle θ with horizontal find the value of θ.
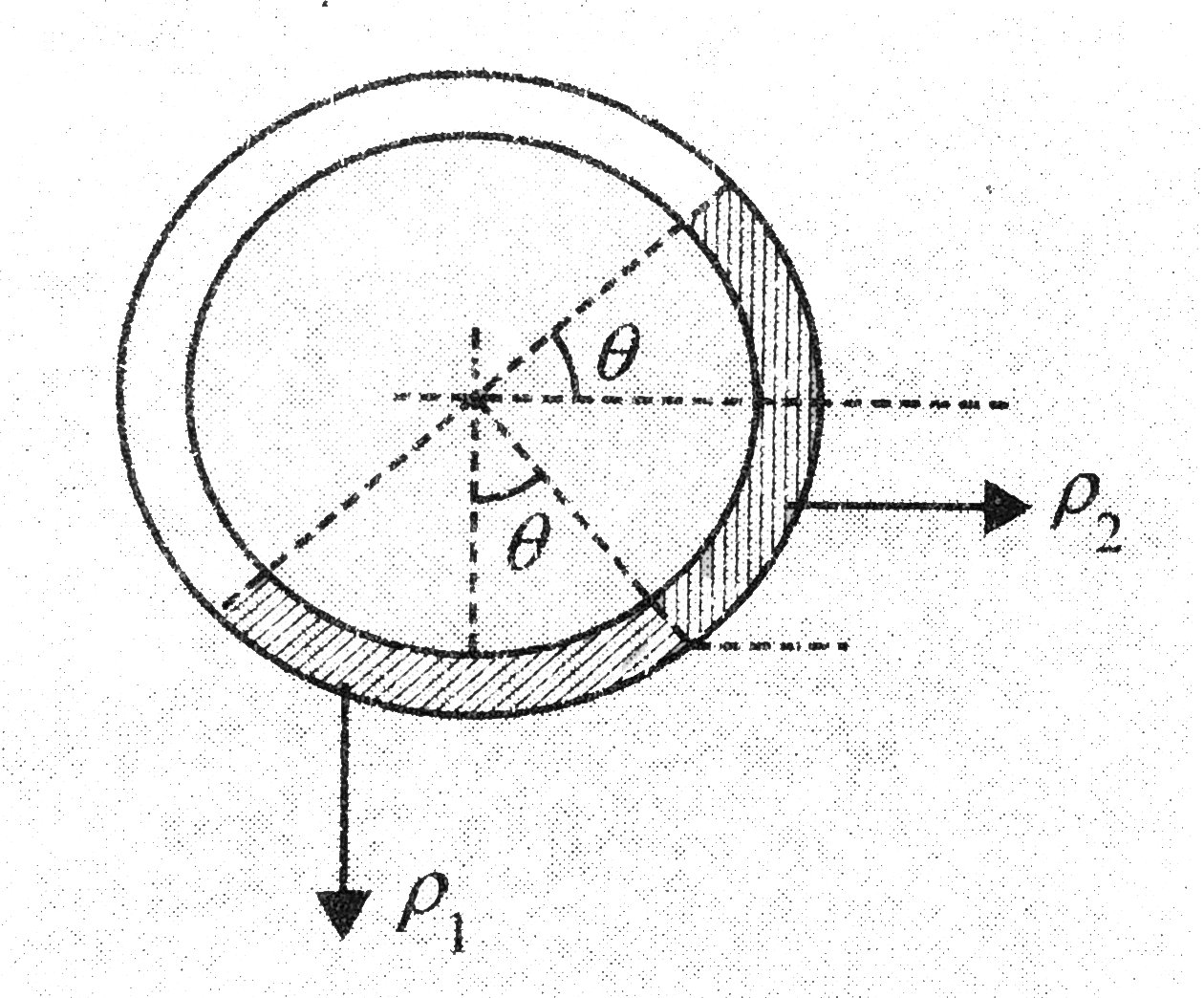
View SolutionWhen a capillary tube of radius r is immersed in a liquid of density ρ, the liquid rises to a height h in it. If m is the mass of the liquid in the capillary tube, find the potential energy of this mass of the liquid in the tube.
View SolutionA V-shaped glass tube of uniform cross section is kept in a vertical plane as shown. A liquid is poured in the tube. In equilibrium the level of liquid in both limbs of tube are equal. Find the angular frequency of small oscillation of liquid.

View SolutionA uniform cube of mass M is floating on the surface of a liquid with three fourth of its volume immersed in the liquid (density=ρ). The length of the side of the cube is equal to

View SolutionWhen a loaded test tube floats vertically with 13 and 14 of the lengths inside two liquids, then the ratio of the densities of the two liquids is
View Solution
