ABC is triangle. D is the middle point of BC. If AD is perendicular to AC, then prove that
cosAcosC=2(c2−a2)3acSolution in Tamil
Video Solution
Text Solution
In ΔADC, we have
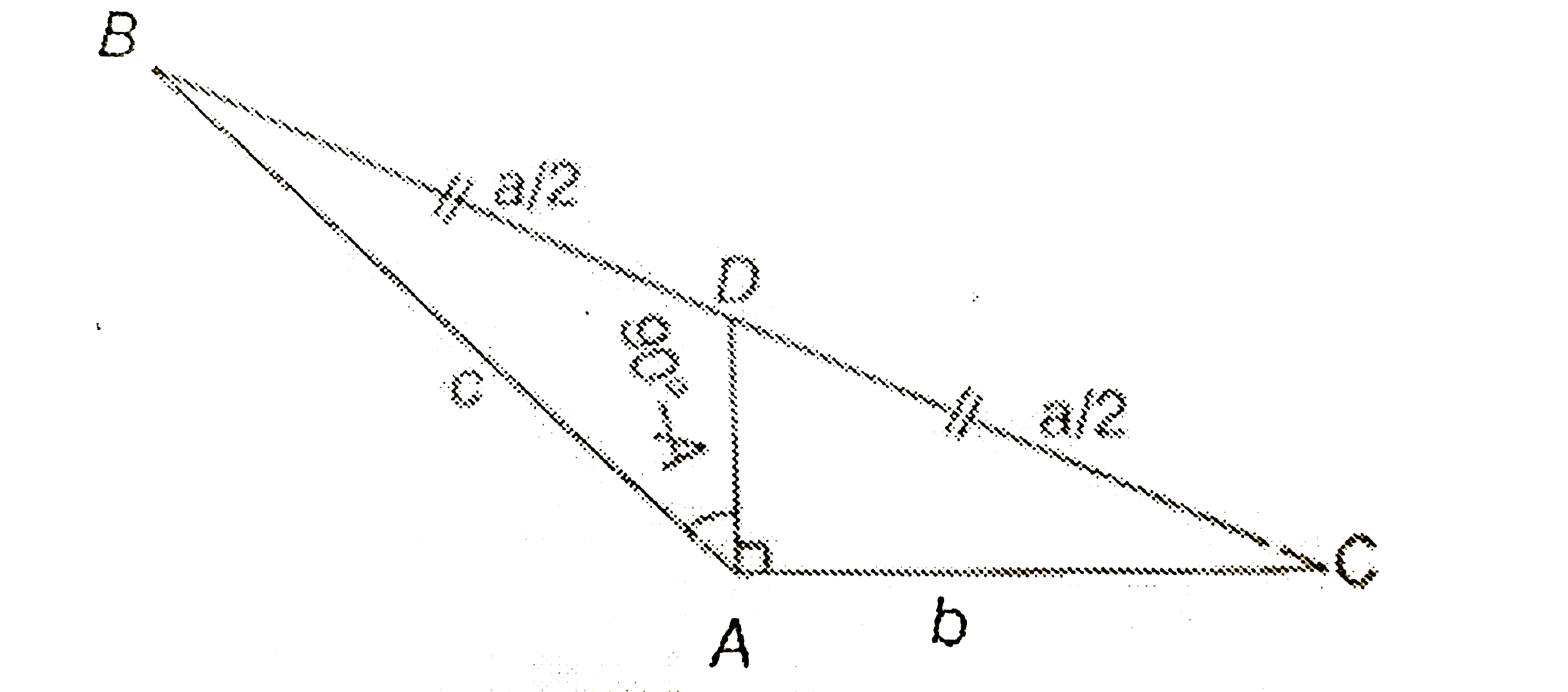
cosC=ACCD
cosC=2ba ...(i)
Applying cosine formula in ΔABC, we have
cosA=b2+c2−a22bc
and cosC=a2+b2−c22ab ....(ii)
From Eqs.(i) and (ii),
a2+b2−c22ab=2ba
⇒a2+b2−c2=4b2
⇒a2−c2=3b2 ....(iii)
Now, cosAcosC=b2+c2−a22bc⋅2ba
b2+c2−a2bc=3b2+3(c2−a2)3ac
(a2+c2)+3(c2−a2)3ac=2(c2−a2)3ac
|
Similar Questions
ABC একটি ত্রিভুজ । BC বাহুর মধ্যবিন্দু D । যদি AC -এর ওপর AD লম্ব হয়, তবে প্রমাণ করো যে cosAcosC=2(c2−a2)3ca।
View SolutionIn Fig. 3, ABC is a right triangle, right angled at C and D is the mid-point of BC. Prove that AB2=4AD2−3AC2.
View SolutionIn ΔABC , D is the mid point of BC and AD is perpendicular to AC , then show that
cosAcosC=(2c2−a23ac
View SolutionD is midpoint of BC in ΔABC such that AD and AC are perpendicular, Show that CosACosC=2(c2−a2)3ac
View SolutionIf D is the middle point of the side BC of the triangle ABC, prove that AB2+AC2=2(AD2+DC2).
View SolutionIn an isosceles triangle ABC, AB = AC and D is a point on BC produced. Prove that :
AD2=AC2+BD.CD
View SolutionABC is a triangle in which AB = AC and D is any point on BC. Prove that:
AB2−AD2=BD.CD
View Solution