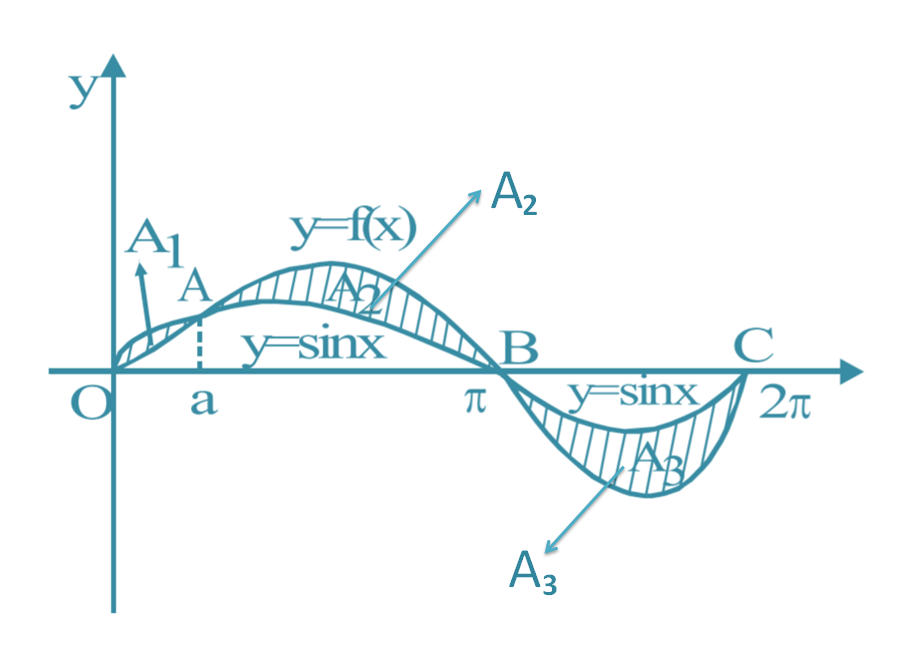
In the following figure, the graphs of two functions y = f(x) and y = sin x are givne. They intersect at origin, A(a,f(a)),B(π,0)andC(2π,0).Ai(i=1,2,3) is the area bounded by the curves as shown in the figure, respectively, for x∈(0,a),x∈(a,π),x∈(π,2π).
If A1=1+(a−1)cosa−sin a, then ,the value of A 2 ?Solution in Tamil
a) (π−1)units2
b) (π/2−1)units2
c) (π−sin1−1)units2
d)π/2units2
The correct Answer is:C
A1=∫a0(sinx−f(x))dx=1+(a−1)cosa−sina
Differentiating w.r.t. 'a' both sides.
∴ sina−f(a)=cosa−(a−1)sina−cosa
∴ f(a)=asina
∴ f(x)=xsinx
Solving y = sin x wity y = x sin x, we have x sin x = sin x
∴ x=1orx=π,2π
∴ A2=∫π1(xsinx−sinx)dx
=(−xcosx)π1−∫π1−cosxdx+(cosx)π1
=(π+cos1)+(0−sin1)+(−1−cos1)
=(π−sin1−1)sq. units
Topper's Solved these Questions
Similar Questions
If the area of bounded between the x-axis and the graph of y=6x−3x2 between the ordinates x=1andx=a is 19 units, then a can take the value 4or−2 two value are in (2,3) and one in (−1,0) two value are in (3,4) and one in (−2,−1) none of these
View SolutionProve that for λ>1, the equation xlogx+x=λ has least one solution in [1,λ].
View SolutionThe area of the region (s) enclosed by the curves y=x2 and y=√|x| is
View SolutionA function y=f(x) satisfies the condition f'(x)sin x+f(x)cos x=1,f(x) being bounded when x rarr0. If I=int_(0)^((pi)/(2))f(x)dx then (A)(pi)/(2)
View SolutionA function y=f(x) satisfies f(x)=-(1)/(x^(2))-pi^(2)sin(pi x);f'(2)=pi+(1)/(2) and f(1)=0. The value of f((1)/(2))
View SolutionA function y=f(x) satisfies f''(x)=−1x2−π2sin(πx), f'(2)=π+12 and f(1)=0then value of f(12) is
View SolutionThe area bounded by the curves y=sin−1|sinx|andy=(sin−1|sinx|)2, where 0≤x≤2π, is
View SolutionArea bounded by y=f−1(x) and tangent and normal drawn to it at points with abscissae π and 2π, where f(x)=sinx−x is
View SolutionIn the adjacent figure the graph of two function y=f(x) and y=sinx are given y=sin x intersects, y=f(x) at A(a,f(a)),B(π,0)andC(2π,0).
Ai(i=1,2,3) is the area bounded by the curves y=f(x)andy=sinx between x=0 and x=a,i=1 between x=a and x=π,i=2 between x=π and x=2π,i=3. If A1=1−sina+(a−1) cos a, determine the function f(x). Hence, determine a and A1. Also, calculate A2andA3.
 .
.
View SolutionIf A_(1) is the area bounded by the curve y = cos x and A_(2) is the area bounded by y = cos 2 x along with x - axis from x = 0 and x = (pi)/(0) , then
View Solutionsin−1x+cos−1x,x∈[−1,1]=
(A)0(B)π2(C)π(D)2π
View SolutionThe area bounded by the curves y=sin−1|sinx|andy=(sin−1|sinx|)2, where 0≤x≤2π, is
View SolutionIn the following figure, the graphs of two functions y = f(x) and y = sin x are givne. They intersect at origin, A(a,f(a)),B(π,0)andC(2π,0).Ai(i=1,2,3) is the area bounded by the curves as shown in the figure, respectively, for x∈(0,a),x∈(a,π),x∈(π,2π).
If A1=1+(a−1)cosa−sin a, then

The function f(x) is
View SolutionIf x∈[0.π2],y∈[0,π2]andsinx+cosy=2, then the value of x+y is equal to........ A) 2π B) π C) π4 D) π2
View Solutionएक फलन y=f(x),f''(x)=−1x2−π2sin(πx),f'(2)=π+12 को संतुष्ट करता है तथा f(1)=0 है तो f(12) का मान है।
View Solutionदिए गये चित्र में दो फलन y=f(x) तथा y=sinx एक दुसरे को बिन्दु A(a,f(a)),B(π,0) तथा C(2π,0) पर काटते है | Ai(i=1,2,3) वक्र y=f(x) तथा y=sinx का x=0 तथा x=a के मध्य क्षेत्रफल, (i=1),x=a तथा x=π के मध्य, (i=2),x=π तथा x=2π के मध्य, (i=3) है| यदि Ai=1−sina+(a−1)cosa तो फलन f(x) ज्ञात कीजिए 'a',A1,A2 तथा A3 के मान ज्ञात कीजिए |
View Solution