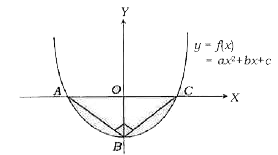
In the given figure, vertices of ΔABC lie on y=f(x)=ax2+bx+c. The ΔABC is right angled isosceles triangle whose hypotenuse AC=4√2 units. Number of integral values of k for which one root of f(x)=0 is more than k and other less than kSolution in Tamil
6
4
5
7
The correct Answer is:3
f(x)=0
⇒x2/(2√2)−2√2=0 or x=±2√2
Therefore, number of integral values of K for which for which k lies in (−2√2,2√2) is 5.
Topper's Solved these Questions
- THEORY OF EQUATIONSBOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - Exercise (Matrix)
6 Videos
- THEORY OF EQUATIONSBOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - Exercise (Numerical)
43 Videos
- THEORY OF EQUATIONSBOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - Exercise (Multiple)
38 Videos
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - JEE Advanced Previous Year
4 Videos
- THREE DIMENSIONAL GEOMETRYBOOK - CENGAGECHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - Question Bank
12 Videos
Similar Questions
Match the following for the equation x2+a|x|+1=0 where, a is a parameter.

View SolutionIf x=2+223+213, then the value of x3−6x2+6x is:
View Solutionif c≠0 and the equation p2x=ax+c+bx−c has two equal roots, then p can be
View Solutionabout to only mathematics
View SolutionThe equation of the plane which has the property that the point Q(5,4,5) is the reflection of point P(1,2,3) through that plane, is ax+by+cz=d where a,b,c,d∈N. Find the least value of (a+b+c+d).
View SolutionIn the given figure,vertices of Delta ABC lie on y=f(x)=ax^(2)+bx+c. The Delta ABC is right angled isosceles triangle whose hypotenuse AC=4sqrt(2) units.Number of integral values of k sqrt(2) which one root of f(x)=0 is more than k and other less than k
View SolutionIn the given figue vertices of ΔABC lie on y=f(x)=ax2+bx+c. The ΔAB is right angled isosceles triangle whose hypotenuse AC=4√2 units.
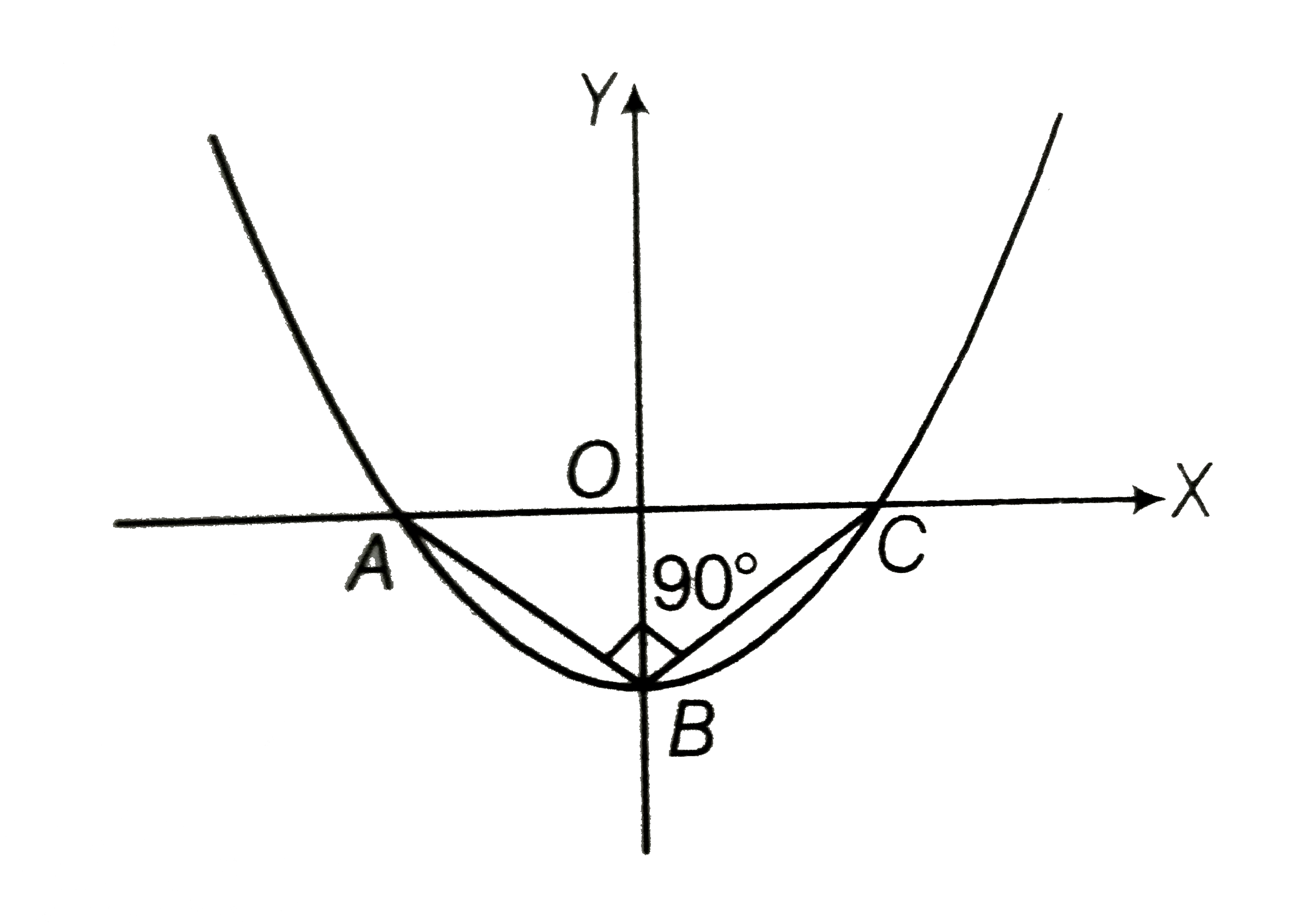
y=f(x) is given by
View SolutionIn the given figue vertices of ΔABC lie on y=f(x)=ax2+bx+c. The ΔAB is right angled isosceles triangle whose hypotenuse AC=4√2 units.
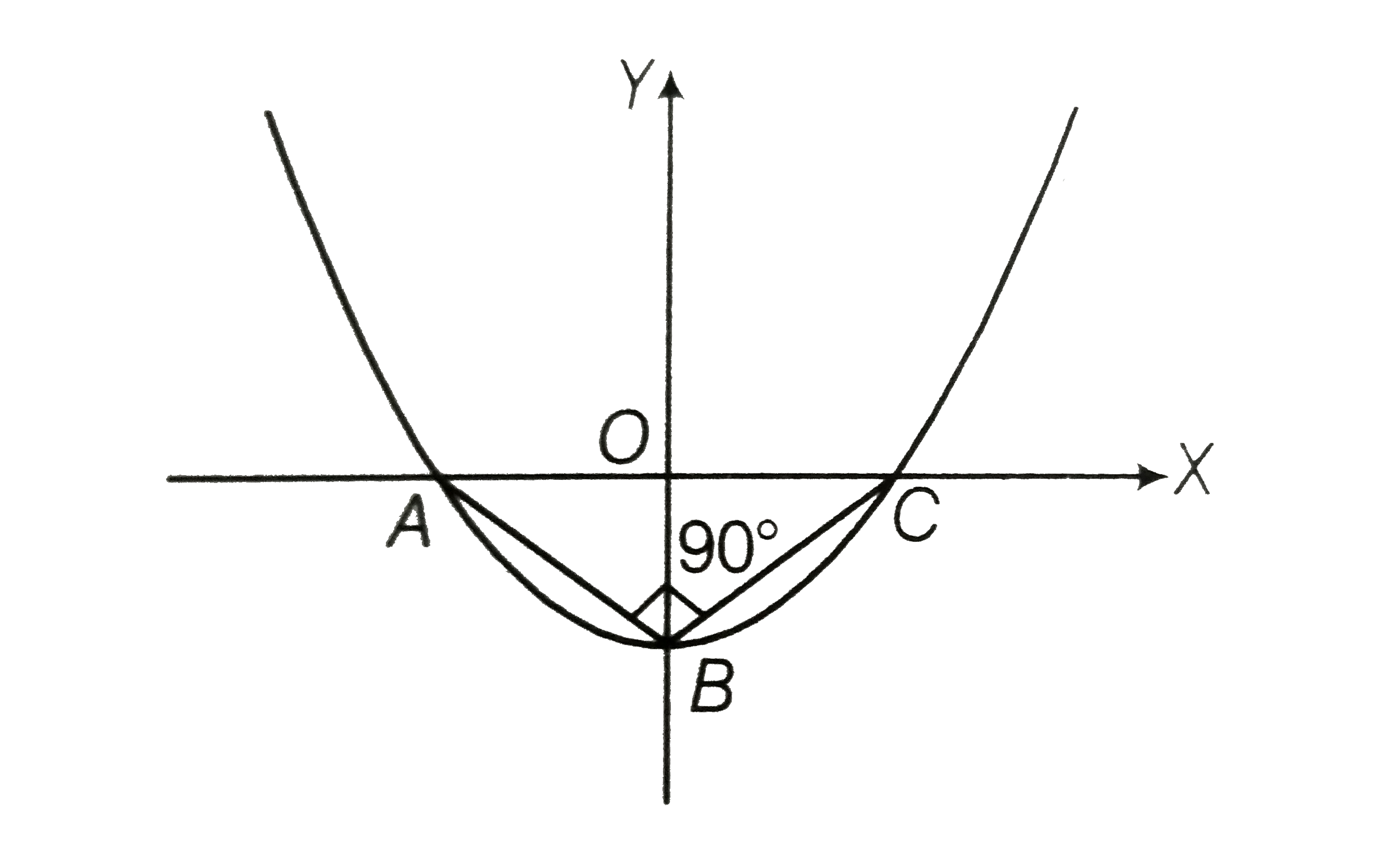
Minimum valueof y=f(x) is
View SolutionIn the given figue vertices of ΔABC lie on y=f(x)=ax2+bx+c. The ΔAB is right angled isosceles triangle whose hypotenuse AC=4√2 units.
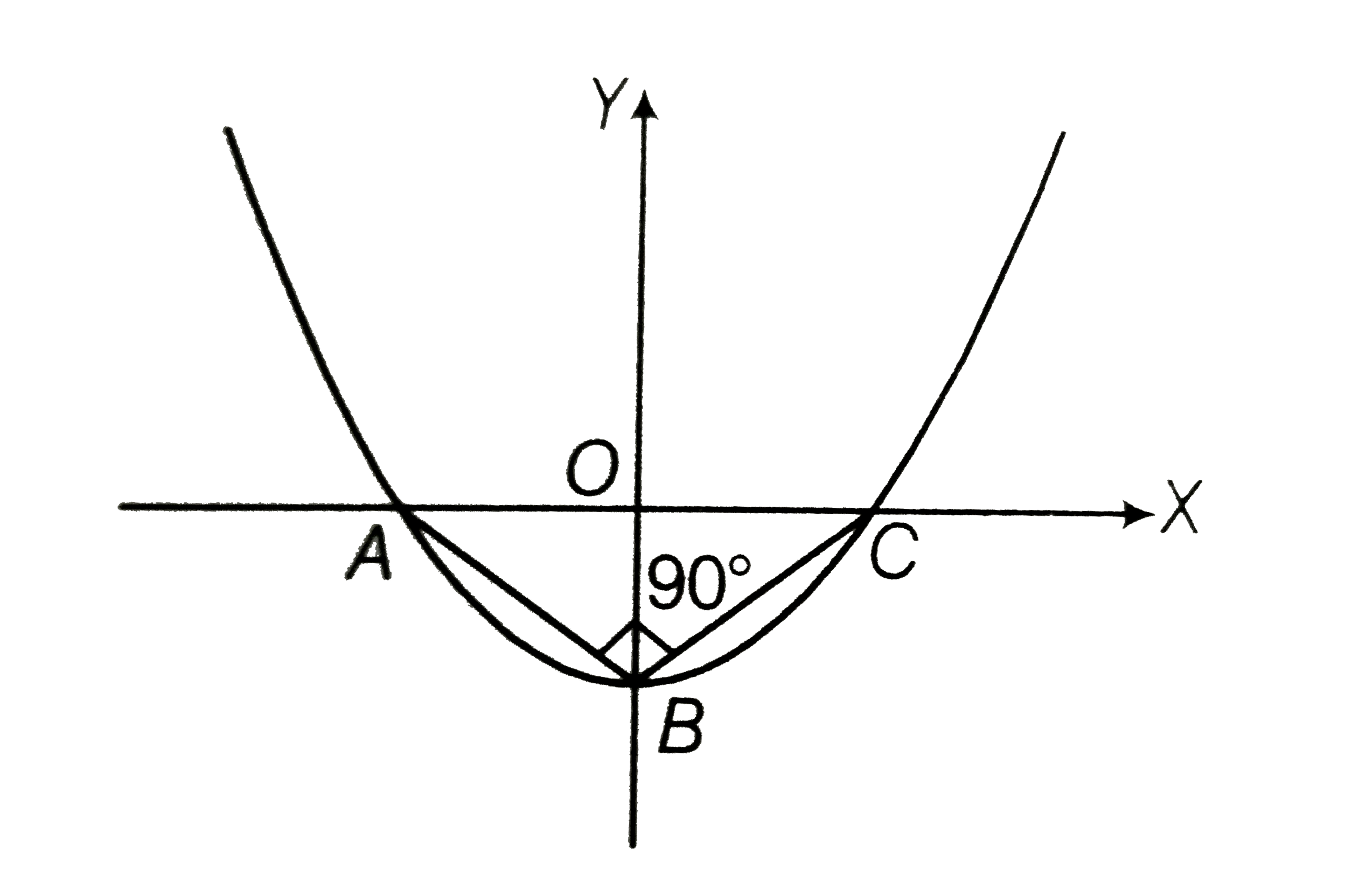
Number of integral value of λ for which λ2 lies between the roots of f(x)=0, is
View Solutionदिये गये चित्र में ΔABC के शीर्ष y=f(x)=ax2+bx+c पर स्थित है ΔABC समकोण समद्विबाहु त्रिभुज है जिसका कर्ण AC=4√2 इकाई है, तब

y=f(x) दिया गया है
View Solutionदिये गये चित्र में ΔABC के शीर्ष y=f(x)=ax2+bx+c पर स्थित है ΔABC समकोण समद्विबाहु त्रिभुज है जिसका कर्ण AC=4√2 इकाई है, तब
समीकरण y=f(x) का न्यूनतम मान है
View Solutionदिये गये चित्र में ΔABC के शीर्ष y=f(x)=ax2+bx+c पर स्थित है ΔABC समकोण समद्विबाहु त्रिभुज है जिसका कर्ण AC=4√2 इकाई है, तब
k के पूर्णांक मानों की संख्या जिसके लिए k2,f(x)=0 के मूलों के बीच स्थित है
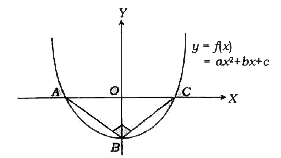
View SolutionIn the given figue vertices of ΔABC lie on y=f(x)=ax2+bx+c. The ΔABC is right angled isosceles triangle whose hypotenuse AC=4√2 units.

y=f(x) is given by
View SolutionIn the given figue vertices of ΔABC lie on y=f(x)=ax2+bx+c. The ΔABC is right angled isosceles triangle whose hypotenuse AC=4√2 units.

Minimum valueof y=f(x) is
View SolutionIn the given figue vertices of ΔABC lie on y=f(x)=ax2+bx+c. The ΔAB is right angled isosceles triangle whose hypotenuse AC=4√2 units.

Number of integral value of λ for which λ2 lies between the roots of f(x)=0, is
View Solution
