Solve x2−4−[x]=0 (where [] denotes the greatest integer function).Solution in Tamil
The best method to solve such an equation is graphical one.
Give equation is x2−4=[x].
Then the solutions to the question are values of x where the graphs of y=x2−4andy=[x] interesect.
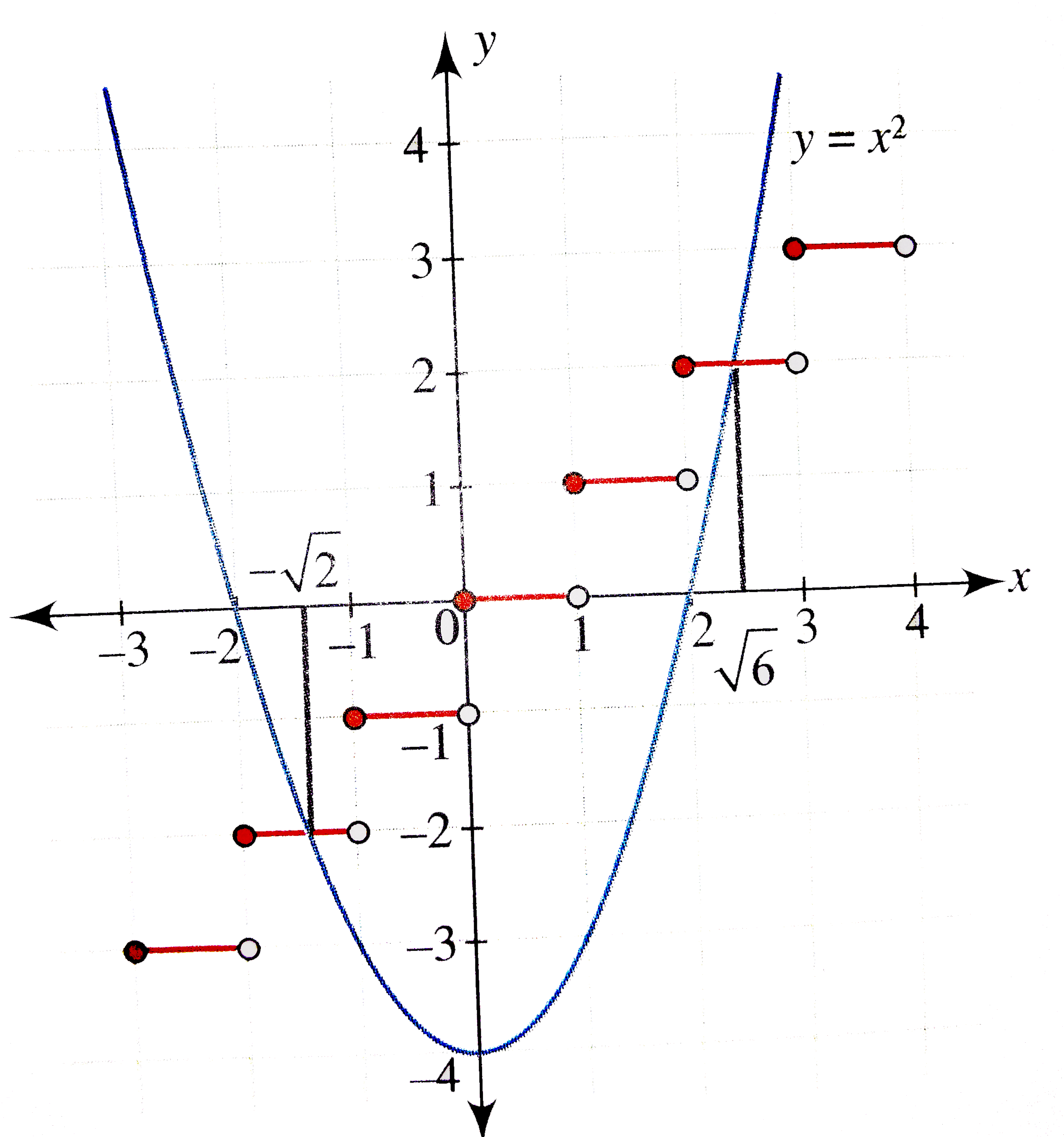
From the figure, it is seen that the graphs intersect when
x2−4=2andx2−4=2.
⇒ x2=6orx2k=2
⇒ x=x=√6or−√2
Topper's Solved these Questions
- GRAPHS OF ELEMENTARY FUNCTIONS BOOK - CENGAGECHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - Exercise
34 Videos
- GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercises
4 Videos
- GRAPHS OF TRIGONOMETRIC FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise
22 Videos
Similar Questions
Draw the graph of y=1(1/x).
View SolutionSketch the graph of an example of a rational function f that satisfies all the given conditions.
(i) f(0)=0,f(1),limx→∞f(x)=0,f is odd
(ii) limx→0+f(x)=∞,limx→0−f(x)=−∞,limx→∞f(x)=1,limx→−∞f(x)=1
(iii) limx→−2f(x)=∞,limx→−∞f(x)=3,limx→∞f(x)=3,f(0)=0
View SolutionDraw the graph of y= cosec 2x−cot2x. Is f(x) periodic ? If yes, what is its fundamental period ?
View SolutionSolve x2−4−[x]=0 (where [] denotes the greatest integer function).
View SolutionIf [log_(2)((x)/([x]))]>=0, where [.] denote the greatest integer function,then
View SolutionSolve : 4{x}=x+[x](where [⋅] denotes the greatest integer function and {⋅} denotes the fractional part function.
View SolutionSolve x^2-4-[x]=0 (where [] denotes the greatest integer function).
View SolutionSolve x^2-4x-[x]=0 (where [] denotes the greatest integer function).
View SolutionSolve : 4{x}=x+[x](where [⋅] denotes the greatest integer function and {⋅} denotes the fractional part function.
View SolutionSolve x^2-4-[x]=0 (where [] denotes the greatest integer function).
View SolutionSolve
limx→0+[sinxx]
(where [.] denotes greatest integer function.)
View SolutionSolve
limx→0−[sinxx]
(where [.] denotes greatest integer function.)
View SolutionSolve
limx→0+[tanxx]
(where [.] denotes greatest integer function.)
View SolutionSolve
limx→0−[tanxx]
(where [.] denotes greatest integer function.)
View SolutionSolve x^2-4-[x]=0 (where [] denotes the greatest integer function).
View SolutionSolve : 4{x}= x+ [x] (where [*] denotes the greatest integer function and {*} denotes the fractional part function.
View Solution
