A tower AB leans towards west making an angle α with the vertical . The anlgular elevation of B , the topmost point of the tower is β as obsreved from a point C due east of A at distance d from A.If the angular elevation of B from a pont D at a distance 2d due east of C is γ , then prove that 2 tan α = cot γ -cot βSolution in Tamil
2tanα=2cotβ−cotγ
2tanα=3cotβ−cotγ
tanα=cotβ−cotγ
None of these
Observe the following figure
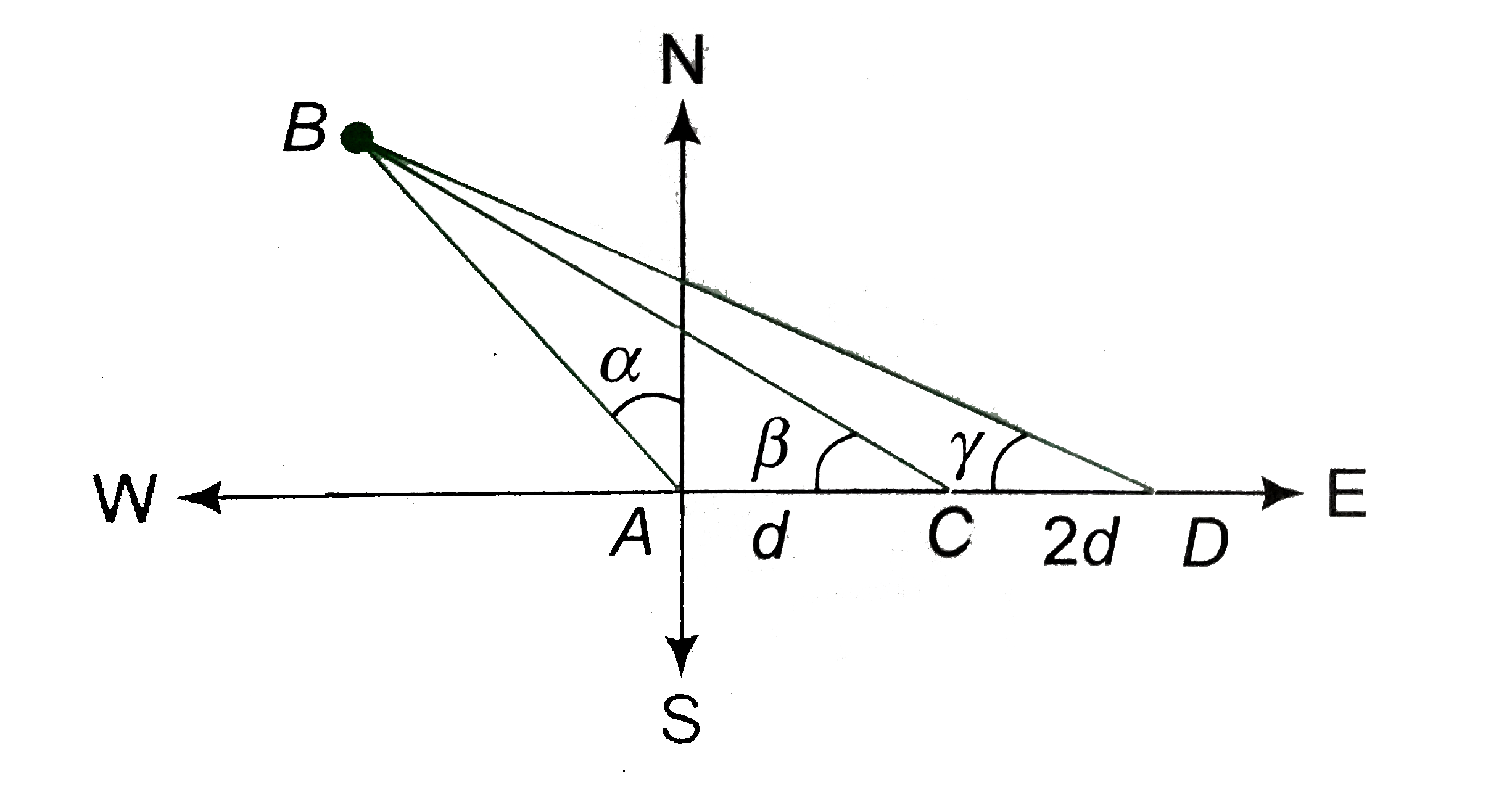
Using m-n theorem in triangle ABD we have
(d+2d)cotBη=dcotα−2dcot(90+α)
⇒3dcotβ=cotα+2dtanα
∴2tanα=3cotβ−cotα
Topper's Solved these Questions
- HIGHT AND DISTANCE BOOK - CENGAGECHAPTER - HIGHT AND DISTANCE EXERCISE - JEE Previous Year
3 Videos
- HIGHT AND DISTANCE BOOK - CENGAGECHAPTER - HIGHT AND DISTANCE EXERCISE - JEE Previous Year
3 Videos
- GRAPHS OF TRIGONOMETRIC FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise
22 Videos
- HYPERBOLABOOK - CENGAGECHAPTER - HYPERBOLAEXERCISE - JEE Advanced Previous Year
14 Videos
Similar Questions
A bird is sitting on the top of a vertical pole 20 m high and its elevation from a point O on the ground is 45o . It flies off horizontally straight away from the point O. After one second, the elevation of the bird from O is reduced to 30o . Then the speed (in m/s) of the bird is (1) 40(√2−1) (2) 40(√3−2) (3) 20√2 (4) 20(√3−1)
View SolutionA bird is sitting on the top of a vertical pole 20 m high and its elevation from a point O on the ground is 45o . It flies off horizontally straight away from the point O. After one second, the elevation of the bird from O is reduced to 30o . Then the speed (in m/s) of the bird is (1) 40(√2−1) (2) 40(√3−2) (3) 20√2 (4) 20(√3−1)
View SolutionDraw the graph of y= cosec 2x−cot2x. Is f(x) periodic ? If yes, what is its fundamental period ?
View SolutionLet P(6,3) be a point on the hyperbola x2a2−y2b2=1. If the normal at point P intersects the x-axis at (9, 0), then find the eccentricity of the hyperbola.
View SolutionThe angle of elevation of the top of a tower from a point A due south of the tower is alpha and from B due east of tower is beta. If AB=d , show that the height of the tower is (d)/(sqrt(cot^(2)alpha+cot^(2)beta))
View SolutionA tower AB leans towards west making an angle α with the vertical . The anlgular elevation of B , the topmost point of the tower is β as obsreved from a point C due east of A at distance d from A.If the angular elevation of B from a pont D at a distance 2d due east of C is γ , then prove that 2 tan α = cot γ -cot β
View Solutionजमीन पर एक बिंदु P से एक मीनार के शिखर का उन्नयन कोण α है। मीनार के पाद की ओर d दूरी चलने के बाद उन्नयन कोण β हो जाता है तब-
View Solutionएक टॉवर के दक्षिण में स्थित एक बिंदु A पर टॉवर के शीर्ष से उन्नयन कोण α तथा पूर्व में स्थित बिंदु B से उन्नयन कोण β है| यदि AB=d, तब सिद्ध कीजिए की टॉवर की ऊंचाई d√cot2α+cot2β है|
View SolutionA tower AB leans towards west making an angle α with vertical. The angular elevation of B, the top most point of the tower, is 60∘ as observed from a point C due east of A at a elevation of 10 ft from A. If the angular elevation of B from a point D due east of C at a distance of 20 ft from C is 45∘, then the value of 2tanα is equal to
View SolutionA tower leans towards west making an angle α with the vertical. The angular elevation of B, the top most point of the tower, is 75∘ as observed from a point C due east of A at a distance of 20 units. If the angular elevation of B from a point due east of C at a distance of 20 units from C is 45∘, then tanα is equal to
View SolutionThe angle of elevation of the top of a tower at a point A due south of the tower is α and at β due east of the tower is β. If AB=d, then height of the tower is
View Solutionपश्चिम दिशा की ओर झुका हुआ एक स्तम्भ ऊर्ध्वाधर से α कोण बनाता है | बिन्दु 'A' के पश्चिम दिशा में A से 'd' दूरी पर स्थित एक बिन्दु 'C' से स्तम्भ के शीर्ष बिन्दु B का कोणीय उन्नतांश β है | यदि C के पूर्व में C से 2d दूरी पर स्थित एक बिन्दु D से, B का कोणीय उन्नतांश γ है, तो सिद्ध कीजिए कि 2tanα=cotγ−cotβ
View Solutionकिसी टॉवर के शिखर का टॉवर के दक्षिण में स्थित बिंदु A पर उन्नयन कोण αतथा टॉवर के पूर्व में स्थित बिंदु B पर उन्नयन कोण β है। यदि AB = d हो, तो टॉवर की ऊंचाई है
View SolutionA tower AB leans towards west making an angle α with the vertical . The anlgular elevation of B , the topmost point of the tower is β as obsreved from a point C due east of A at distance d from A.If the angular elevation of B from a pont D at a distance 2d due east of C is γ , then prove that 2 tan α = cot γ -cot β
View SolutionThe angle of elevation of the top of a tower from a pointA due south of the tower is α and from B due east of tower is β. If AB=d, show that the height of the tower is d√cot2α+cot2β.
View Solution
