Question

An electric dipole of small length lies along X-axis of a coordinates system. Derive an expression for the magnitude of electric field E due to the dipole at any point on its equatorial line. Mention its direction.

Explanation

Consider an electric dipole consisting of two point charges `+q and -q` separated by a small distance AB= 2l. Let P be a point on the equatorial line of a dipole at a distance r from the mid-point of dipole.

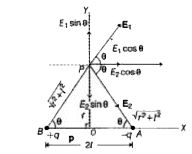
Resultant electric field intensity at point P is
`E_(P)= E_(1) + E_(2)`
The vectors `E_(1) and E_(2)` are acting at an angle `2theta`.
Here, `E_(1)= (1)/(4pi epsi_(0)).(q)/(r^(2)+ l^(2))`
and `E_(2)= (1)/(4pi epsi_(0)).(q)/(r^(2) + l^(2))`
On resolving `E_(1) and E_(2)` into rectangular components, we observe that vectors `E_(1) sin theta and E_(2) sin theta` are equal in magnitude and opposite to each other and hence cancel out.
The vectors `E_(1) cos theta and E_(2) cos theta` are acting along the same direction and thus add up.
`therefore E_(P)= E_(1) cos theta + E_(2) cos theta` ....(1)
`=2 E_(1) cos theta [ because E_(1)= E_(2)]`
`=(2)/(4pi epsi_(0)) (q)/((r^(2) + l^(2))).(l)/((r^(2) + l^(2))^(1//2)) [ because cos theta= (1)/((r^(2) + l^(2))^(1//2))]`
`= (1)/(4pi epsi_(0)).(2ql)/((r^(2) + l^(2))^(3//2))`
`therefore E_(P)= (1)/(4pi epsi_(0)).(|p|)/((r^(2) + l^(2))^(3//2)) [ because |p| = q xx 21]`
The net electric field will be in the opposite direction of p, i.e., opposite to AB.
`E_("equatorial")= - (1)/(4pi epsi_(0)).(p)/((r^(2)+ l^(2))^((3)/(2))) hat(p)`
As `l lt lt r`, so l can be neglected in denominator part.
`E_("equatorial")= (1)/(4pi epsi_(0)).(p)/(r^(3)) hat(p) rArr |E_("equatorial")|= (1)/(4pi epsi_(0)) .(p)/(r^(3))` ...(1)
The direction of electric field at any point on the equatorial line of dipole will be anti-parallel to the dipole moment.