Calculate the electric field due to a dipole on its equatorial plane. (OR) Electric field due to an electric dipole at a point on the equatorial planeSolution in Tamil

Video Solution

Text Solution

Verified by Experts

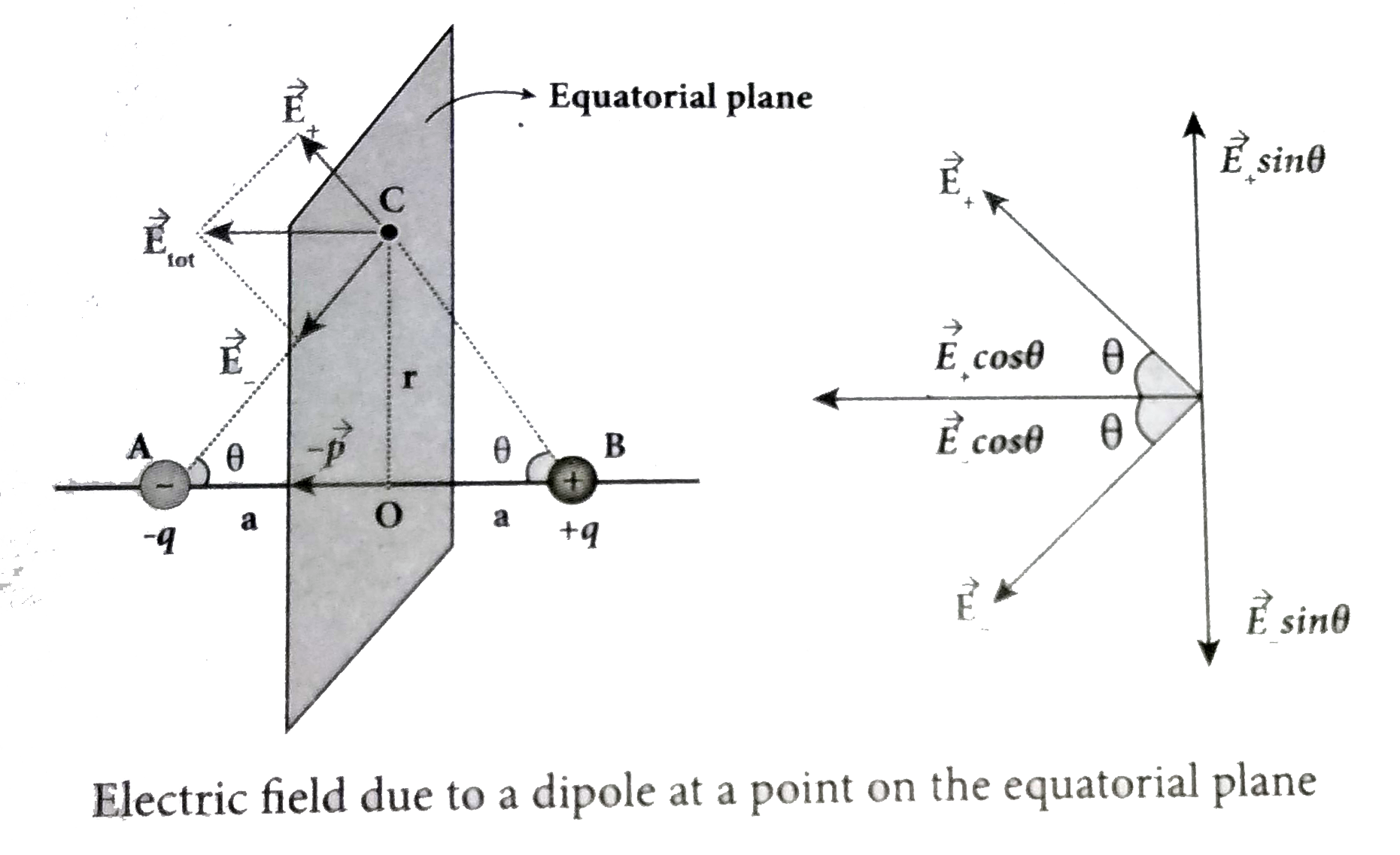
Consider a point C at a distance r from the midpoint O of the dipole equatorial plane on as shown in Figure. Since the point C is equidistant from $+ q$ and $- q$ the magnitude of the electric fields of $+ q$ and $- q$ are the same. The direction of $\to E (+)$ is along BC and the direction of ${\to E}_{-}$ is alongCA. ${\to E}_{+}$ and $\to - - - (-)$ are resolved into twocomponents, one component parallel to the dipole axis and the other perpendicular to it. The perpendicular components of $∣ ∣ {\to E}_{+} ∣ ∣ sin θ$ and ${\to E}_{-} sin θ$ are oppositely directed and cancel each other. The magnitude of the total electric field at point C is the sum of the parallel components of ${\to E}_{+}$ and ${\to E}_{-}$ and its direction is along $ˆ p$
${\to E}_{\to t} = - | E_{+} | cos θ ˆ p - ∣ ∣ {\to E}_{-} ∣ ∣ cos θ ˆ p$ ldots(1)
The magnitudes ${\to E}_{+}$ and ${\to E}_{-}$ are the same and are given by
$∣ ∣ {\to E}_{+} ∣ ∣ = ∣ ∣ {\to E}_{-} ∣ ∣ = \frac{1}{4 π ε_{0}} \frac{q}{(r^{2} + a^{2})} ... (2)$ By substituting equation (1) into equation (2), we get
${\to E}_{\to t} = - \frac{1}{4 π ε_{0}} \frac{2 q cos θ}{(r^{2} + a^{2})} ˆ p = \frac{1}{4 π ε_{0}} \frac{2 q a}{{(r^{2} + a^{2})}^{\frac{3}{2}}} ˆ p$
$sin n cos = \frac{a}{\sqrt{r^{2} + a^{2}}}$
${\to E}_{\to t} = - \frac{1}{4 π ε_{0}} \frac{\to p}{{(r^{2} + a^{2})}^{\frac{3}{2}}}$
$sin c e \to p = 2 q a ˆ p ... (3)$ ,
At very large distances $(r > > a)$ , the equation becomes $\to - - - (\to t) = - \frac{1}{4 π ε_{0} r^{3} (r > > a)}$

Updated on:21/07/2023

|

Class 12 PHYSICS SAMPLE PAPER-07 (SOLVED )

Topper's Solved these Questions

Similar Questions

FULL MARKS-SAMPLE PAPER-07 (SOLVED )-(PART - IV)(Answer all the questions.)

Home
Ask Doubt
Profile