Question

Explain the J.J. Thomson experiment to determine the specific charge of electron.

Explanation

In 1887, J. J. Thomson made remarkable improvement in the scope of study of gases in discharge tubes. In the presence of electric and magnetic fields, the cathode rays are deflected. By the variation of electric and magnetic fields, mass normalized charge or the specific charge (charge per unit mass) of the cathode rays is measured.

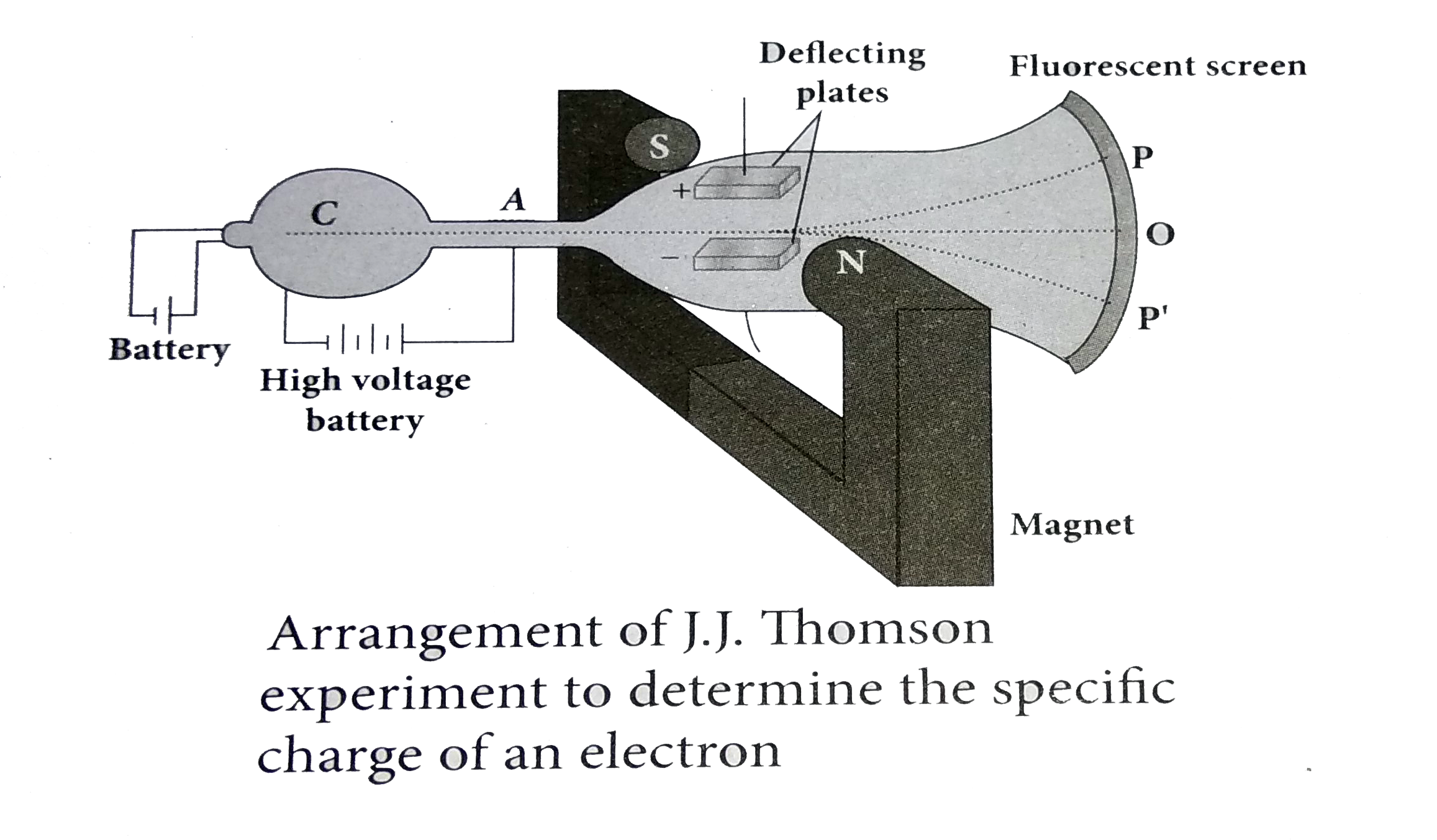
A highly evacuated discharge tube is used and cathode rays (electron beam) produced at cathode are attracted towards anode disc A. Anode disc is made with pin hole in order to allow only a narrow beam of cathode rays. These cathode rays are now allowed to pass through the parallel metal plates, maintained at high voltage. Further, this gas discharge tube is kept in between pole pieces of magnet such that both electric and magnetic fields are perpendicular to each other. When the cathode rays strike the screen, they produce scintillation and hence bright spot is observed. This is achieved by coating the screen with zinc sulphide.
(1) Determination of velocity of cathode rays
For a fixed electric field between the plates, the magnetic field is adjusted such that the cathode rays (electron beam) strike at the original position 0. This means that the magnitude of electric force is balanced by the magnitude of force due to magnetic field. Let e be the charge of the cathode rays, then
`e E = eBv`
`implies(v)=(E)/(B)`ldots(1)

(ii) Determination of specific charge
Since the cathode rays (electron beam) are accelerated from cathode to anode, the potential energy of the electron beam at the cathode is converted into kinetic energy of the electron beam at the anode. Let V be the potential difference between anode and cathode, then the potential energy is eV. Then from law of conservation of energy,
`ev=(1)/(2)mnu^(2)implies(e)/(m)=(nu^(2))/(2V)`
Substituting the value of velocity from equation (1), we get
`(e)/(m)=(1)/(2V)(E^(2))/(B^(2))ldots(2)`
.Substituting the values of E, B and V, the specific charge can be determined as
`(e)/(m)=1.7xx10^(11)Ckg^(-1)`
(iii) Deflection of charge only due to uniform electric field
When the magnetic field is turned off, the deflection is only due to electric field. The deflection in vertical direction is due to the electric force.
` F_(e)= eEldots(3)`
Let m be the mass of the electron and by applying Newton.s second law of motion, acceleration of the electron is
`a_(e)=(1)/(m)F_(e)ldots(4)`
Substituting equation (4) in equation (3),
`a_(e)=(1)/(m)eE=(e)/(m)E`
Let y be the deviation produced from original position on the screen. Let the initial upward velocity of cathode ray be u=0 before entering the parallel electric plates. Let 1 be the time taken by the cathode rays to travel in electric field. Let / be the length of one of the plates, then the time taken is

Hence, the deflection y. of cathode rays is `(note: mu = 0 `and `a_(e) =(e0//(m)E)`
`y.=ut+(1)/(2)at^(2)impliesy=ut+(1)/(2)a_(e)t^(2)=(1)/(2)a_(e)t^(2)=(1)/(2)((e)/(m)E)((l)/(nu))^(2)`
`y.=(1)/(2)(e)/(m)(l^(2)B^(2))/(E)ldots(6)` Therefore, the deflection y on the screen is
`yprop.y.impliesy=Cy.`
where Cis proportionality constant which depends on the geometry of the discharge tube andsubstituting y. value in equation (6), we get
`y=C(1)/(2)(e)/(m)(l^(2)B^(2))//(E)ldots(7)`
Rearranging equation (7) as
`(e)/(m)=(2yeE/(Cl^(2)B^(2)Idots(8))`
Substituting the values on RHS, the value of specific charge is calculated as `(e)/(m)=1.7xx10^(11)Ckg^(-1)`