Show that the following system of linear inequalities has no solutin x+2y≤3,3x+4y≥12,x≥0,y≥1.
Video Solution
Text Solution
The correct Answer is:N/a
First inequation : x−2y≤3
Corresponding : x−2y=3
This line passes through the points A (3, 0) and B(0,−32). Join AB .
At point (0, 0), from the inequatioin, 0≤3 (True )The solution of this inequation is that region of XY-plane dividing by line AB in which (0, 0) lies .
Second inequation : 3x+4y≥12
Corresponding equation : 3x+4y=12
This lines passes through the points C(4, 0) and D (0, 3). Join CD.
At point (0, 0) , from the inequation, 0≥12 (False) The solution of this inequation is that region of XY-plane divided by line CD in which (0, 0) does not lie .
The solution of x≥oisx=0 and its right side.
The solution of y≥1isy=1 and its above.
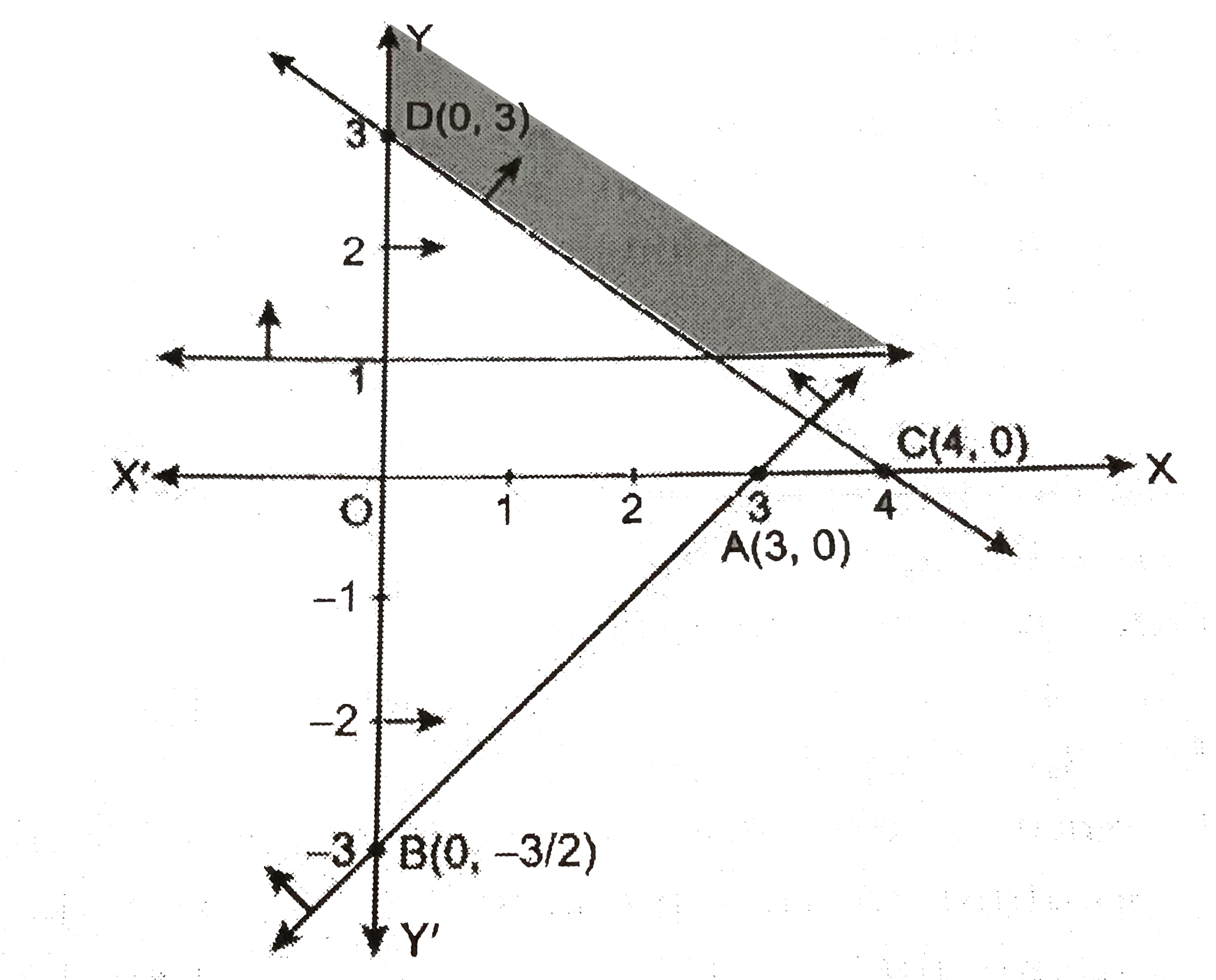
∴ The common solution of given inequation is shown by the shaded region.
|
Topper's Solved these Questions
- LINEAR INEQUALITIESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - LINEAR INEQUALITIESEXERCISE - MISCELLANEOUS EXERCISE
14 Videos
- LINEAR INEQUALITIESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - LINEAR INEQUALITIESEXERCISE - EXERCISE
76 Videos
- LIMITS AND DERIVATIVESBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - LIMITS AND DERIVATIVESEXERCISE - Miscellaneous Exercise
30 Videos
- MATHEMATICAL REASONING BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - MATHEMATICAL REASONING EXERCISE - Misellaneous exercise
7 Videos
Similar Questions
Show that the following system of linear inequalities has no solution x+2y≤3,3x+4y≥12,x≥0,y≥1.
View Solution3x+2y≤12,x≥1,y≥2
View Solutiony−2x≤1,x+y≤2,x≥0,y≥0
View Solutionx−y≤2,x+y≤4,x≥0,y≥0
View Solution2x+y≤6,x+2y≤8,x≥0,y≥0
View Solution2x+y≥6,x+2y≥8,x≥0,y≥0
View Solutionx+y≥24,2x+y≥32,x≥0,y≥0
View SolutionShow that the solution set of the following system of linear inequalities is an unbounded region 2x+y≥8,x+2y≥10,x≥0,y≥0.
View Solutionx+y≤6,x+y≥4
View Solutionx+y≤10,4x+3y≤24,x≥0,y≥0
View Solution
