P and Q are the mid-point of the opposite sides AB and CD of a parallelogram ABCD. AQ intersects DP at S and BQ intersects CP at R. Show that PQRS is a parallelogram.
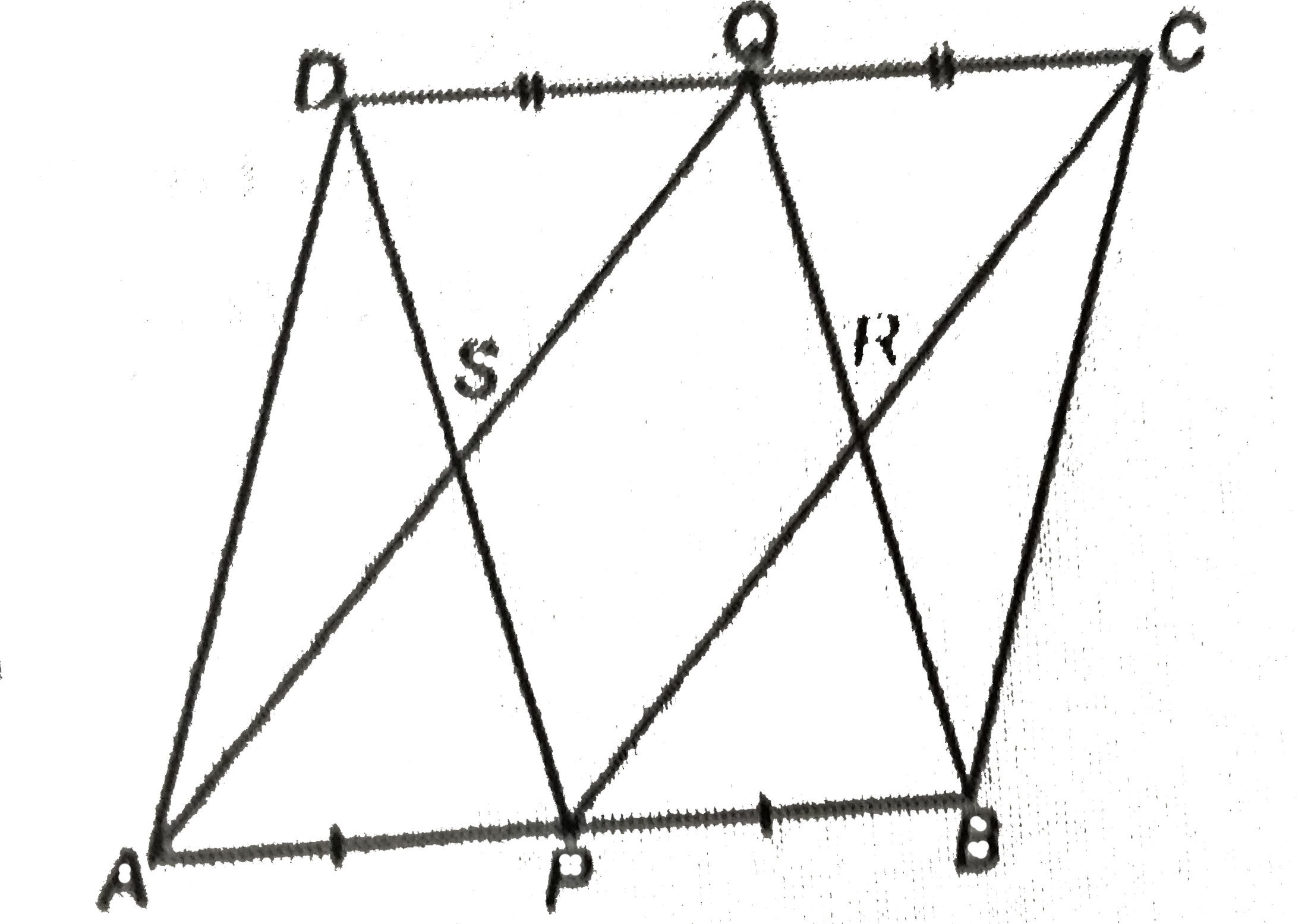
Given: A parallelogram ABCD in which P and Q are the mid-points of the sides AB and CD respectively. AQ intersects DP at S and BQ interects CP respectively. AQ intersects DP at S and BQ intersects Cp at R.
To prove: PRQS is a parallelogram.
Proof: DC||AB (becauseopposite sides of a parallelogram are parallel)
⇒ AP||QC
DC||AB (∵opposite sides of a parallelogram are equal)
⇒ 12DC=12AB
⇒ QC=AP (∵P is mid-point of AB and Q is mid-points of CD)
⇒ APCQis a parallelogram. (∵AP||QCandQC=AP)
∴ AQ||PC (∵opposite sides of a parallelogram are parallel)
⇒ SQ||PR
Similarly, SP||QR
∴ Quadrilateral PQRS is a parallelogram.
Topper's Solved these Questions
- QUADRILATERALSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - QUADRILATERALSEXERCISE - Exercise 8a
29 Videos
- QUADRILATERALSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - QUADRILATERALSEXERCISE - Exercise 8b
15 Videos
- QUADRILATERALSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - QUADRILATERALSEXERCISE - Revision Exercise (long Answer Questions)
5 Videos
- PROBABILITYBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - PROBABILITYEXERCISE - Revision Exercise (very Short Answer /short Answer Questions)
10 Videos
- STATISTICSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICSEXERCISE - Revision Exercise
12 Videos
Similar Questions
ABCD is a parallelogram in which P and Q are mid-points of opposite sides AB and CD (see Fig. 8.18). If AQ intersects DP at S and BQ intersects CP at R, show that: (i) APCQ is a parallelogram. (ii) DPBQ is a parallelogram.
View SolutionIf P and Q are the middle points of the sides BC and CD of the parallelogram ABCD, then AP+AQ is equal to
View SolutionX and Y are the mid-points of the opposite sides AB and DC of a parallelogram ABCD. Prove that □AXCY is a parallelogram.
View SolutionL and M are the mid-points of sides AB and DC respectively of parallelogram ABCD. Prove that segments DL and BM trisect diagonal AC.
View SolutionIf P and Q are the mid points of the sides AB and CD of a parallelogram ABCD, prove that DP and BQ cut the trisection which also the points of trisection of DP and BQ respectively.
View SolutionIn Figure, X,Y are the mid-points of opposite sides AB and DC of a parallelogram ABCD.AY and DX are joined intersecting inP;CX and BY are joined intersecting in Q. Show that DXBY is a parallelogram, PXQY is parallelogram.
View SolutionP and Q are points on opposite sides AD and BC of a parallelogram ABCD such that PQ passes through the point of intersection O of its diagonals AC and BD. Show that PQ is bisected at O.
View SolutionIn Figure, X, Y are the mid-points of opposite sides AB and DC of a parallelogram ABCD.AY and DX are joined intersecting in P;CX and BY are joined intersecting in Q . Show that
(i) AXCY is a parallelogram
(ii) DXBY is a parallelogram
(iii)PXQY is a parallelogram
View SolutionE is a point on the side AD produced of a parallelogram ABCD and BE intersects CD at F. Show that ΔABE~ΔCFB .
View SolutionE is a point on the side AD produced of a parallelogram ABCD and BE intersects CD at F. Show that ΔABE~ΔCFB.
View Solution
