In figure, P is the mid-point of side BC of a parallelogram ABCD such that ∠BAP=∠DAP. Prove that AD = 2CD. 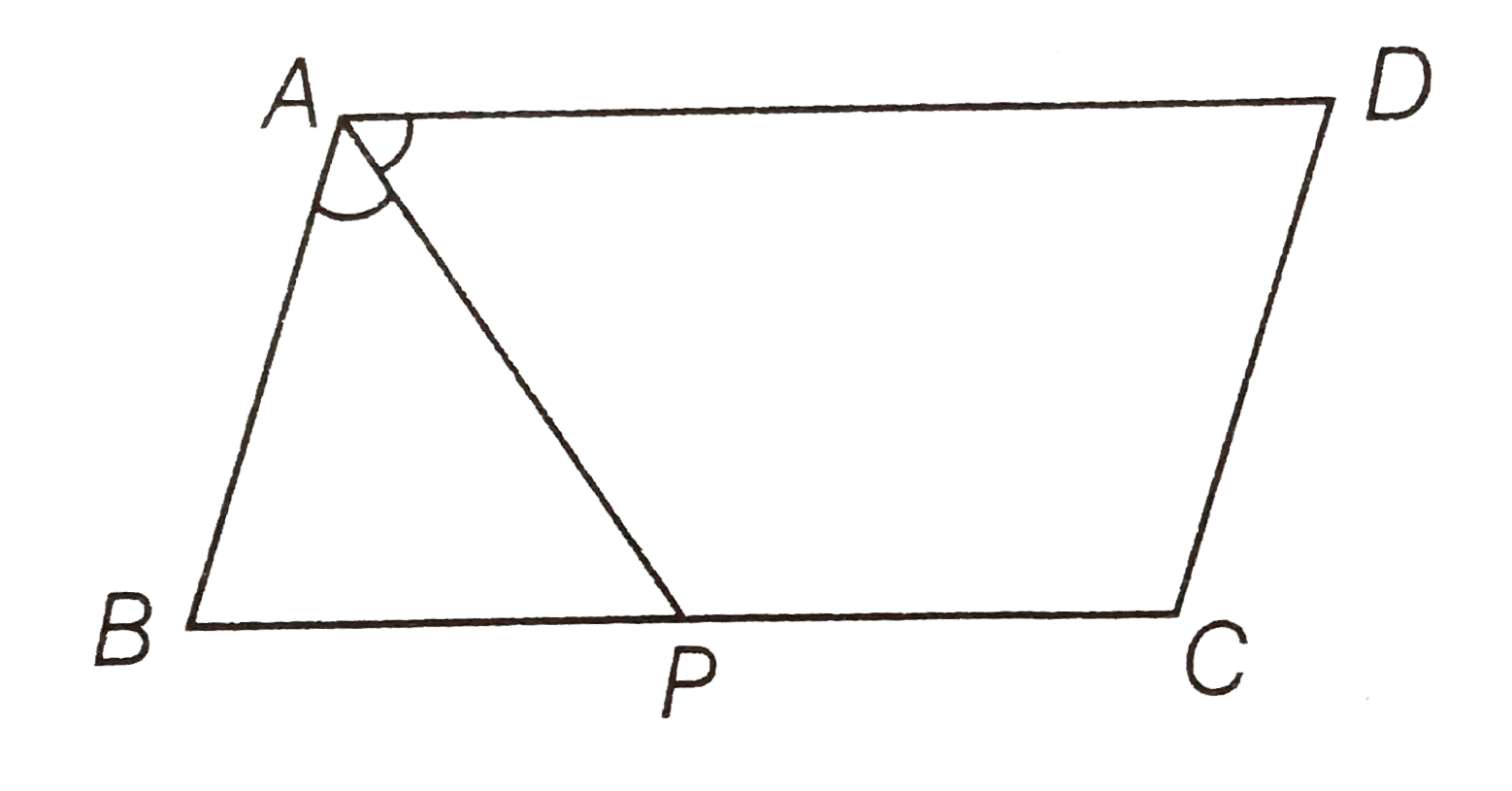
angleBAP=angleDAP=1/2angleA" "…(1)
Since, ABCD is a parallelogram, we have
∠DAB+∠ABC=180∘ ..(2)
(∵sum of co-interior angles on the same side of transversal is180∘)
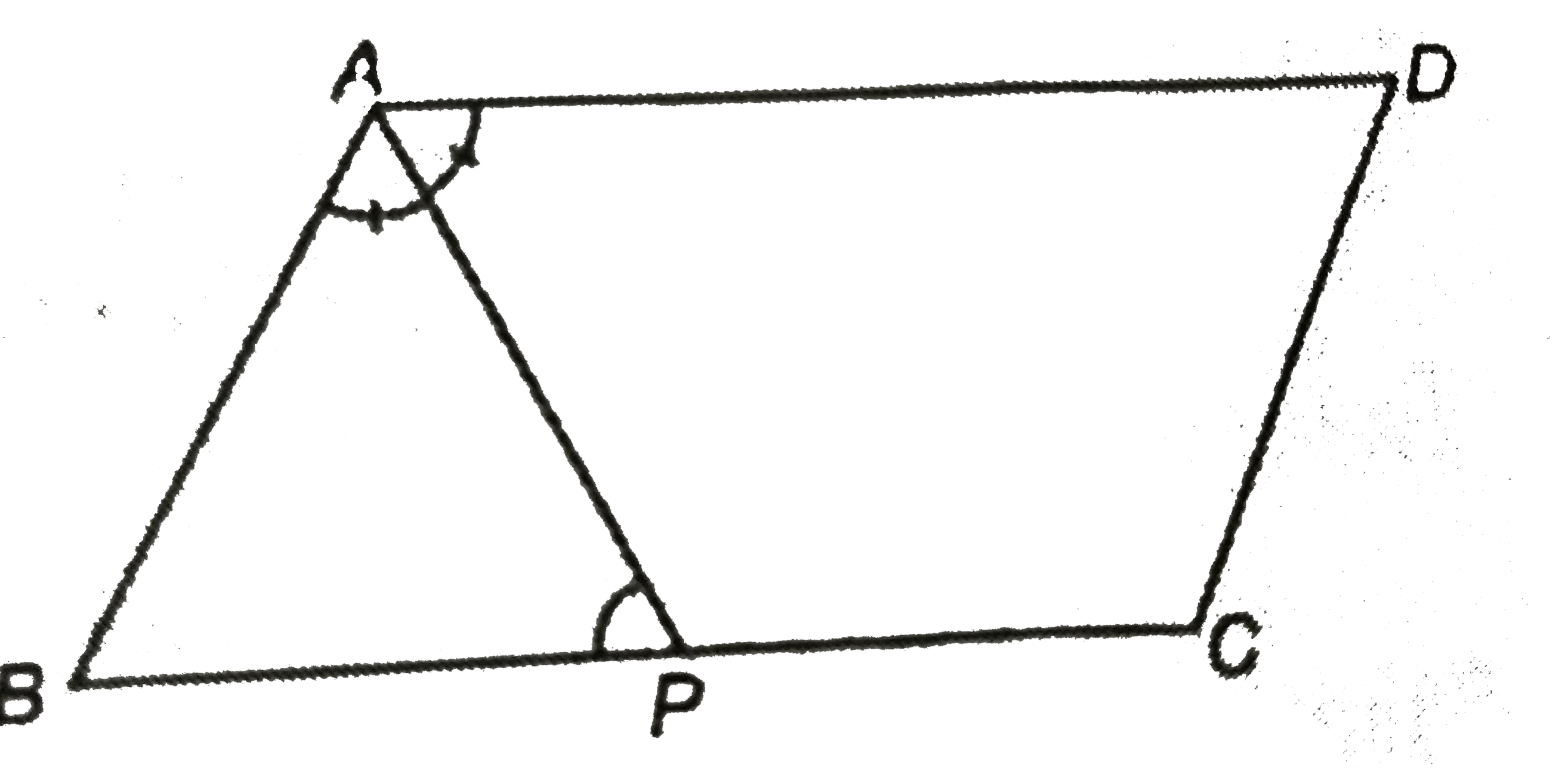
In ΔABP we have
∠BAP+∠B+∠APB=180∘ (angle sum property)
⇒12∠BAD+180∘−∠A+∠APB=180∘
⇒ ∠APB−12∠A [using (1) and (2)]
From (1) and (3), we get ...(3)
∠BAP=∠APB
BP=AB(∴side opposite to equal angles are equal)...(4)
Since, opposite sides of a parallelogram are equal, we have
AD=BC⇒12AD=12BC
⇒ 12AD=BP (∵Pis the mid-point of BC)
⇒ 12AD=AB [∵from(4)]
Since, opposite sides of a parallelogram are equal, we have
12AD=CD⇒AD=2CD
Topper's Solved these Questions
- QUADRILATERALSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - QUADRILATERALSEXERCISE - Exercise 8a
29 Videos
- QUADRILATERALSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - QUADRILATERALSEXERCISE - Exercise 8b
15 Videos
- QUADRILATERALSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - QUADRILATERALSEXERCISE - Revision Exercise (long Answer Questions)
5 Videos
- PROBABILITYBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - PROBABILITYEXERCISE - Revision Exercise (very Short Answer /short Answer Questions)
10 Videos
- STATISTICSBOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - STATISTICSEXERCISE - Revision Exercise
12 Videos
Similar Questions
The angles of a quadrilateral are 89∘and113∘ If the other two angles are equal, find the equal angles.
View SolutionThe sides AB and AC are equal of an isosceles triangle ABC. D E and F are the mid-points of sides BC, CA and AB respectively. Prove that:
(i) Line segment AD is perpedicular to line segment EF.
(ii) Line segment AD bisects the line segment EF.
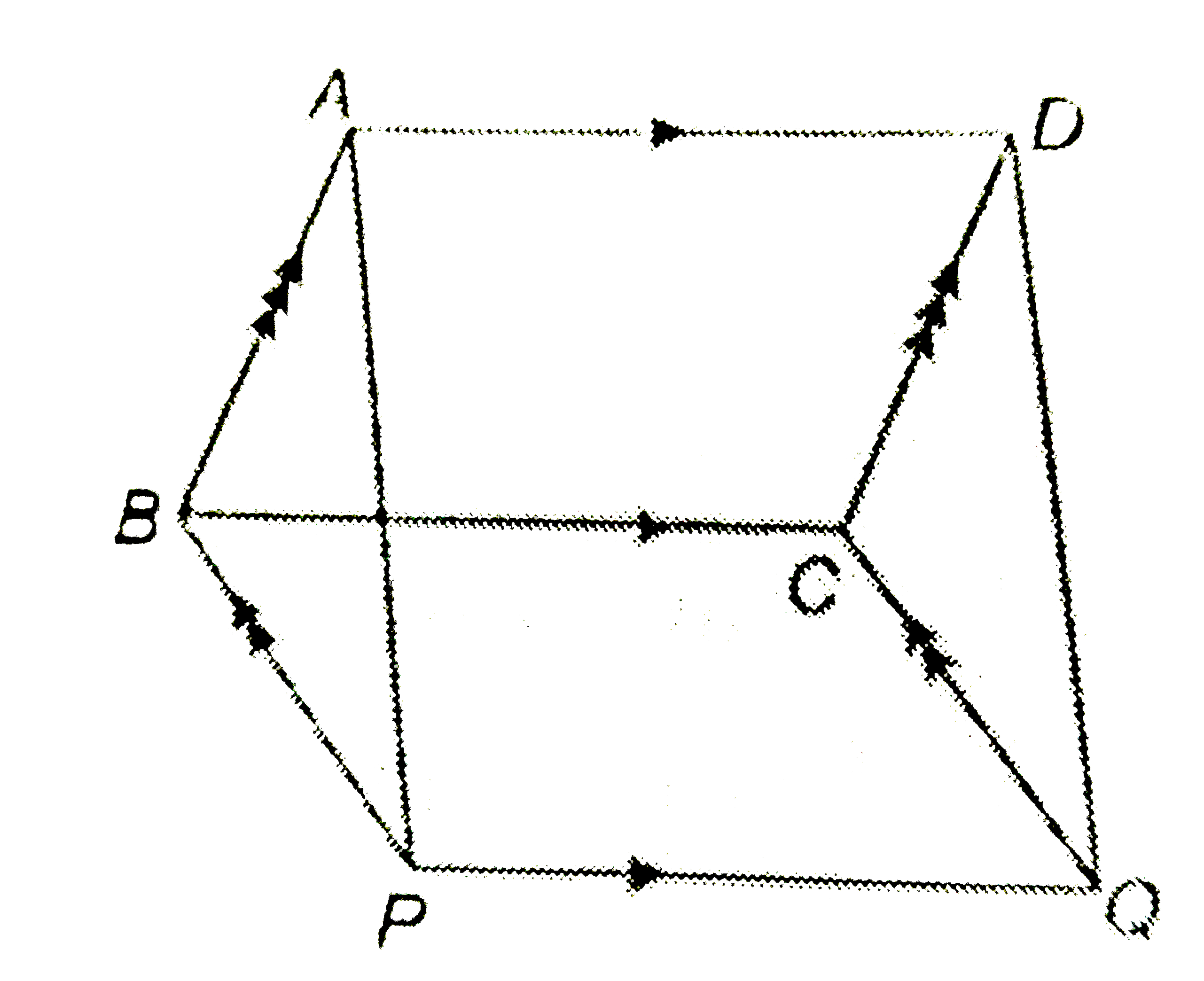
View SolutionIn the adjoining figure, ABCD and PBCQ are paralelograms. Prove that
ΔABP≅ΔDCQ
View SolutionWhat is the probability of an impossible event?
View SolutionIf the heights of 5 persons are 140 cm, 150 cm, 152 cm, 158 cm and 161 cm respectively, find the mean height.
View SolutionIn figure, P is the mid-point of side BC of a parallelogram ABCD such that ∠BAP=∠DAP. Prove that AD = 2CD. ltBrgt

View SolutionIn the given figure, P is the mid-point of side BC of a parallelogram ABCD such that ∠BAP=∠DAP. Prove that AD = 2CD.
View SolutionHow many lines of symmetry does the above figure have ?
View SolutionDetermine the point of symmetry of a regular hexagon.
View SolutionDtermine the images of the following figure about the given line :
View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionFind the area of the figure given below.

View SolutionThe area of the shaded part in the figure given below is ______.

View SolutionThe inequation represented by the graph given below is :
View Solution
