Consider two electric dipoles P1 and P2 placed at (0, 0) and (1,√2 ) respectively. The centers of these dipoles are fixed so that they can rotate about their centers but the centers cannot move. Answer the following questions based on this arrangement.
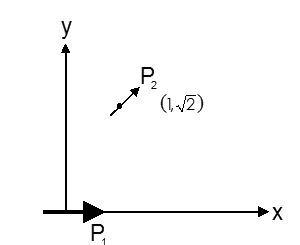
Initially P1 is held such that it is pointing towards positive x-axis and P2 is free to rotate. In the equilibrium position, P2 will point :
both along x-axis
both along y-axis
both along line joining P1 and P2
at 90° with each other
The correct Answer is:C
If process is repeated infinite times. Equilibrium will be in minimum potential energy state
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - JEE MAIN65videos
ELECTROSTATICS
- BOOK - VMC MODULES ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - JEE Advanced (Archive)89videos
ELECTROSTATICS
- BOOK - VMC MODULES ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - LEVEL - 1105videos
ELECTROSTATICS
- BOOK - VMC MODULES ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT EXERCISE - IMPECCABLE52videos
ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT
- BOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - JEE ADVANCE (ARCHIVE) - TRUE/FALSE TYPE1videos
ENERGY & MOMENTUM
Similar Questions
Consider two electric dipoles P1 and P2 placed at (0, 0) and (1,√2 ) respectively. The centers of these dipoles are fixed so that they can rotate about their centers but the centers cannot move. Answer the following questions based on this arrangement.
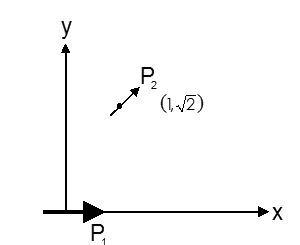
Now P2 is fixed in the equilibrium position obtained in last question and P1 is released so that it can rotate freely. In its equilibrium position P1 will point along:View SolutionTwo short dipoles pˆk and P2ˆk are located at (0,0,0) & (1m,0,2m) respectivley. The resultant electric field due to the two dipoles at the point (1m,0,0) is
View Solution
Knowledge Check
- Question 1 - Select One
Electric field intensity (E) due to an electric dipole varies with distance (r ) from the point of the center of dipole as :
A1rand1r2B1r2and1rC1r3and1r3D1r3and1r2
Two short dipoles pˆk and P2ˆk are located at (0,0,0) & (1m,0,2m) respectivley. The resultant electric field due to the two dipoles at the point (1m,0,0) is
View SolutionIn the figure, two point charges +qand−q are placed on the x-axis at (+a,0)and(−a,0) respectively. A tiny dipole of dipolem moment (p) is kept at the origin along the y-axis. The torque on the dipole equals
 View Solution
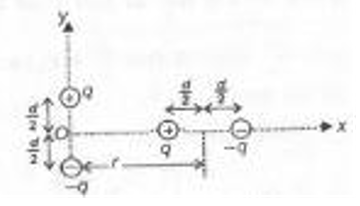
View SolutionTwo identical electric dipoles of dipole moments p are placed as shown in the figure. The electrostatic force on dipole placed along x-axis is (d << r) (14πε0 )
 View Solution
View SolutionThe coordinates of the point AandB are (a,0) and (−a,0), respectively. If a point P moves so that PA2−PB2=2k2, when k is constant, then find the equation to the locus of the point P.
View Solution
Two point electric dipoles with dipole moment P1 and P2 are separated by a distance r with their dipole axes mutually perpendicular as shown. The force of interaction between the dipoles is (where k=14πε0)View SolutionA tiny electric dipole of dipole moment →P=P0ˆj is placed at point (l,0). There exists an electric field →E=2ax2ˆi+(2by2+2cy)ˆj.
View SolutionThe coordinates of the point A and B are (a,0) and (−a,0), respectively. If a point P moves so that PA2−PB2=2k2, when k is constant, then find the equation to the locus of the point P.
View Solution
