A magnetic dipole is acted upon by two magnetic fields with inclined to each other at an angle of 75∘. One of the fields has a magnitude of 15 mT. The dipole attains stable equilibrium at an angle of 30∘ with this field. The magnitude of the other field (in mT) is close to
1
11
36
1060
The correct Answer is:B
To solve the problem, we need to analyze the magnetic dipole in the presence of two magnetic fields. Here’s the step-by-step solution:
Step 1: Understand the Problem
We have two magnetic fields, B1 and B2, inclined at an angle of 75∘ to each other. The dipole is in stable equilibrium at an angle of 30∘ with B1, which has a magnitude of 15mT.
Step 2: Identify Angles
Since the two magnetic fields are inclined at 75∘, and the dipole makes an angle of 30∘ with B1, we can find the angle θ2 that the dipole makes with B2:
θ2=75∘−30∘=45∘
Step 3: Write the Torque Equation
For the dipole to be in stable equilibrium, the net torque acting on it must be zero. The torque (τ) due to a magnetic field is given by:
τ=mBsinθ
where m is the magnetic moment, B is the magnetic field strength, and θ is the angle between the magnetic moment and the magnetic field.
Step 4: Set Up the Torque Balance Equation
Since the torques due to both fields must be equal for equilibrium, we can write:
mB1sin(30∘)=mB2sin(45∘)
We can cancel m from both sides since it is common:
B1sin(30∘)=B2sin(45∘)
Step 5: Substitute Known Values
Substituting B1=15mT, sin(30∘)=12, and sin(45∘)=1√2:
15⋅12=B2⋅1√2
Step 6: Solve for B2
Now, we can solve for B2:
152=B2√2
Multiplying both sides by √2:
B2=15√22
Step 7: Calculate the Numerical Value
Calculating B2:
B2=15⋅1.4142≈21.212≈10.605mT
Rounding this gives approximately 11mT.
Final Answer
The magnitude of the other field B2 is approximately 11mT.
---
We have two magnetic fields, B1 and B2, inclined at an angle of 75∘ to each other. The dipole is in stable equilibrium at an angle of 30∘ with B1, which has a magnitude of 15mT.
Since the two magnetic fields are inclined at 75∘, and the dipole makes an angle of 30∘ with B1, we can find the angle θ2 that the dipole makes with B2:
θ2=75∘−30∘=45∘
For the dipole to be in stable equilibrium, the net torque acting on it must be zero. The torque (τ) due to a magnetic field is given by:
τ=mBsinθ
where m is the magnetic moment, B is the magnetic field strength, and θ is the angle between the magnetic moment and the magnetic field.
Since the torques due to both fields must be equal for equilibrium, we can write:
mB1sin(30∘)=mB2sin(45∘)
We can cancel m from both sides since it is common:
B1sin(30∘)=B2sin(45∘)
Substituting B1=15mT, sin(30∘)=12, and sin(45∘)=1√2:
15⋅12=B2⋅1√2
Now, we can solve for B2:
152=B2√2
Multiplying both sides by √2:
B2=15√22
Calculating B2:
B2=15⋅1.4142≈21.212≈10.605mT
Rounding this gives approximately 11mT.
The magnitude of the other field B2 is approximately 11mT.
Topper's Solved these Questions
- MAGNETIC EFFECTS OF CURRENT Book:VMC MODULES ENGLISHChapter:MAGNETIC EFFECTS OF CURRENT Exercise:JEE Advanced (Archive)
Explore 78 Videos
- MAGNETIC EFFECTS OF CURRENT Book:VMC MODULES ENGLISHChapter:MAGNETIC EFFECTS OF CURRENT Exercise:LEVEL 2
Explore 50 Videos
- LIQUIDSBook:VMC MODULES ENGLISHChapter:LIQUIDSExercise:JEE ADVANCED (LEVEL -2)
Explore 55 Videos
- MOCK TEST 1Book:VMC MODULES ENGLISHChapter:MOCK TEST 1Exercise:PART I : PHYSICS (SECTION-2)
Explore 10 Videos
Similar Questions
Knowledge Check
A magnetic dipole is under the influence of two magnetic fields. The angle between the field direction is 60∘ and one of the fields has magnitude of 1.2×10−2T. If the dipole comes to stable equilibrium at an angle of 30∘ with this Held, then the magnitude of the field is
A1.2×10−4TB2.4×10−4TC1.2×10−2TD2.4×10−2T
Why don't two magnetic field lines intersect each other?
View SolutionA magnetic dipole is under the influence of two magnetic fields. The angle between the field directions is 60∘ and one of the fields has a magnitude of 1⋅2×10−2 tesla. If the dipole comes to stable equilibrium at an angle of 15∘ with this field, figure, what is the magnitude of the other field?
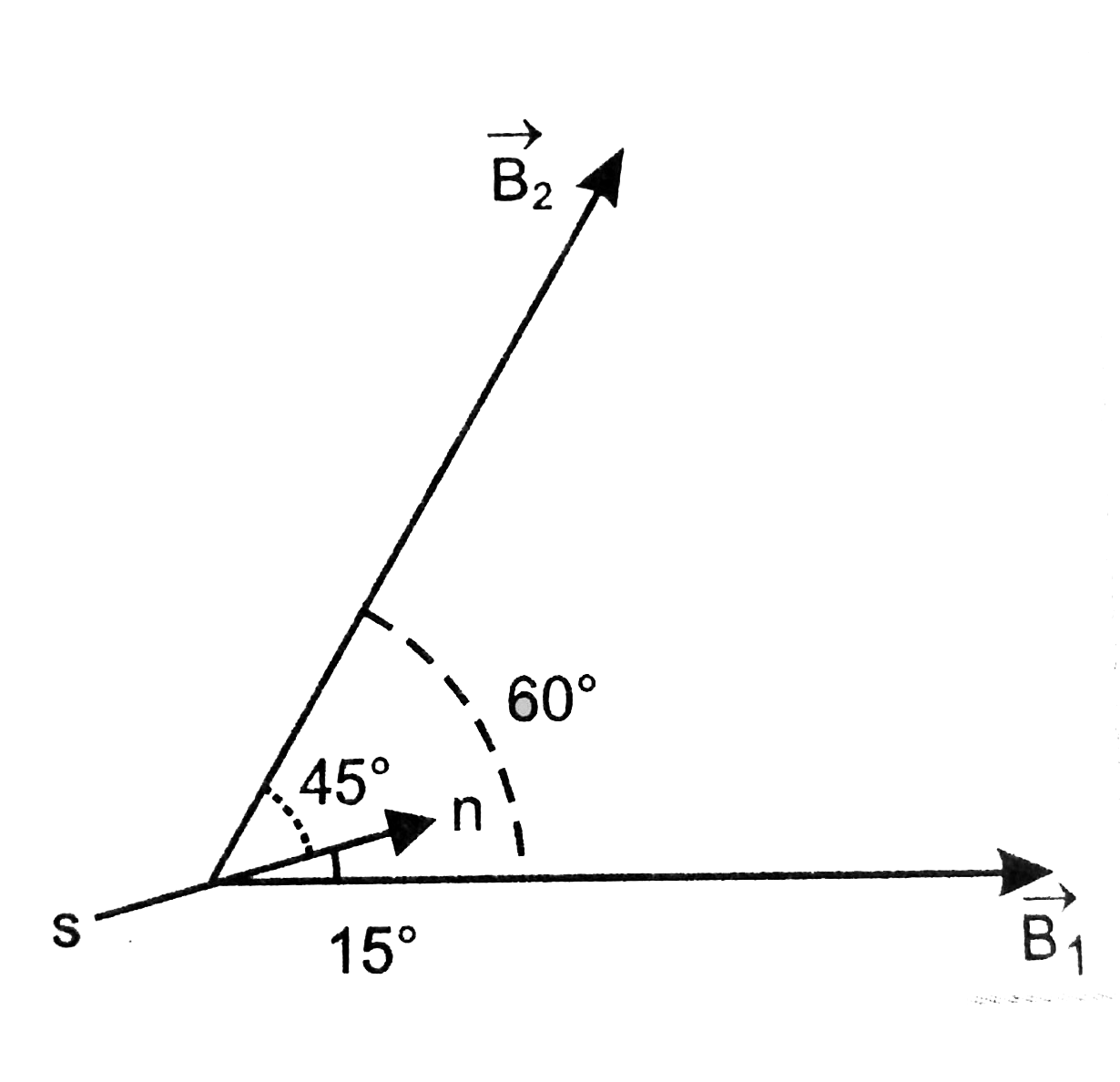
View SolutionThe amplitudes of electric and magnetic fields are related to each other as
View SolutionTwo equal forces (P each) act at a point inclined to each other at an angle of 120∘. The magnitude of their resultant is
View SolutionAn electric dipole is placed in an uniform electric field with the dipole axis making an angle θ with the direction of the electric field. The orientation of the dipole for stable equilibrium is
View SolutionAn electric dipole is plced at an angle of 30∘ to a nonuniform electric field. The dipole will experience
View SolutionA charge particle of charge 'q' and mass 'm' enters in a given magnetic field 'B' and perpendicular to the magnetic field as shown in the figure. It enters at an angle of 60∘ with the boundary surface of magnetic field and comes out at an angle of 30∘ with the boundary surface of the magnetic field as shown in the figure. Find width 'd' in which magnetic field exist.
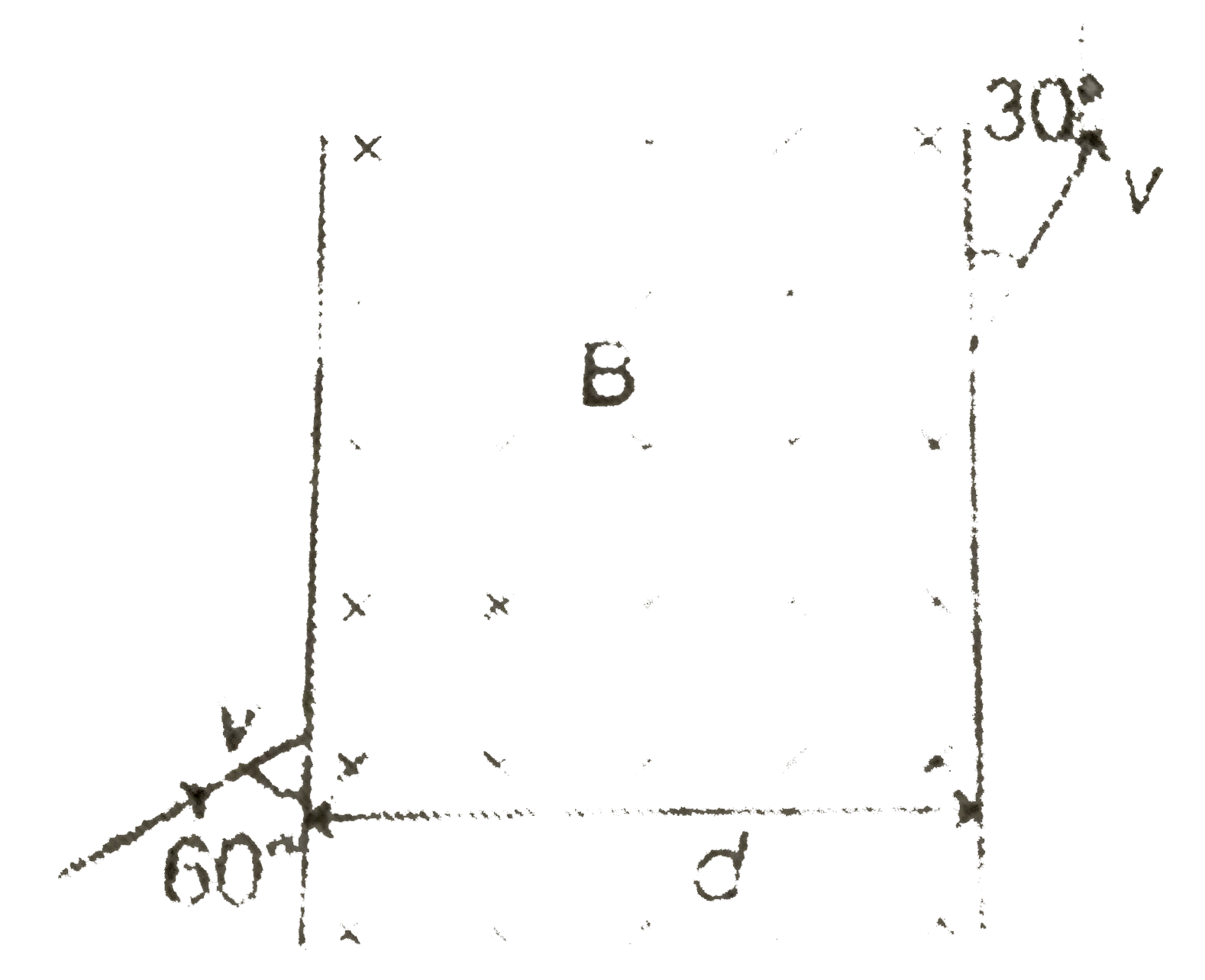
View Solution