A particle of charge −16×10−18 coo–mb moving with velocity 10ms−1 along the x−aξs, and an electric field of magnitude (104)/(m) is along the negative z−ais. If the charged particle continues moving along the x- axis , the magnitude of B is
103Wb/m2
105Wb/m2
1016Wb/m2
10−3Wb/m2
The correct Answer is:D
To solve the problem, we need to find the magnitude of the magnetic field B acting on a charged particle moving in an electric field. The steps to solve the problem are as follows:
Step 1: Understand the Forces Acting on the Charged Particle
The charged particle experiences two forces: the electric force FE due to the electric field E and the magnetic force FB due to the magnetic field B. For the particle to continue moving along the x-axis without deflection, these two forces must be equal in magnitude and opposite in direction.
Step 2: Write the Expression for Electric Force
The electric force FE acting on the charged particle can be expressed as:
FE=Q⋅E
where Q is the charge of the particle and E is the magnitude of the electric field.
Step 3: Write the Expression for Magnetic Force
The magnetic force FB acting on the charged particle can be expressed as:
FB=Q⋅v⋅B
where v is the velocity of the particle and B is the magnitude of the magnetic field.
Step 4: Set the Forces Equal
Since the particle is undeflected, we can set the magnitudes of the electric force and magnetic force equal to each other:
Q⋅E=Q⋅v⋅B
Step 5: Cancel the Charge
Since the charge Q is non-zero, we can cancel it from both sides of the equation:
E=v⋅B
Step 6: Solve for the Magnetic Field B
Rearranging the equation gives us:
B=Ev
Step 7: Substitute the Given Values
Now we can substitute the given values into the equation:
- E=104N/C
- v=10m/s
Thus,
B=10410=103T
Step 8: Conclusion
The magnitude of the magnetic field B is 103T.
Final Answer
The magnitude of B is 103T.
---
The charged particle experiences two forces: the electric force FE due to the electric field E and the magnetic force FB due to the magnetic field B. For the particle to continue moving along the x-axis without deflection, these two forces must be equal in magnitude and opposite in direction.
The electric force FE acting on the charged particle can be expressed as:
FE=Q⋅E
where Q is the charge of the particle and E is the magnitude of the electric field.
The magnetic force FB acting on the charged particle can be expressed as:
FB=Q⋅v⋅B
where v is the velocity of the particle and B is the magnitude of the magnetic field.
Since the particle is undeflected, we can set the magnitudes of the electric force and magnetic force equal to each other:
Q⋅E=Q⋅v⋅B
Since the charge Q is non-zero, we can cancel it from both sides of the equation:
E=v⋅B
Rearranging the equation gives us:
B=Ev
Now we can substitute the given values into the equation:
- E=104N/C
- v=10m/s
B=10410=103T
The magnitude of the magnetic field B is 103T.
The magnitude of B is 103T.
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - MAGNETIC EFFECTS OF CURRENT EXERCISE - JEE Advanced (Archive)78videos
MAGNETIC EFFECTS OF CURRENT
- BOOK - VMC MODULES ENGLISHCHAPTER - MAGNETIC EFFECTS OF CURRENT EXERCISE - LEVEL 250videos
MAGNETIC EFFECTS OF CURRENT
- BOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - JEE ADVANCED (LEVEL -2)55videos
LIQUIDS
- BOOK - VMC MODULES ENGLISHCHAPTER - MOCK TEST 1EXERCISE - PART I : PHYSICS (SECTION-2)10videos
MOCK TEST 1
Similar Questions
For a particle moving along the x-axis, mark the correct statement(s).
View SolutionA charged particle carrying charge q=10μC moves with velocity v1=106ms−1 at angle 45∘ with x-axis in the xy plane and experience a force F1=5√2mN along the negative z-axis. When the same particle moves with velocity v2=106ms−1 along the z-axis, it experiences a force F2 in y-direction.
Find the magnitude of the force F2.View SolutionA particle of charge 1μC& mass 1g moving with a velocity of 4m/s is subjected to a uniform electric field of magnitude 300Vm for 10sec. Then it's final speed cannot be :
View SolutionA charged particle carrying charge q=10μC moves with velocity v1=106,s−1 at angle 45∘ with x-axis in the xy plane and experience a force F1=5√2mN along the negative z-axis. When the same particle moves with velocity v2=106ms−1 along the z-axis, it experiences a force F2 in y-direction.
Find the magnetic field →B.View SolutionA proton is moving with a velocity of 5×105m/s along the Y-direction. It is acted upon by an electric field of intensity 105 V/m along the X-direction and a magnetic field of 1Wb/m2 along the Z-direction. Then the Lorentz force on the particle is :
View SolutionA charge q moves with a velocity 2m/s along x- axis in a unifrom magnetic field B=(ˆi+2ˆj+3ˆk) tesla.
View SolutionA charged particle carrying charge q=1μc moves in uniform magnetic with velocity v1=106m/s at angle 45∘ with x-axis in the xy-plane and experiences a force F1=5√2N along the negative z-axis. When te same particle moves with velocity v2=106m/s along the z-axis it experiences a force F2 in y-direction. Find
a. the magnitude and direction of the magnetic field
b. the magnitude of the force F2.View SolutionA charged particle is moved along a magnetic field line. The magnetic force on the particle is
View SolutionAn electron with kinetic energy 2.5 keV moving along the positive direction of an x axis enters a region in which a uniform electric field of magnitude 10 kV/m is in the negative direction of the y-axis. A uniform magnetic field →B is to be set up to keep the electron moving along the x-axis , and the direction →B of is to be chosen to minimize the required magnitude of →B. In unit-vector notation, what →B should be set up?
View SolutionDraw the acceleration-time graphs for the given velocity-time graphs of a particle moving along x-axis.
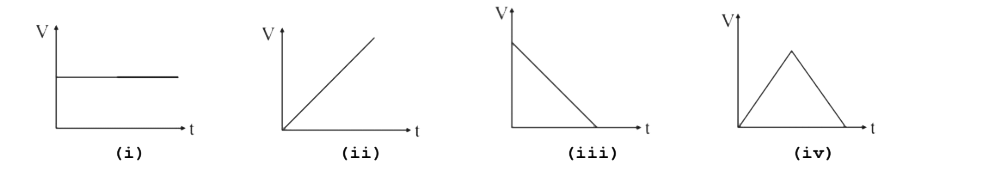 View Solution
View Solution
