A capacitor of capacitance 2μF has a dielectric slab of dielectric constant 8 between its plates. The slab covers the entire volume between the plates. This capacitor is connected to a battery if EMF 20 V and fully charged Now with the battery still connected, the slab is removed from the capactor. during the removal, the work done by the battery is (in mJ).
(a)0.7
(b)−0.7
(c)5.6
(d)−5.6
The correct Answer is:B
To solve the problem step by step, we will follow these calculations:
Step 1: Calculate the initial charge on the capacitor.
The charge Q on a capacitor is given by the formula:
Q=C×V
where:
- C is the capacitance,
- V is the voltage.
Given:
- C=2μF=2×10−6F
- V=20V
Calculating the initial charge:
Q=(2×10−6F)×(20V)=40×10−6C=40μC
Step 2: Calculate the new capacitance after the dielectric slab is removed.
When the dielectric slab is removed, the capacitance C′ of the capacitor changes. The new capacitance is given by:
C′=CK
where K is the dielectric constant.
Given:
- K=8
Calculating the new capacitance:
C′=2μF8=0.25μF=0.25×10−6F
Step 3: Calculate the new charge on the capacitor.
Since the battery remains connected, the voltage across the capacitor remains the same. Therefore, the new charge Q′ can be calculated as:
Q′=C′×V
Calculating the new charge:
Q′=(0.25×10−6F)×(20V)=5×10−6C=5μC
Step 4: Calculate the change in charge.
The change in charge ΔQ is given by:
ΔQ=Q′−Q
Calculating the change in charge:
ΔQ=5μC−40μC=−35μC
Step 5: Calculate the work done by the battery.
The work done W by the battery when charge flows is given by:
W=ΔQ×V
Calculating the work done:
W=(−35×10−6C)×(20V)=−700×10−6J=−0.7mJ
Final Answer:
The work done by the battery is −0.7mJ.
---
The charge Q on a capacitor is given by the formula:
Q=C×V
where:
- C is the capacitance,
- V is the voltage.
- C=2μF=2×10−6F
- V=20V
Q=(2×10−6F)×(20V)=40×10−6C=40μC
When the dielectric slab is removed, the capacitance C′ of the capacitor changes. The new capacitance is given by:
C′=CK
where K is the dielectric constant.
- K=8
C′=2μF8=0.25μF=0.25×10−6F
Since the battery remains connected, the voltage across the capacitor remains the same. Therefore, the new charge Q′ can be calculated as:
Q′=C′×V
Q′=(0.25×10−6F)×(20V)=5×10−6C=5μC
The change in charge ΔQ is given by:
ΔQ=Q′−Q
ΔQ=5μC−40μC=−35μC
The work done W by the battery when charge flows is given by:
W=ΔQ×V
W=(−35×10−6C)×(20V)=−700×10−6J=−0.7mJ
The work done by the battery is −0.7mJ.
Topper's Solved these Questions
- CAPACITORSBook:VMC MODULES ENGLISHChapter:CAPACITORSExercise:LEVEL 2
Explore 40 Videos
- CAPACITORSBook:VMC MODULES ENGLISHChapter:CAPACITORSExercise:JEE Main (Archive) LEVEL-1
Explore 36 Videos
- CAPACITORSBook:VMC MODULES ENGLISHChapter:CAPACITORSExercise:LEVEL 0 (LONG ANSWER TYPE)
Explore 3 Videos
- BASIC MATHEMATICS & VECTORSBook:VMC MODULES ENGLISHChapter:BASIC MATHEMATICS & VECTORSExercise:Impeccable
Explore 50 Videos
- CURRENT ELECTRICITYBook:VMC MODULES ENGLISHChapter:CURRENT ELECTRICITYExercise:IN-CHAPTER EXERCISE-F
Explore 10 Videos
Similar Questions
A parallel plate capacitor is connected across a battery. Now, keeping the battery connected, a dielectric slab is inserted between the plates. In the process,
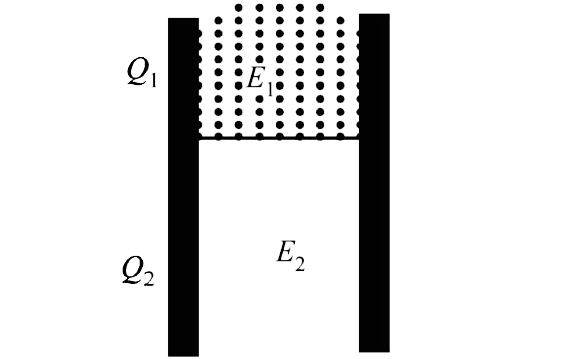
View SolutionA parallel plate capacitor has a dielectric slab of dielectric constant K between its plates that covers 1/3 of the area of its plates, as shown in the figure. The total capacitance of the capacitor is C while that of the portion with dielectric in between is C1. When the capacitor is charged, the plate area covered by the dielectric gets charge Q1 and the rest of the area gets charge Q2. The electric field in the dielectric is E1 and that in the other portion is E2. Choose the correct option/options, ignoring edge effects.
View SolutionA dielectric slab of dielectric constant 3 is inserted into an uncharged capacitor C1. The slab covers the entire volume of the capacitor now, this capacitor and an identical uncharged capacitor C2, with air between the plates are placed in series and connected to a battery. after the capacitor are fully charged, the ratio of the electric field inside them, E1E∘ is:
View SolutionA capacitor is connected to a battery of voltage V. Now a di-electric slab of di-electric constant k is completely inserted between the plates, then the final charge on the capacitor will be : (If initial charge is q0)
View SolutionAfter charging a capacitor the battery is removed. Now by placing a dielectric slab between the plates
View SolutionA dielectric slab of area A and thickness d is inderted between then plates of a capaitor of area 2A with constant speed υ as shown in. Dustance between the plates is (d).
 .
.
The capacitor is connected to a battery of emf E. The current in the ciruit varies with time as.
View SolutionAn air capacitor is first charged through a battery. The charging battery is then removed and a electric slab of dielectric constant K=4 is inserted between the plates. Simultaneously, the distance between the plates is reduced to half, then find change, C,E,V and U.
View SolutionThe capacitance of a capacitor becomes4/3 times its original value if a dielectric slab of thickness t=d/2 is inserted between the plates (d is the separation between the plates). What is the dielectric constant of the slab?
View SolutionTwo identical capacitors A and B shown in the given circuit are joined in series with a battery. If a dielectric slab of dielectric constant K is slipped between the plates of capacitor B and battery remains connected, then the energy of capacitor A will-
View SolutionIn a parallel-plate capacitor, the region between the plates is filled by a dielectric slab. The capacitor is connected to a cell and the slab is taken out. Then
View Solution