Two dielectric slabs of area of cross-section same as the area of the plates are introduced inside a capacitor as shown. Now, the capacitor is charged. If the potential of the upper plate of the capacitor is
and the potential of the lower plate is , the potential at the interface of the two slabs is:
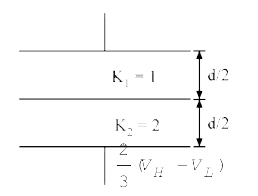
The correct Answer is:A
Let us assume that . This assumption will not affect our answer, and you can test this yourself by taking the opposite assumption and solving.
Let the electric field insie the upper slab be and the electric inside the lower slab be .
Then, we know that
Let the potential at the interface be V.
Then,
Solving, we get
Topper's Solved these Questions
- CAPACITORSBook:VMC MODULES ENGLISHChapter:CAPACITORSExercise:LEVEL 2
Explore 40 Videos
- CAPACITORSBook:VMC MODULES ENGLISHChapter:CAPACITORSExercise:JEE Main (Archive) LEVEL-1
Explore 36 Videos
- CAPACITORSBook:VMC MODULES ENGLISHChapter:CAPACITORSExercise:LEVEL 0 (LONG ANSWER TYPE)
Explore 3 Videos
- BASIC MATHEMATICS & VECTORSBook:VMC MODULES ENGLISHChapter:BASIC MATHEMATICS & VECTORSExercise:Impeccable
Explore 50 Videos
- CURRENT ELECTRICITYBook:VMC MODULES ENGLISHChapter:CURRENT ELECTRICITYExercise:IN-CHAPTER EXERCISE-F
Explore 10 Videos
Similar Questions
Knowledge Check
A slab of material of dielectric constant K has the same area as the plates of a parallel capacitor, but has a thickness (34d),
where d is the separation of the plates. How is the capacitance changed when the slab is inserted between the platesAC=ε0Ad(K+34K)BC=ε0Ad(2KK+3)CC=ε0Ad(KK+3)DC=ε0Ad(4KK+3)
The potentials of the two plates of capacitor are +10V and −10V. The charge on one of the plate is 40C. The capacitance of the capacitor is
View SolutionA dielectric slab fills the lower as shown in. (Take plate area as A).
 .
.
View SolutionA dielectric slab is inserted between the plates of a capacitor. The charge on the capacitor is Q and the magnitude of the induced charge on each surface of the dielectric is Q'.
View SolutionA slab of material of dielectric constant K has the same area as the plates of a parallel capacitor, but has a thickness (34d),
where d is the separation of the plates. How is the capacitance changed when the slab is inserted between the plates
View SolutionTwo capacitors of capacitance 2μF and 3μF are joined in series. Outer plate first capacitor is at 1000 volt and outer plate of second capacitor is earthed (grounded). Now the potential on inner plate of each capacitor will be
View SolutionA parallel plate capacitor has a dielectric slab of dielectric constant K between its plates that covers 1/3 of the area of its plates, as shown in the figure. The total capacitance of the capacitor is C while that of the portion with dielectric in between is C1. When the capacitor is charged, the plate area covered by the dielectric gets charge Q1 and the rest of the area gets charge Q2. The electric field in the dielectric is E1 and that in the other portion is E2. Choose the correct option/options, ignoring edge effects.
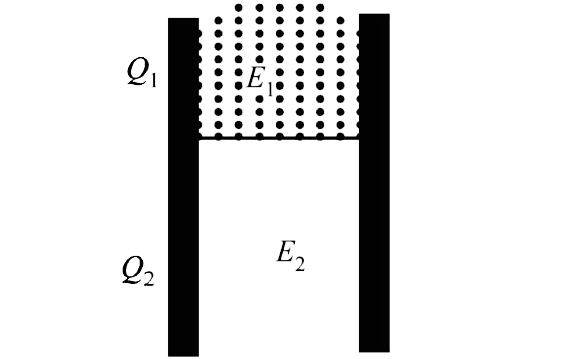
View SolutionThe capacitor shown has square plates of side L and has gap d between the photos. In the dielectric constant of the materiale of the slab is k , the capacitance of the capacitor is

View Solution
_2019_CBT2_E01_002_Q01.png)