Suppose you are a judge and the case under your consideration is : “Whether a car driver was exceeding a speed limit of 40 km/h before he made an emergency stop, the length of the skid marks on the road was 3m? You may assume the maximum deceleration to be 10m/s2? "
To determine whether the car driver was exceeding the speed limit of 40 km/h before making an emergency stop, we can follow these steps:
Step 1: Identify the given values
- Length of skid marks (s) = 3 m
- Maximum deceleration (a) = -10 m/s² (negative because it is deceleration)
- Final velocity (v) = 0 m/s (the car comes to a stop)
Step 2: Use the third equation of motion
The third equation of motion is given by:
v2=u2+2as
Where:
- v = final velocity
- u = initial velocity (what we need to find)
- a = acceleration (deceleration in this case)
- s = distance covered (length of skid marks)
Step 3: Substitute the known values into the equation
Since the final velocity v is 0, we can rewrite the equation as:
0=u2+2(−10)(3)
Step 4: Simplify the equation
This simplifies to:
0=u2−60
Rearranging gives:
u2=60
Step 5: Solve for the initial velocity u
Taking the square root of both sides:
u=√60
Calculating this gives:
u≈7.75m/s
Step 6: Convert the initial velocity to km/h
To convert from meters per second to kilometers per hour, we use the conversion factor 185:
u≈7.75×185
Calculating this gives:
u≈27.9km/h
Step 7: Compare with the speed limit
The speed limit is 40 km/h. Since 27.9 km/h is less than 40 km/h, we conclude that the driver was not exceeding the speed limit.
Final Conclusion
The driver was driving at approximately 27.9 km/h, which is below the speed limit of 40 km/h. Therefore, the driver is not guilty of exceeding the speed limit.
---
- Length of skid marks (s) = 3 m
- Maximum deceleration (a) = -10 m/s² (negative because it is deceleration)
- Final velocity (v) = 0 m/s (the car comes to a stop)
The third equation of motion is given by:
v2=u2+2as
Where:
- v = final velocity
- u = initial velocity (what we need to find)
- a = acceleration (deceleration in this case)
- s = distance covered (length of skid marks)
Since the final velocity v is 0, we can rewrite the equation as:
0=u2+2(−10)(3)
This simplifies to:
0=u2−60
Rearranging gives:
u2=60
Taking the square root of both sides:
u=√60
Calculating this gives:
u≈7.75m/s
To convert from meters per second to kilometers per hour, we use the conversion factor 185:
u≈7.75×185
Calculating this gives:
u≈27.9km/h
The speed limit is 40 km/h. Since 27.9 km/h is less than 40 km/h, we conclude that the driver was not exceeding the speed limit.
The driver was driving at approximately 27.9 km/h, which is below the speed limit of 40 km/h. Therefore, the driver is not guilty of exceeding the speed limit.
Topper's Solved these Questions
- KINEMATICS OF A PARTICLE BOOK - VMC MODULES ENGLISHCHAPTER - KINEMATICS OF A PARTICLE EXERCISE - LEVEL 1
75 Videos
- KINEMATICS OF A PARTICLE BOOK - VMC MODULES ENGLISHCHAPTER - KINEMATICS OF A PARTICLE EXERCISE - LEVEL 2
65 Videos
- JEE MAIN REVISON TEST-23BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISON TEST-23EXERCISE - PHYSICS (SECTION 2)
1 Videos
- LAWS OF MOTION BOOK - VMC MODULES ENGLISHCHAPTER - LAWS OF MOTION EXERCISE - IMPECCABLE
53 Videos
Similar Questions
A driver having a definite reaction time is capable of stopping his car over a distance of 30 m on seeing a red traffic signal, when the speed of the car is 72 km/hr andover a distance of 10 m when the speed is 36 km/hr. Find the distance over which he can stop the car if it were running at a speed of 54 km/hr. Assume that his reaction time and the deceleration of the car remains same in all the three cases.
View SolutionA driver takes 0.20 s to apply the brakes after he sees a need for it. This is called the reaction time of the driver. If he ils driving a car at a speed of 54 km/h and the brakes cause a deceleration of 6.0ms2, find the distance travelled by the car after he sees the need to put the brakes on.
View Solution
Knowledge Check
A car is moving with a constant speed of 60kmh−1 on a straight road. Looking at the rear view mirror, the driver finds that the car following him is at a distance of 100m and is approaching with a speed of 5kmh−1. In order to keep track of the car in the rear, the driver begins to glane alternatively at the rear and side mirror of his car after every 2s till the other car overtakes. If the two cars were maintaining their speeds, which of the following statement (s) is/are correct ?
AThe speed of the car in the rear is 65kmh−1.BIn the side mirror the car in the rear would appear to approach with a speed of 5kmh−1 to the driver of the leading car.CIn the rear view mirror the speed of the approaching car would appear to decrease as the distance between the cars decreases.DIn the side mirror, the speed of the approaching car would appear to increase as the distance between the cars decreases.
The driver of a car moving with a speed of 10ms−1 sees a red light ahead, applies brakes and stops after covering 10 m distance. If the same car were moving with a speed of 20ms−1 the same driver would have stopped the car after covering 30 m distance. Within what distance the car can be stopped if traveling with a velocity of 15ms−1 Assume the same reaction time and the same deceleration in each case
View SolutionA car is moving on a horizontal circular track of radius 0.2 km with a constant speed. If coefficient of friction between tyres of car and road is 0.45, then maximum speed of car may be [Take g=10m/s2]
View SolutionA simple pendulum is suspended from the ceiling of a car taking a turn of radius 10 m at a speed of 36 km/h. Find the angle made by the string of the pendulum with the vertical if this angle does not change during the turn. Take g=10ms2.
View SolutionA car is moving with a constant speed of 60kmh−1 on a straight road. Looking at the rear view mirror, the driver finds that the car following him is at a distance of 100m and is approaching with a speed of 5kmh−1. In order to keep track of the car in the rear, the driver begins to glane alternatively at the rear and side mirror of his car after every 2s till the other car overtakes. If the two cars were maintaining their speeds, which of the following statement (s) is/are correct ?
View SolutionA car is moving with a constant speed of 60kmh−1 on a straight road. Looking at the rear view mirror, the driver finds that the car following him is at a distance of 100m and is approaching with a speed of 5kmh−1. In order to keep track of the car in the rear, the driver begins to glane alternatively at the rear and side mirror of his car after every 2s till the other car overtakes. If the two cars were maintaining their speeds, which of the following statement (s) is/are correct ?
View SolutionA block of mass m height 2h and width 2b rests on a flat car which moves horizontally with constant acceleration a as shown in figure. Determine
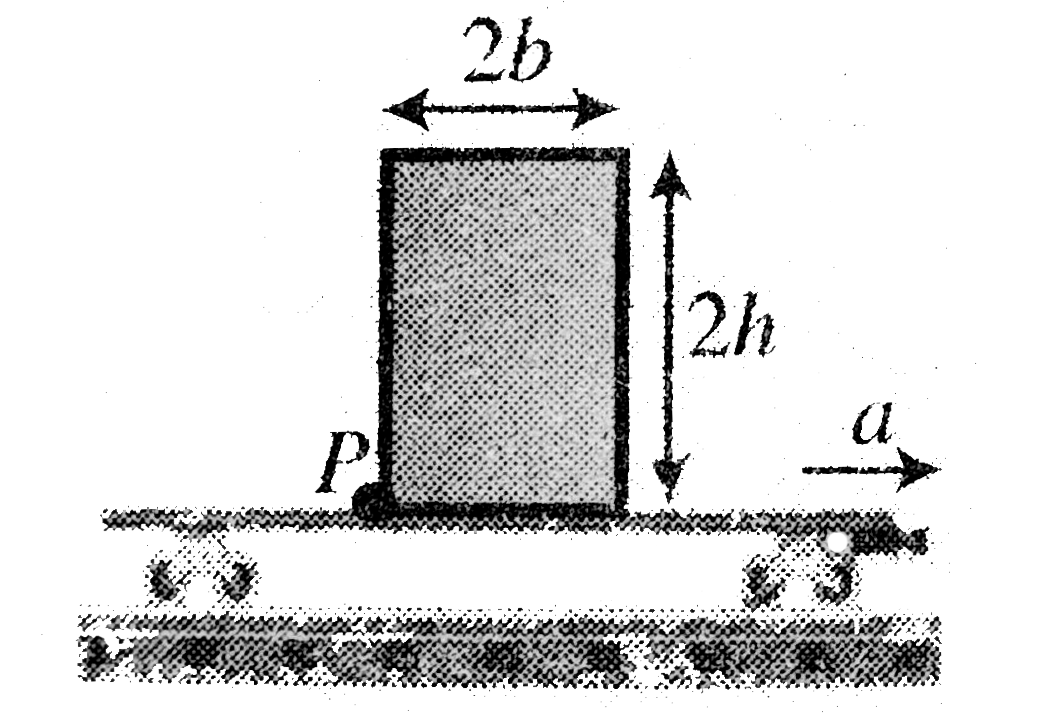
a. the value of the acceleration at which slipping of the block on the car starts, if the coefficient of friction is μ.
b. the value of the acceleration at which block topples about A, assuming sufficient friction to prevet slipping and
c. the shortest distance in which it can be stopped from a speed of 20ms−1 with constant deceleration so that the block is not disturbed. The following data are given b=0.6m,h=0.9m,μ=0.5 and g=10ms−2
View SolutionTen small planes are flying at a speed of 150km/h in total darkness in an air space that is 20×20×1.5km3 in volume. You are in one of the planes, flying at random within this space with no way of knowing where the other planes are, On the average about how long a time will elapse between near collision with your plane. Assume for this rough computation that a safety region around the plane can be approximately by a sphere of radius 10 m.
View Solution
