Question

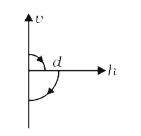
A ball is dropped vertically from a height d above the ground. It hits the ground and bounces up vertically to a height d/2. Neglecting subsequent motion and air resistance, its velocity v varies with the heiht h above the ground as

Options

A.
B.
C.
D.

Answer

A

Explanation

For the given condition initial height h = d and velocity of the ball is zero. When the ball moves downwards its velocity increases and it will be maximum when the ball hits the ground and just after the collision it becomes half and in opposite direction. As the ball moves upward its velocity again decreases and becomes zero at height d/2. This explanation matches with graph (a).Alternate solution
We know for uniformly accelerated / decelerated motion ` v^(2) =u^(2) +-2gh `
Before hitting the ground, the velocity v is given by (quadratic equation and hence parabolic path) Downwards direction means negative velocity. After collision, the direction becomes positive and velocity decreases
` (##VMC_PHY_XI_WOR_BOK_01_C02_E05_006_S01.png" width="80%"> As the direction is reversed and speed is decreased and hence graph (a) represents these conditions correctly.