Acceleration of a particle is given by
a=3t2+2t+1
Where t is time . Find
(i) its velocity at time t assuming velocity to be 10 m/s at t = 0.
(ii) its position at time t assuming that the particle is at origin at t = 0.
(iii) What is the average speed of the particle in the duration t = 0 to t = 1s?
(iv) What is the average acceleration of the particle in the duration t = 0 to t = 1s?
To solve the problem step by step, we will break down each part of the question systematically.
Given:
Acceleration of the particle is given by:
a(t)=3t2+2t+1
(i) Find the velocity at time t assuming velocity is 10m/s at t=0.
1. Start with the relationship between acceleration and velocity:
a=dvdt
This implies:
dv=adt
2. Integrate the acceleration to find the velocity:
v(t)=∫adt=∫(3t2+2t+1)dt
3. Perform the integration:
v(t)=t3+t2+t+C
where C is the constant of integration.
4. Use the initial condition v(0)=10:
v(0)=03+02+0+C=10
Thus, C=10.
5. Final velocity equation:
v(t)=t3+t2+t+10
(ii) Find the position at time t assuming the particle is at the origin at t=0.
1. Start with the relationship between velocity and position:
v=dxdt
This implies:
dx=vdt
2. Integrate the velocity to find the position:
x(t)=∫vdt=∫(t3+t2+t+10)dt
3. Perform the integration:
x(t)=t44+t33+t22+10t+D
where D is the constant of integration.
4. Use the initial condition x(0)=0:
x(0)=0+0+0+0+D=0
Thus, D=0.
5. Final position equation:
x(t)=t44+t33+t22+10t
(iii) Find the average speed of the particle from t=0 to t=1.
1. Average speed is defined as:
Average Speed=Total DistanceTotal Time
2. Calculate the position at t=1:
x(1)=144+133+122+10(1)
x(1)=14+13+12+10
x(1)=312+412+612+10=1312+10=13312
3. Total distance from t=0 to t=1 is x(1)−x(0)=13312−0=13312.
4. Total time is 1−0=1 second.
5. Average speed:
Average Speed=133121=13312m/s≈11.08m/s
(iv) Find the average acceleration of the particle from t=0 to t=1.
1. Average acceleration is defined as:
Average Acceleration=a(1)−a(0)1−0
2. Calculate a(1) and a(0):
a(1)=3(1)2+2(1)+1=3+2+1=6
a(0)=3(0)2+2(0)+1=1
3. Average acceleration:
Average Acceleration=6−11−0=5m/s2
Summary of Answers:
(i) v(t)=t3+t2+t+10
(ii) x(t)=t44+t33+t22+10t
(iii) Average Speed ≈11.08m/s
(iv) Average Acceleration =5m/s2
Acceleration of the particle is given by:
a(t)=3t2+2t+1
a=dvdt
This implies:
dv=adt
v(t)=∫adt=∫(3t2+2t+1)dt
v(t)=t3+t2+t+C
where C is the constant of integration.
v(0)=03+02+0+C=10
Thus, C=10.
v(t)=t3+t2+t+10
v=dxdt
This implies:
dx=vdt
x(t)=∫vdt=∫(t3+t2+t+10)dt
x(t)=t44+t33+t22+10t+D
where D is the constant of integration.
x(0)=0+0+0+0+D=0
Thus, D=0.
x(t)=t44+t33+t22+10t
Average Speed=Total DistanceTotal Time
x(1)=144+133+122+10(1)
x(1)=14+13+12+10
x(1)=312+412+612+10=1312+10=13312
Average Speed=133121=13312m/s≈11.08m/s
Average Acceleration=a(1)−a(0)1−0
a(1)=3(1)2+2(1)+1=3+2+1=6
a(0)=3(0)2+2(0)+1=1
Average Acceleration=6−11−0=5m/s2
(i) v(t)=t3+t2+t+10
(ii) x(t)=t44+t33+t22+10t
(iii) Average Speed ≈11.08m/s
(iv) Average Acceleration =5m/s2
Topper's Solved these Questions
- KINEMATICS OF A PARTICLE BOOK - VMC MODULES ENGLISHCHAPTER - KINEMATICS OF A PARTICLE EXERCISE - LEVEL 1
75 Videos
- KINEMATICS OF A PARTICLE BOOK - VMC MODULES ENGLISHCHAPTER - KINEMATICS OF A PARTICLE EXERCISE - LEVEL 2
65 Videos
- JEE MAIN REVISON TEST-23BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISON TEST-23EXERCISE - PHYSICS (SECTION 2)
1 Video
- LAWS OF MOTION BOOK - VMC MODULES ENGLISHCHAPTER - LAWS OF MOTION EXERCISE - IMPECCABLE
53 Videos
Similar Questions
Acceleration of a particle is given by
a=1/V
Where V is the velocity . Find
(i) Velocity of particle at time t, given that v = 10 m/s at t = 0.
(ii) Velocity of particle when its position is x, given that speed is 3 m/s at x = 0.
View SolutionPosition of a particle at any instant is given by x=3t2+1 , where x is in m and t in sec. Its average velocity in the time interval t=2 sec to t=3 sec will be :
View SolutionThe velocity of a particle is given by v=(2t2−4t+3)m/s where t is time in seconds. Find its acceleration at t=2 second.
View SolutionThe velocity of a particle is given by v=(4t2−4t+3)m/s where t is time in seconds. Find its acceleration at t=1 second.
View SolutionThe acceleration a in ms−2 of a particle is given by a=3t2+2t+2, where t is the time. If the particle starts out with a velocity v=2ms−1 at t=0, then find the velocity at the end of 2s.
View Solutionthe angular velocity omega of a particle varies with time t as ω=5t2+25rads. the angular acceleration of the particle att=1s is
View SolutionThe position of a particle is expressed as →r=(4t2ˆi+2tˆj) m, where t is time in second. Find the velocity o the particle at t = 3 s
View SolutionFigure shows x-t graph of a particle. Find the Time T such that the average velocity of the particle during the period 0 to T is Zero.
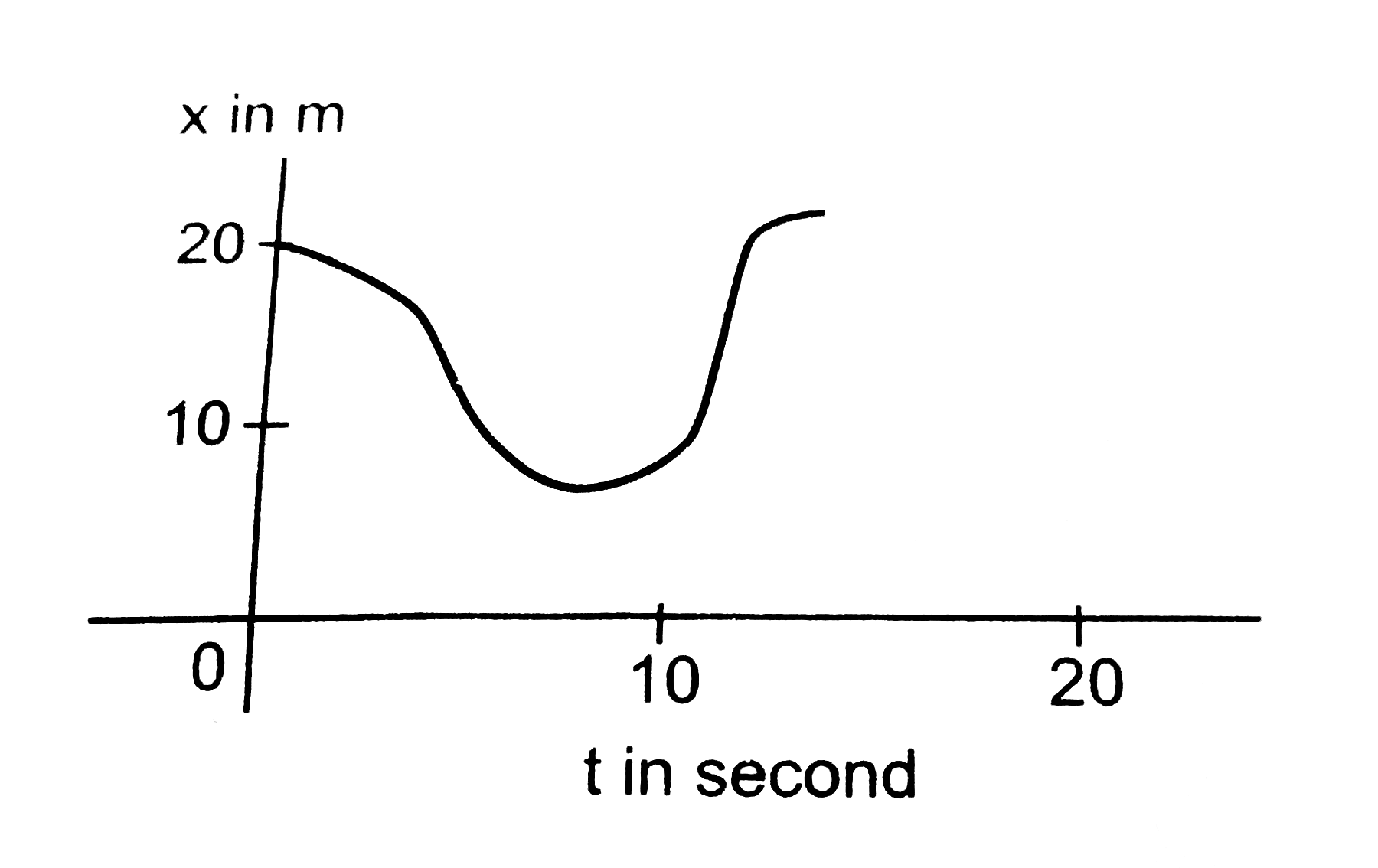
View SolutionFor a particle moving in a straight line, the displacement of the particle at time t is given by
S=t3−6t2+3t+7
What is the velocity of the particle when its acceleration is zero?
View SolutionFor a particle moving in a straight line, the displacement of the particle at time t is given by
S=t3−6t2+3t+7
What is the velocity of the particle when its acceleration is zero?
View Solution
