Two independent harmonic oscillators of equal mass are oscillating about the origin with angular frequencies ω1 and ω2 and have total energies E1 and E2 repsectively. The variations of their momenta p with positions x are shown in figures. If ab=n2 and aR=n, then the correc t equation(s) is (are) :

E1ω1=E2ω2
ω2ω1=n2
ω1ω2=n2
E1ω1=E2ω2
The correct Answer is:BD
x=Asin(ωt)
V=Acos(ωt)
At mean position
P1=mA1ω1=b,A1=a(from ellipse)
P2=mA2ω2=R,A2=R (From circle)
Also, ab=n2andaR=n⇒Rb=n , E1=P212m and E2=P222m
E1E2=(bR)2=1n2⇒mω21A21mω22A22=1n2 ⇒ω1ω2=1n2⇒E1ω1=E2ω2
Topper's Solved these Questions
- SIMPLE HARMONIC MOTION BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - 6-previous year question
56 Videos
- ROTATIONAL MOTION BOOK - VMC MODULES ENGLISHCHAPTER - ROTATIONAL MOTION EXERCISE - JEE Advanced (Archive) (True/False Type)
3 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE F
10 Videos
Similar Questions
Two bodies of different masses m1 and m2 have equal momenta. Their kinetic energies E1 and E2 are in the ratio
View SolutionTwo bodies of different masses m1 and m2 have equal momenta. Their kinetic energies E1 and E2 are in the ratio
View SolutionTwo bodies of different masses m1 and m2 have equal momenta. Their kinetic energies E1 and E2 are in the ratio
View SolutionIn forced oscillation of a particle the amplitude is maximum for a frequency ω1 of the force while the energy is maximum for a frequency ω2 of the force, then .
View SolutionA particle performing SHM with angular frequency ω=5000 radian/second and amplitude A=2 cm and mass of 1 kg. Find the total energy of oscillation.
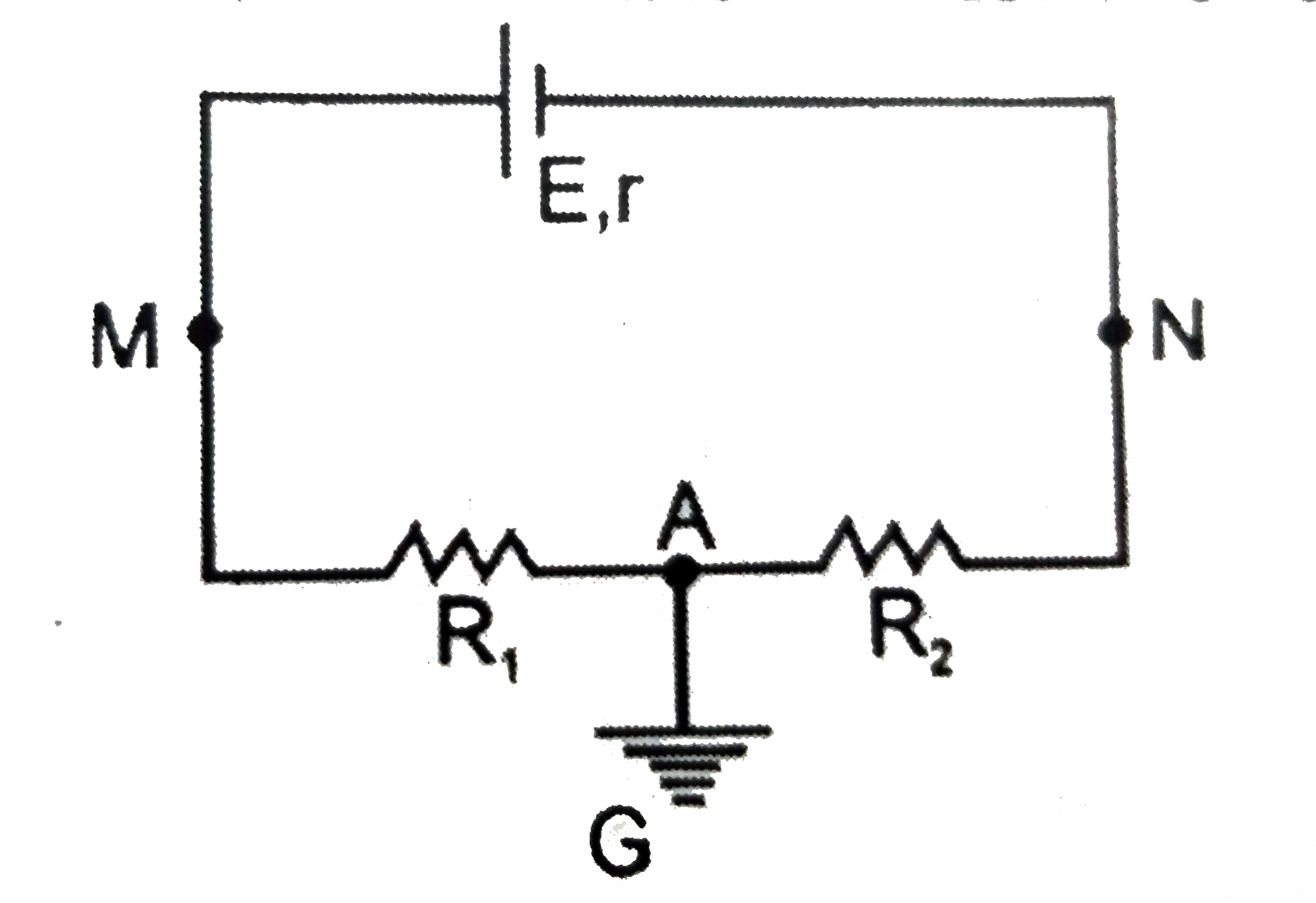
View SolutionIn the given figure, E=12V, R1=3Ω,R2=2Ω and r=1Ω. The choose the correct option/s
View SolutionTwo bodies with masses m1kgandm2kg have equal kinetic energies. If p1andP2 are their respective momenta, then p1/P2 is equal to :
View SolutionA particle of mass m=1kg excutes SHM about mean position O with angular frequency ω=1.0rad/s and total energy 2J. x is positive if measured towards right from O. At t=0, particle is at O and movel towards right.
View SolutionFind the total energy of oscillations if mass of the body is 100g
A=1m , ω= 2
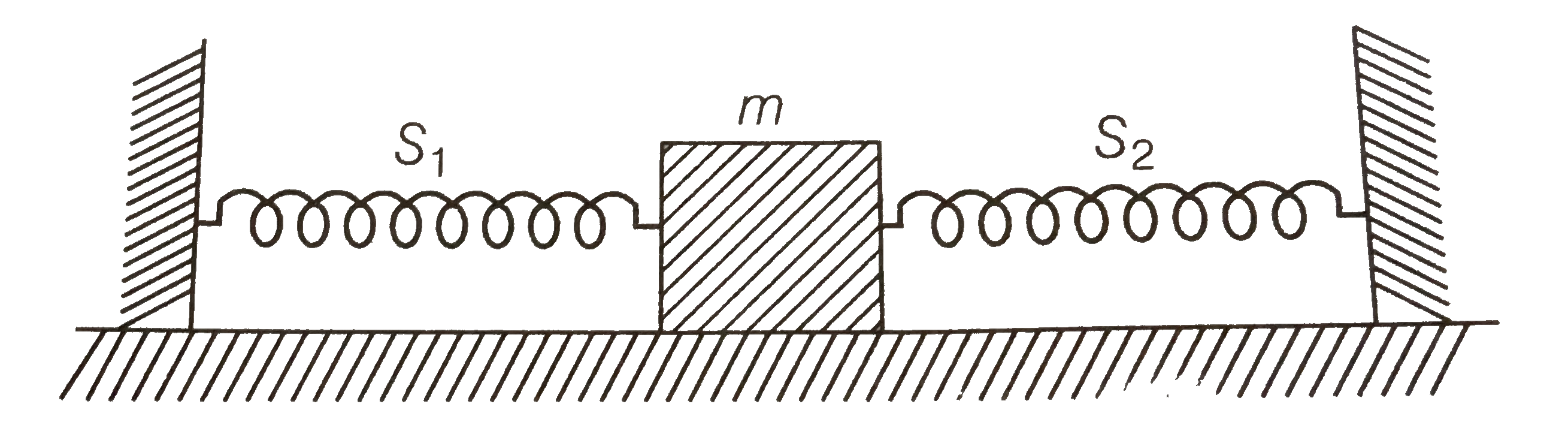
View SolutionWhen a mass m is connected individually to two springs S1 and S2, the oscillation frequencies are v1 and v2. If the same mass is attached to the two springs as shown in figure, the oscillation frequency would be
View Solution
