Question

When a particle is mass $m$ moves on the $x-$ axis in a potential of the from $V\left(x\right)=k{x}^2$, it performs simple harmonic motion. The corresponding thime periond is proportional to $\sqrt{\frac{m}{k}}$, as can be seen easily asing dimensional analysis. However, the motion of a pariticle can be periodic even when its potential enem increases on both sides $x=0$ in a way different from $k{x}^2$ and its total energy is such that the particel does not escape to infinity. consider a particle of mass $m$ moving onthe $x-$axis . Its potential energy is $V\left(x\right)=\omega (\alpha >0$) for $\left|x\right|$ near the origin and becomes a constant equal to $V_0$ for $\left|x\right|\ge X_0$ (see figure)

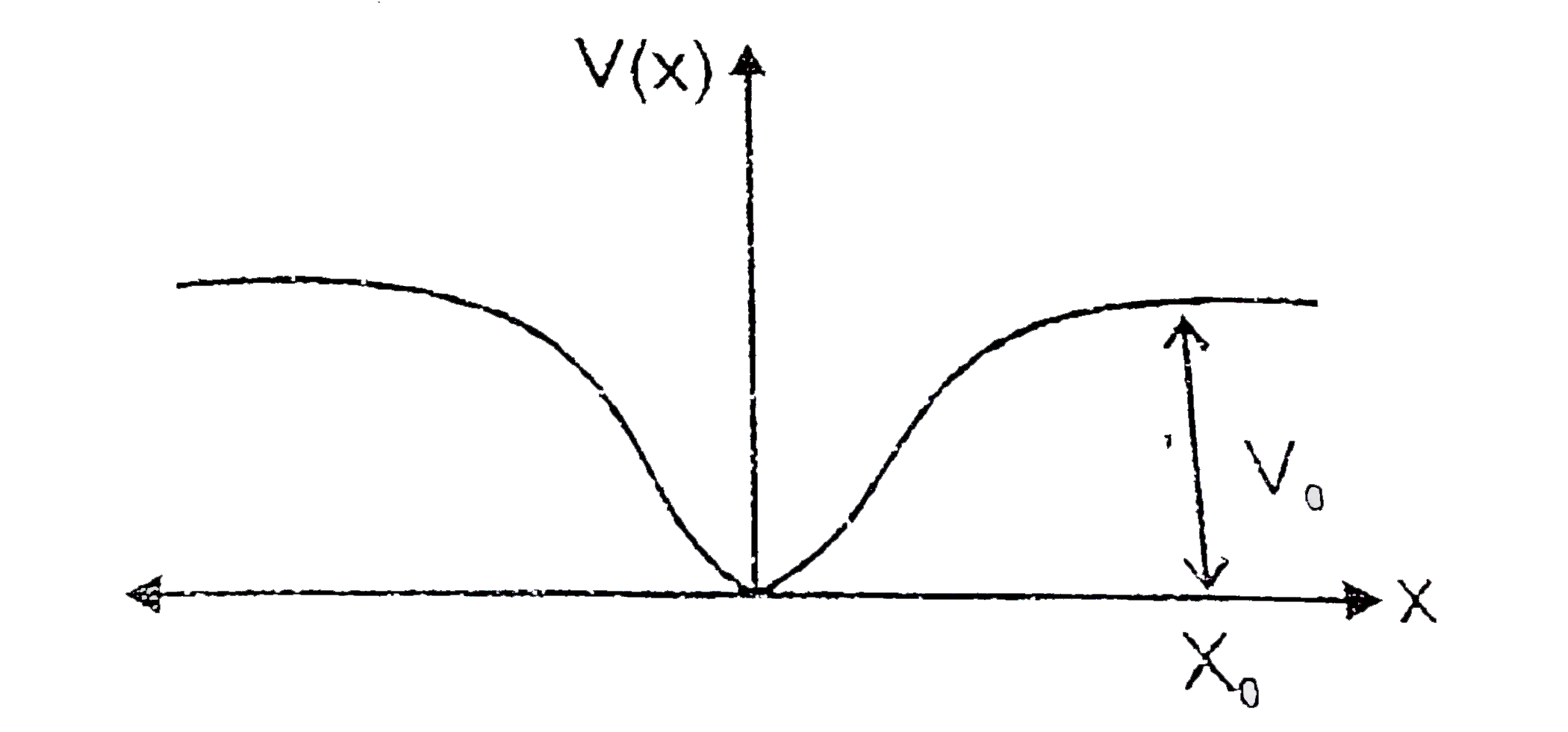
If the total energy of the particle is $E$, it will perform is periodic motion why if :

Options

A. `Elt0`
B. `Egt0`
C. `V_0gtEgt0`
D. `EgtV_0`

Answer

B

Explanation

Dimension `alpha` can be found as `[alpha]=ML^(-2)T^(-2)`
Only option (B) has dimension of time
Alternatively:
From conservation of energy: `(1)/(2)m((dx)/(dt))^(2)+ax^(4)=alphaA^(4)`, `((dx)/(dt))^(2)=(2a)/(m)(A^(4)-x^(4)) `
`overset(T//4)underset(0)intdt=sqrt((m)/(2a))overset(A)underset(0)int(dx)/(sqrt(A^(4)-x^(4)))`
implies `(T)/(4)=(1)/(A)sqrt((m)/(2a))overset(1)underset(0)int(dx)/(sqrt(1-u^(4)))` [Substitudex=Au]
implies `Tprop(1)/(A)sqrt((m)/(a))`