A child swinging on a swing in sitting position, stands up, then the time period of the swing will.
(a)increase
(b)decrease
(c)remain the same
(d)increase if the child is long and decrease if the child is short
The correct Answer is:B
To solve the problem of how the time period of a swing changes when a child standing up, we can follow these steps:
Step 1: Understand the time period of a pendulum
The time period T of a simple pendulum is given by the formula:
T=2π√Lg
where:
- T is the time period,
- L is the length of the pendulum,
- g is the acceleration due to gravity.
Step 2: Analyze the situation when the child is sitting
When the child is sitting on the swing, the effective length L is the distance from the pivot point (the top of the swing) to the center of mass of the child.
Step 3: Consider the change when the child stands up
When the child stands up, the center of mass of the child rises. This means that the effective length L of the pendulum decreases.
Step 4: Relate the change in length to the time period
Since T is proportional to the square root of L, if L decreases, T will also decrease. This can be expressed as:
- If L decreases, then T decreases.
Step 5: Conclusion
Thus, when the child stands up, the time period of the swing will decrease.
Final Answer
The time period of the swing will decrease when the child stands up.
The time period T of a simple pendulum is given by the formula:
T=2π√Lg
where:
- T is the time period,
- L is the length of the pendulum,
- g is the acceleration due to gravity.
When the child is sitting on the swing, the effective length L is the distance from the pivot point (the top of the swing) to the center of mass of the child.
When the child stands up, the center of mass of the child rises. This means that the effective length L of the pendulum decreases.
Since T is proportional to the square root of L, if L decreases, T will also decrease. This can be expressed as:
- If L decreases, then T decreases.
Thus, when the child stands up, the time period of the swing will decrease.
The time period of the swing will decrease when the child stands up.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTION BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - 7-previous year question
46 Videos
- SIMPLE HARMONIC MOTION BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - LEVEL (2)
40 Videos
- ROTATIONAL MOTION BOOK - VMC MODULES ENGLISHCHAPTER - ROTATIONAL MOTION EXERCISE - JEE Advanced (Archive) (True/False Type)
3 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE F
10 Videos
Similar Questions
When a boy is playing on a swing in the sitting position , the time period of oscillations of the swing is T. If the boy stands up , the time period of oscillation of the spring will be
View SolutionAssertion : When a girl sitting on a swing stands up, the periodic time of the swing will increase.
Reason : In standing position of the girl, the length of swing will increase.
View SolutionA boy is swinging in a swing. If he stands , the time period will
View SolutionA ball of mass m is fastened to a string. The ball swings in a vertical circle of radius R with the other end of the string held fixed. Neglecting the air resistance, the difference between the string's tension at the bottom of the circle and at the quarter of the cirlce is
View SolutionA boy sitting on a swing which is moving to an angle of 30∘ from the vertical is blowing a whistle which has a frequency of 100 Hz. The whistle is at 2.0 m from the point of support of the swing. A girl stands in front of the swing. The maximum frequency she will hear is nearly 10x Hz (velocity of sound 330 m/s,g= 9.8m/s2). Find x
View SolutionA 50 kg girl is swinging on a swing from rest. Then, the power delivered when moving with a velocity of 2ms−1 upwards in a direction making an angle 60∘ with the vertical is
View SolutionA girl is swinging in a swing with a constant angular amplitude 60∘. Force constnat of the cushion on which she sits is K. Strings of swing are massless and extensible. Assuming highest point as Datum, match the entries in column I and II
View SolutionA person of mass M is setting on a swing of length L and swinging with an angular amplitude θ0 if the person stands up when the swing passes through its lowest point, the work done by him, assuming that his centre of mass moves by a distance l(<<L)
View SolutionA ball is held at rest in position A by two light cords. The horizontal cord is now cut and the ball swings to the position B. What is the ratio of the tension in the cord in position B to that in position A?
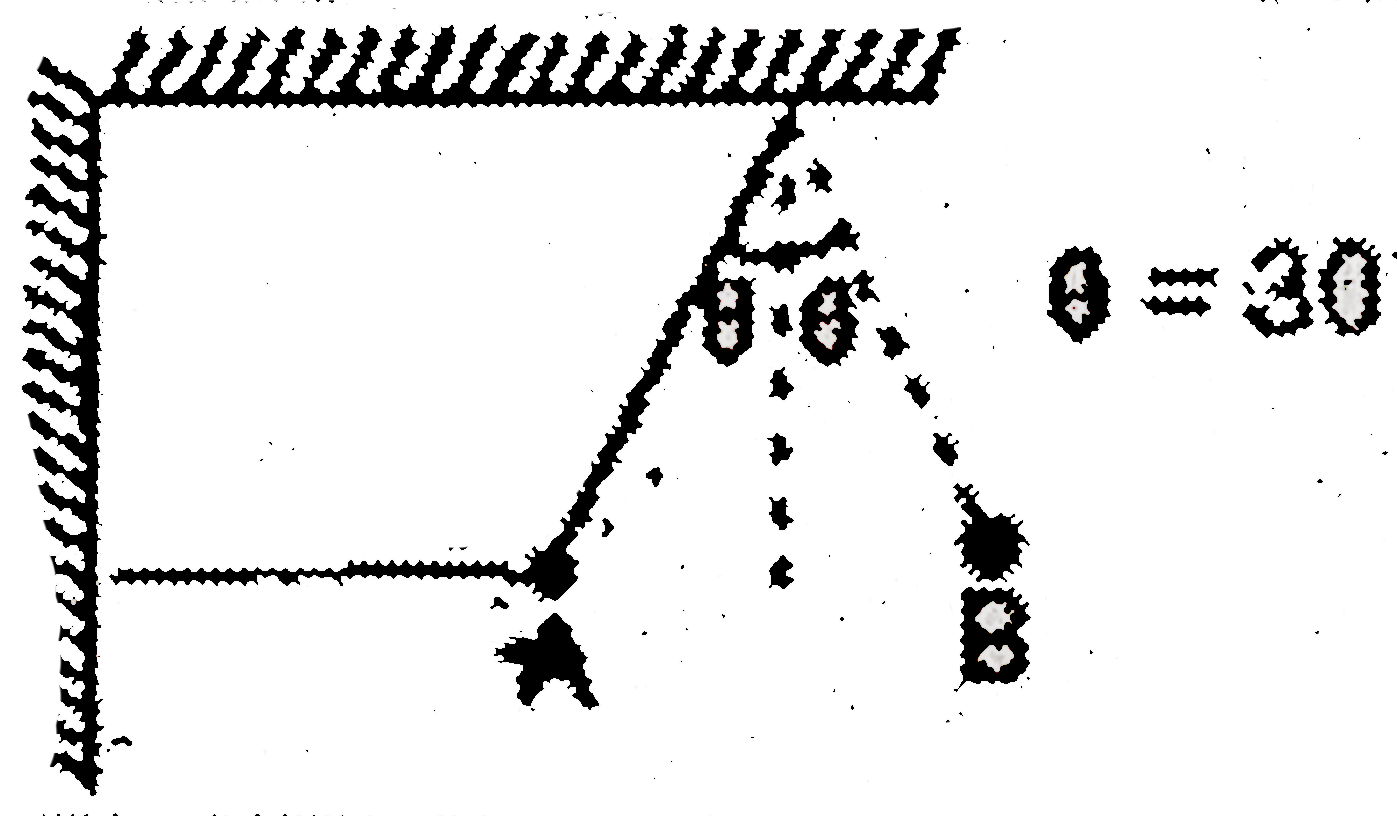
View SolutionA small bucket of mass M is attached to a long inextensible cord of length L. The bucket is released from rest when the cord is in a horizontal position. In its lowest position the bucket scoops up m of water, what is the height of the swing above the lowest position?
View Solution
