A system undergoes a cyclic process in which it absorbs Q1 heat and gives out Q2 heat. The efficiency of the process is η and work done is W. Select correct statement:
W=Q1−Q2
η=WQ1
η=Q2Q1
η=1−Q2Q1
The correct Answer is:A, B, D
To solve the problem, we need to analyze the cyclic process of the system and derive the relationships between the heat absorbed, heat given out, work done, and efficiency.
1. Understanding the Efficiency of the Process:
The efficiency (η) of a heat engine is defined as the ratio of the work done (W) to the heat absorbed (Q1). Mathematically, this can be expressed as:
η=WQ1
2. Relating Work Done to Heat Absorbed and Heat Given Out:
In a cyclic process, the work done by the system can also be expressed in terms of the heat absorbed (Q1) and the heat rejected (Q2). The work done (W) is given by the difference between the heat absorbed and the heat rejected:
W=Q1−Q2
3. Substituting Work Done into the Efficiency Formula:
We can substitute the expression for work done into the efficiency formula:
η=Q1−Q2Q1
4. Simplifying the Efficiency Expression:
This can be further simplified:
η=1−Q2Q1
5. Conclusion:
Based on the above derivations, we can conclude the following:
- The efficiency of the process is given by η=WQ1.
- The work done can be expressed as W=Q1−Q2.
- The efficiency can also be expressed as η=1−Q2Q1.
Correct Statements:
From the analysis, we find that:
- The efficiency is correctly defined.
- The work done is correctly expressed.
- The alternative expression for efficiency is also correct.
Thus, the correct statements are A, B, and D.
The efficiency (η) of a heat engine is defined as the ratio of the work done (W) to the heat absorbed (Q1). Mathematically, this can be expressed as:
η=WQ1
In a cyclic process, the work done by the system can also be expressed in terms of the heat absorbed (Q1) and the heat rejected (Q2). The work done (W) is given by the difference between the heat absorbed and the heat rejected:
W=Q1−Q2
We can substitute the expression for work done into the efficiency formula:
η=Q1−Q2Q1
This can be further simplified:
η=1−Q2Q1
Based on the above derivations, we can conclude the following:
- The efficiency of the process is given by η=WQ1.
- The work done can be expressed as W=Q1−Q2.
- The efficiency can also be expressed as η=1−Q2Q1.
From the analysis, we find that:
- The efficiency is correctly defined.
- The work done is correctly expressed.
- The alternative expression for efficiency is also correct.
Topper's Solved these Questions
- GASEOUS STATE & THERMODYNAMICSBook:VMC MODULES ENGLISHChapter:GASEOUS STATE & THERMODYNAMICSExercise:JEE MAIN (ARCHIVE )
Explore 81 Videos
- GASEOUS STATE & THERMODYNAMICSBook:VMC MODULES ENGLISHChapter:GASEOUS STATE & THERMODYNAMICSExercise:JEE ADVANCED (ARCHIVE )
Explore 111 Videos
- GASEOUS STATE & THERMODYNAMICSBook:VMC MODULES ENGLISHChapter:GASEOUS STATE & THERMODYNAMICSExercise:Level - 1
Explore 75 Videos
- ENERGY & MOMENTUMBook:VMC MODULES ENGLISHChapter:ENERGY & MOMENTUMExercise:JEE ADVANCE (ARCHIVE) - TRUE/FALSE TYPE
Explore 1 Video
- GRAVITATIONBook:VMC MODULES ENGLISHChapter:GRAVITATIONExercise:JEE Advance (Archive) TRUE/FALSE
Explore 1 Video
Similar Questions
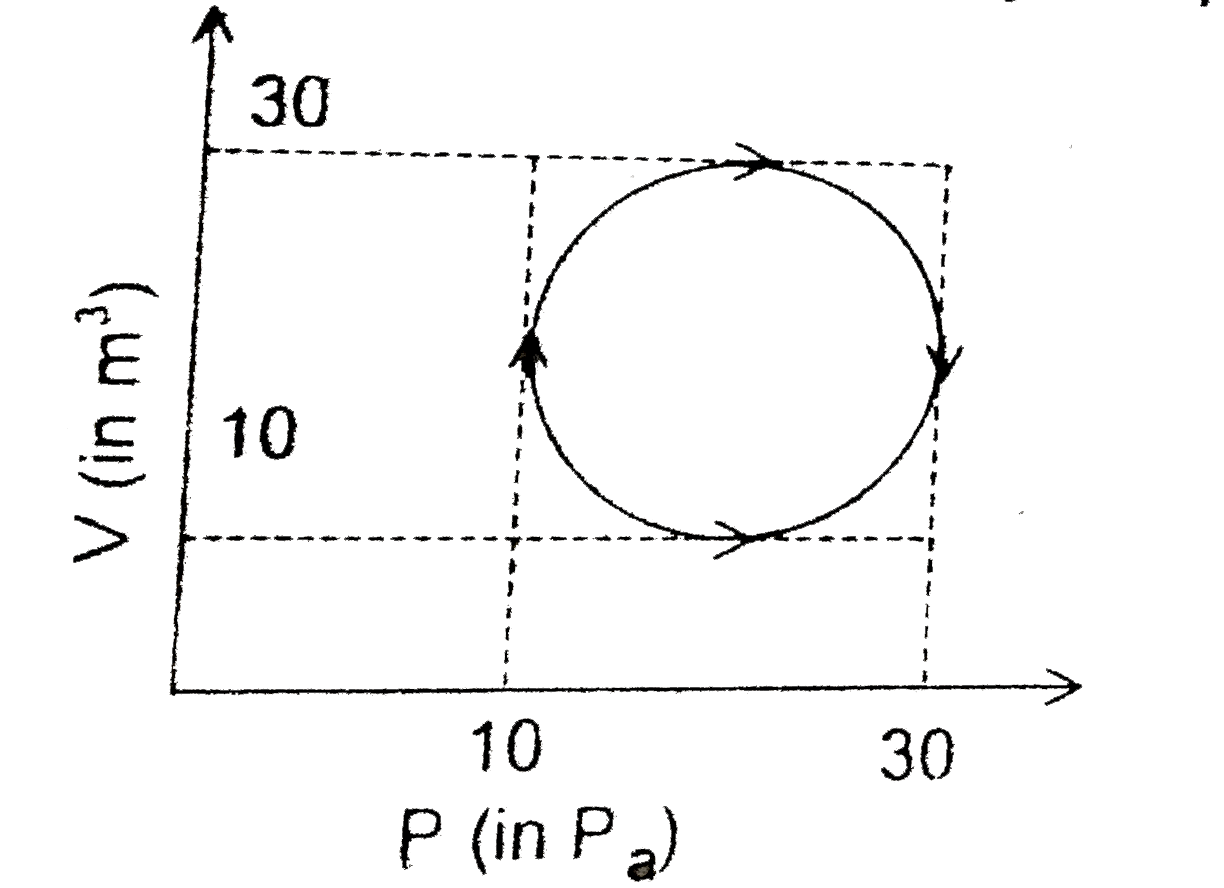
A system works under cyclic process as follow
Heat absorbed during the process is
View SolutionA system works in a cyclic process. It absorbs 20 calories of heat and rejects 60J of heat during the process. The magnitude of work done (J) is [1calorie=4.2J]:
View SolutionIn a cyclic process, work done by the system is
View SolutionDuring a process, a system abdorbs 710J of heat and does work. The change in ΔU for the process is 460J. What is the work done by the system?
View SolutionA system undergoes a process which absorbed 0.5KJ of heat and undergoing an expansion againt external pressure of 1atm, during the process change in internal energy is 300J. Then predict the change in volume (lit)
View SolutionA system undergoes two cyclic process 1 and 2. Process 1 is reversible and process 2 is irreversible. The correct statement relating to the two processes is
View SolutionThe internal energy of a system remains constant when it undergoes
(i) a cyclic process
(ii) an isothermal process
(iii) an adiabatic process
(iv) any process in which the heat given out by the system equal to the work done on the system
View SolutionA : Heat absorbed in a cyclic process is zero.
R : Work done in a cyclic process is zero.
View Solution
An ideal gas undergoes a cyclic process, in which one process is isochoric, one process is isothermal and one process is adiabatic. During the isothermal process, 40 J heat is released by the gas, and during the isochoric process, 80 J heat is absorbed by the gas. if work done by the gas during adiabatic process is W1 and during isothermal process is W2 then the magnitude of W1W2 will be equal to:
View SolutionIn a certain process, 400J of work is done on a system which gives off 200J of heat. What is ΔU for the process?
View Solution