The speed of a motor increases uniformly from 600 rpm to 1200 rpm in 10 second. How many revolutions does it make in this period
150
250
300
325
The correct Answer is:A
To solve the problem of how many revolutions a motor makes as its speed increases uniformly from 600 rpm to 1200 rpm in 10 seconds, we can follow these steps:
1. Convert RPM to RPS:
- The initial speed (ω_initial) is 600 revolutions per minute (rpm).
- The final speed (ω_final) is 1200 revolutions per minute (rpm).
- To work in seconds, we convert these values to revolutions per second (rps):
- ω_initial = 600 rpm ÷ 60 = 10 rps
- ω_final = 1200 rpm ÷ 60 = 20 rps
2. Calculate the Average Speed:
- The average speed (ω_avg) during the time interval can be calculated as:
ωavg=ωinitial+ωfinal2
- Substituting the values:
ωavg=10+202=302=15 rps
3. Calculate the Total Time:
- The total time (t) during which the speed increases is given as 10 seconds.
4. Calculate the Total Revolutions:
- The total number of revolutions (N) made during this time can be calculated using the formula:
N=ωavg×t
- Substituting the values:
N=15 rps×10 s=150 revolutions
Final Answer:
The motor makes 150 revolutions during the 10 seconds of acceleration from 600 rpm to 1200 rpm.
---
- The initial speed (ω_initial) is 600 revolutions per minute (rpm).
- The final speed (ω_final) is 1200 revolutions per minute (rpm).
- To work in seconds, we convert these values to revolutions per second (rps):
- ω_initial = 600 rpm ÷ 60 = 10 rps
- ω_final = 1200 rpm ÷ 60 = 20 rps
- The average speed (ω_avg) during the time interval can be calculated as:
ωavg=ωinitial+ωfinal2
- Substituting the values:
ωavg=10+202=302=15 rps
- The total time (t) during which the speed increases is given as 10 seconds.
- The total number of revolutions (N) made during this time can be calculated using the formula:
N=ωavg×t
- Substituting the values:
N=15 rps×10 s=150 revolutions
The motor makes 150 revolutions during the 10 seconds of acceleration from 600 rpm to 1200 rpm.
Topper's Solved these Questions
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - JEE Main (Archive)
19 Videos
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - JEE Advanced (Archive)
19 Videos
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - Long Answer Type
5 Videos
- Motion in Straight Line BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - IN-CHAPTER EXERCISE-J
10 Videos
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - IN-CHAPTER EXERCISE-K
10 Videos
Similar Questions
The speed of a motor increases from 1200 rpm to 1800 rpm in 20 S. How many revolutions does it make in this period of time?
View SolutionThe angular speed of a motor wheel is increased from 600rpm to 1200rpm in 4 seconds. Calculate how many revolutions does the engine make during this time.
View Solution
Knowledge Check
The angular speed of a motor wheel is increased from 1200 rpm to 3120 rpm in 16 seconds. The angular acceleration of the motor wheel is a) 2 π rads−2 b) 4 π rads−2 c) 6 π rads−2 d) 8 π rads−2
A2πrads−2B4πrads−2C6πrads−2D8πrads−2
The angular speed of a motor wheel is increased from 600rpm to 1200rpm in 4 seconds. Calculate how many revolutions does the engine make during this time.
View SolutionA flywheel rotates with a uniform angular acceleration. Its angular velocity increases from 20πrad/s to 40πrad/s in 10 seconds. How many rotations did it make in this period ?
View SolutionThe speed of a motor decreases from 1200 rpm to 600 rpm in 20s. The total number of rotations it makes before coming to rest is
View SolutionThe angular velocity of a motor wheel changes from 180 rpm to 300 rpm in 4 seconds. Calculate the no. of revolutions does the engine make during this time.
View SolutionThe angular velocity of a motor wheel changes from 180 rpm to 300 rpm in 4 seconds. Calculate the no. of revolutions does the engine make during this time.
View SolutionThe angular frequency of a fan increases uniformly from 30 rpm to 60 rpm in π second. A dust particle is present at a distance of 20 cm from axis of rotation. The tangential acceleration of the particle is :
View SolutionThe speed of a car increases uniformly from zero to 10ms−1 in 2s and then remains constant (figure)
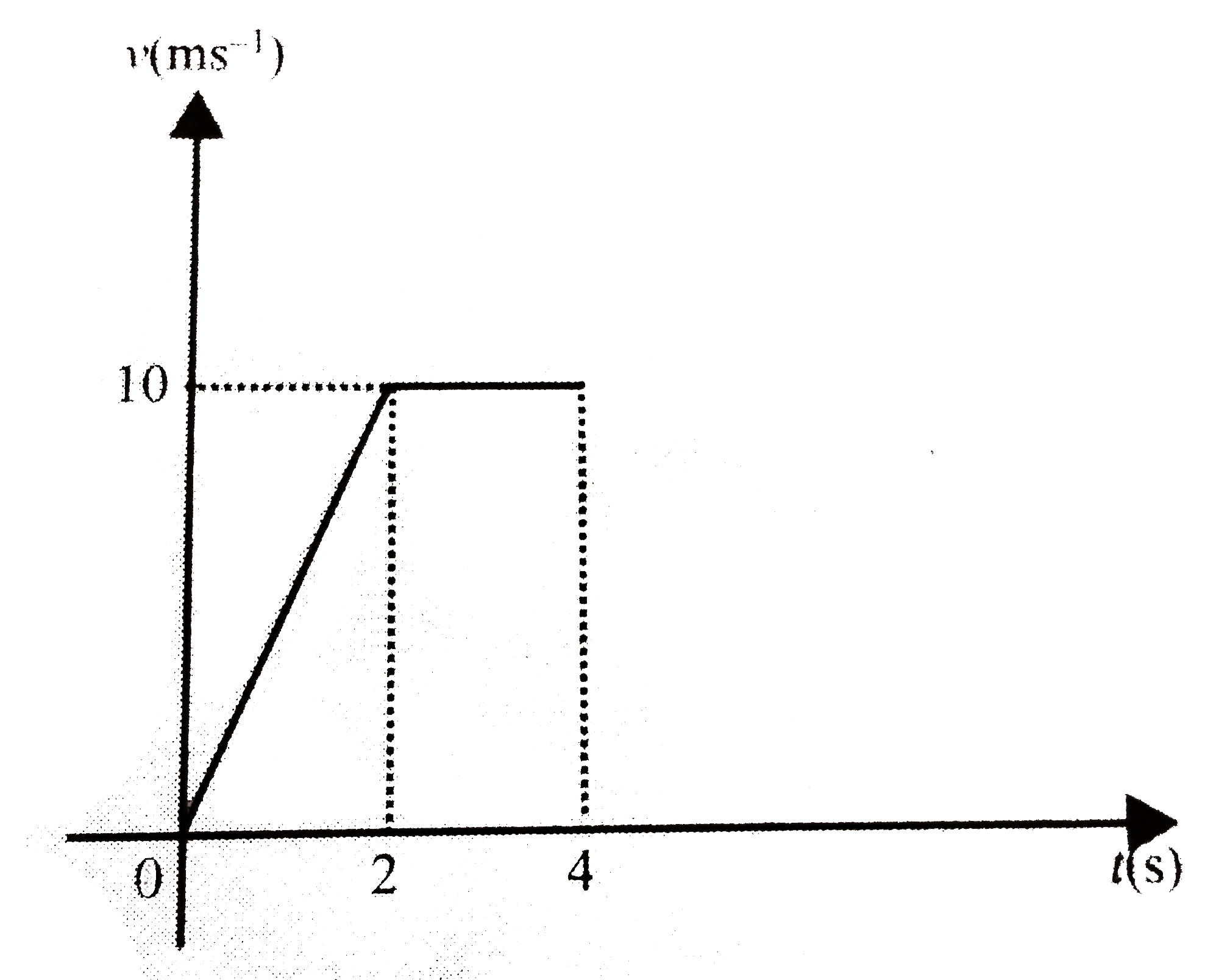
a. Find the distance travelled by the car in the first two seconds.
b. Find the distance travelled by the car in the next two seconds.
c. Find the total distance travelled in 4s.
View Solution
