A shpere S1 of radius r1 encloses a charge Q, if there is another concentric sphere S2 of the radius r2(r1>r1) and there are no additional charges between S1andS2. Find the ratio of electric flux through S1andS2Solution in Odia
∅1 ÷ O2 = Qε0 ÷ Qε0 = 1 : 1
Similar Questions
A conducting sphere S1 of radius r1 is connected by a conducting wire to another conducting sphere S2 of radius r2, where r1=3cmandr2=2 cm. Before they are connected, S1 carries charge of 10 units. The electric potential at the point which is at a distance 4 cm from the centre of S1 and a distance 3 cm from the centre of S2 is
View Solutionदो साबुन के बुलबुले जिनकी त्रिज्या r1 तथा r2 क्रमशः 4 सेमी तथा 5 सेमी के बराबर है जो एक दूसरे को उभयनिष्ठ पृष्ठ S1S2 (चित्र में दिखाए अनुसार) पर स्पर्श करते है तो इसकी त्रिज्या होगी।
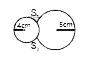
View SolutionA sphere S1 of radius r1 encloses a total charge Q. If there is another concentric sphere S2 of radius r2(>r1) and there be no additional charges between S1andS2 find the ratio of electric flux through S1andS2,
View SolutionConsider two charged metallic spheres S1, and S2, of radii R1, and R2 respectively. The electric fields E1, (on S1,) and E2, (on S2) their surfaces are such that E1/E2=R1/R2. Then the ratio V1(on S1)/V2 (on S2) of the electrostatic potential on each sphere is
View SolutionA sphere of radius r, encloses a net charge Q. If there is another concentric sphere S2 of radius r2(r2>r1) enclosing charge 20, find the ratio of the electric flux through S1 and S2. How will the electric flux through sphere S1 change, if a medium of dielectric constant k is introduced in the space inside S2 in place of air?
View SolutionS1,S2,S3 are three concentric spherical shells, as shown in figure. S1 and S2 have charge 4Q and 8Q respectively, whereas S3 is uncharged. Find the ratio of the flux passing through S1 to that through S3
View SolutionConsider two charged metallic spheres S_(1) and S_(2) of radii 3R and R, respectively. The electric potential V_(1) (on S_(1) ) and (on S_(2)) on their surfaces are such that (V_(1))/(V_(2)) = (4)/(1) . Then the ratio E_(1)(on S_(1)) / E_(2) (on S_(2)) of the electric fields on their surfaces is:
View Solution
