A sphere of radius r, encloses a net charge Q. If there is another concentric sphere S2 of radius r2(r2>r1) enclosing charge 20, find the ratio of the electric flux through S1 and S2. How will the electric flux through sphere S1 change, if a medium of dielectric constant k is introduced in the space inside S2 in place of air?
To solve the problem, we will follow these steps:
Step 1: Understand the setup
We have two concentric spheres:
- Sphere S1 with radius r1 enclosing a charge Q.
- Sphere S2 with radius r2 (where r2>r1) enclosing a charge 20Q.
Step 2: Use Gauss's Law
According to Gauss's Law, the electric flux Φ through a closed surface is given by:
Φ=Qencϵ0
where Qenc is the charge enclosed by the surface and ϵ0 is the permittivity of free space.
Step 3: Calculate the electric flux through S1
For sphere S1:
Φ1=Qϵ0
Step 4: Calculate the electric flux through S2
For sphere S2:
Φ2=20Qϵ0
Step 5: Find the ratio of the electric fluxes
To find the ratio of the electric flux through S1 and S2:
Ratio=Φ1Φ2=Qϵ020Qϵ0=Q20Q=120
Step 6: Effect of introducing a dielectric medium
When a dielectric medium with dielectric constant k is introduced in the space inside S2, the electric flux through S1 does not change. This is because the charge Q enclosed by S1 remains the same, and Gauss's Law states that the electric flux depends only on the enclosed charge, not on the medium outside.
Final Answer
The ratio of the electric flux through S1 and S2 is 120, and the electric flux through sphere S1 remains unchanged when a dielectric medium is introduced.
---
We have two concentric spheres:
- Sphere S1 with radius r1 enclosing a charge Q.
- Sphere S2 with radius r2 (where r2>r1) enclosing a charge 20Q.
According to Gauss's Law, the electric flux Φ through a closed surface is given by:
Φ=Qencϵ0
where Qenc is the charge enclosed by the surface and ϵ0 is the permittivity of free space.
For sphere S1:
Φ1=Qϵ0
For sphere S2:
Φ2=20Qϵ0
To find the ratio of the electric flux through S1 and S2:
Ratio=Φ1Φ2=Qϵ020Qϵ0=Q20Q=120
When a dielectric medium with dielectric constant k is introduced in the space inside S2, the electric flux through S1 does not change. This is because the charge Q enclosed by S1 remains the same, and Gauss's Law states that the electric flux depends only on the enclosed charge, not on the medium outside.
The ratio of the electric flux through S1 and S2 is 120, and the electric flux through sphere S1 remains unchanged when a dielectric medium is introduced.
Similar Questions
S1 and S2 are two hollow concentric spheres enclosing charges Q and 2Q, respectively, as shown in figure.
i. What is the ratio of the electric flux through S1 and S2?
ii. How will the electric flux through sphere S1 change if a medium of dielectric constant 5 is introduced in the space inside S1 in place of air.
 .
.
View SolutionA sphere S1 of radius r1 encloses a total charge Q. If there is another concentric sphere S2 of radius r2(>r1) and there be no additional charges between S1andS2 find the ratio of electric flux through S1andS2,
View SolutionS1andS2 are two hollow concentric spheres enclosing charge Q and 2Q, respectively, as shown in figure. What is the ratio of the electric flux leaving through the surface of S1andS2?

View SolutionA conducting sphere of radius R and carrying a charge Q is joined to an uncharged conducting sphere of radius 2R. The charge flowing between them will be
View SolutionTwo charged spheres of radii R1andR2 having equal surface charge density. The ratio of their potential is
View SolutionA conducting sphere of radius R, carrying charge Q, lies inside uncharged conducting shell of radius 2R. If they are joined by a metal wire,
View SolutionA sphere of radius R and charge Q is placed inside an imaginary sphere of radius 2R whose centre coincides with the given sphere. The flux related to imaginary sphere is:
View SolutionS1,S2,S3 are three concentric spherical shells, as shown in figure. S1 and S2 have charge 4Q and 8Q respectively, whereas S3 is uncharged. Find the ratio of the flux passing through S1 to that through S3
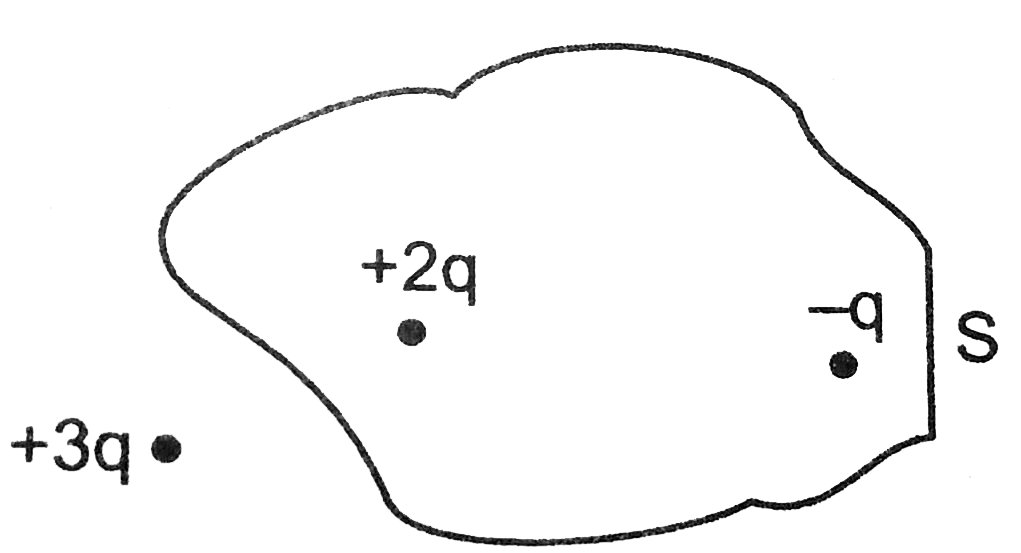
View SolutionFig shows three point charges +2q,−qand+3q, What is the electric flux due to this configuration through the surface S ?
View SolutionTwo concentric, thin metallic spheres of radii R1andR2(R1>R2) charges Q1andQ2 respectively Then the potential at radius r between R1andR2 will be (k=1/4π∈)
View Solution