A sperical shell of radius R with uniform charge q is expanded to a radius 2R. Find the work perfomed by the electric forces and external agent against electric forces in this process.
To solve the problem of finding the work performed by the electric forces and the external agent when a spherical shell of radius R with uniform charge q is expanded to a radius of 2R, we can follow these steps:
Step 1: Understand the Initial and Final States
- We have a spherical shell with a uniform charge q at an initial radius R.
- The shell is expanded to a final radius of 2R.
Step 2: Calculate the Initial Potential Energy Uinitial
- The potential energy U of a uniformly charged spherical shell is given by the formula:
U=q28πϵ0R
- For the initial radius R:
Uinitial=q28πϵ0R
Step 3: Calculate the Final Potential Energy Ufinal
- For the final radius 2R:
Ufinal=q28πϵ0(2R)=q216πϵ0R
Step 4: Calculate the Work Done by Electric Forces
- The work done by the electric forces Welectric is equal to the change in potential energy:
Welectric=Uinitial−Ufinal
- Substituting the values:
Welectric=q28πϵ0R−q216πϵ0R
- To simplify, find a common denominator:
Welectric=2q216πϵ0R−q216πϵ0R=q216πϵ0R
Step 5: Calculate the Work Done by the External Agent
- The work done by the external agent Wexternal is equal to the change in potential energy but in the opposite direction:
Wexternal=Ufinal−Uinitial
- Substituting the values:
Wexternal=q216πϵ0R−q28πϵ0R
- Again, finding a common denominator:
Wexternal=q216πϵ0R−2q216πϵ0R=−q216πϵ0R
Final Results
- The work done by the electric forces is:
Welectric=q216πϵ0R
- The work done by the external agent is:
Wexternal=−q216πϵ0R
- We have a spherical shell with a uniform charge q at an initial radius R.
- The shell is expanded to a final radius of 2R.
- The potential energy U of a uniformly charged spherical shell is given by the formula:
U=q28πϵ0R
- For the initial radius R:
Uinitial=q28πϵ0R
- For the final radius 2R:
Ufinal=q28πϵ0(2R)=q216πϵ0R
- The work done by the electric forces Welectric is equal to the change in potential energy:
Welectric=Uinitial−Ufinal
- Substituting the values:
Welectric=q28πϵ0R−q216πϵ0R
- To simplify, find a common denominator:
Welectric=2q216πϵ0R−q216πϵ0R=q216πϵ0R
- The work done by the external agent Wexternal is equal to the change in potential energy but in the opposite direction:
Wexternal=Ufinal−Uinitial
- Substituting the values:
Wexternal=q216πϵ0R−q28πϵ0R
- Again, finding a common denominator:
Wexternal=q216πϵ0R−2q216πϵ0R=−q216πϵ0R
- The work done by the electric forces is:
Welectric=q216πϵ0R
- The work done by the external agent is:
Wexternal=−q216πϵ0R
Topper's Solved these Questions
- ELECTROSTATICSBook:RESONANCE ENGLISHChapter:ELECTROSTATICSExercise:Problems
Explore 22 Videos
- ELECTROSTATICSBook:RESONANCE ENGLISHChapter:ELECTROSTATICSExercise:Exercise-1 Section (A)
Explore 9 Videos
- ELECTROMAGNETIC INDUCTIONBook:RESONANCE ENGLISHChapter:ELECTROMAGNETIC INDUCTIONExercise:A.l.P
Explore 19 Videos
- EXPERIMENTAL PHYSICSBook:RESONANCE ENGLISHChapter:EXPERIMENTAL PHYSICSExercise:PART -II
Explore 10 Videos
Similar Questions
A spherical shell of radius R1 with uniform charge q is expanded to a radius R2. Find the work performed by the electric forces in this process.
View SolutionA spherical shell of radius R1 with a uniform charge q has a point charge q0 at its center. Find the work performed by the electric forces during the shell expansion from radius R1 to radius R2.
View SolutionA spherical shell of radius R has a uniformly distributed charge ,then electric field varies as
View SolutionA sperical ballon od radius R charged uniformly on its surface with surface density σ. Find work done against electric forces in expanding it upto radius 2R.
View SolutionA spherical shell of radius R carries a uniformly distributed charge q. The electrical forces arising cause the ex-pansion of the shell. Find the mechanical stress in the shell.
View Solutionn charged drops, each of radius r and charge q, coalesce to from a big drop of radius R and charge Q. If V is the electric potential and E is the electric field at the surface of a drop, then.
View SolutionA thin spherical conducting shell of radius R has a charge q. Another charge Q is placed at the centre of the shell. The electrostatic potential at a point P a distance R2 from the centre of the shell is
View SolutionA thin spherical conducting shell of radius R has a charge q. Another charge Q is placed at the centre of the shell. The electrostatic potential at a point P a distance R/2 from the centre of the shell is
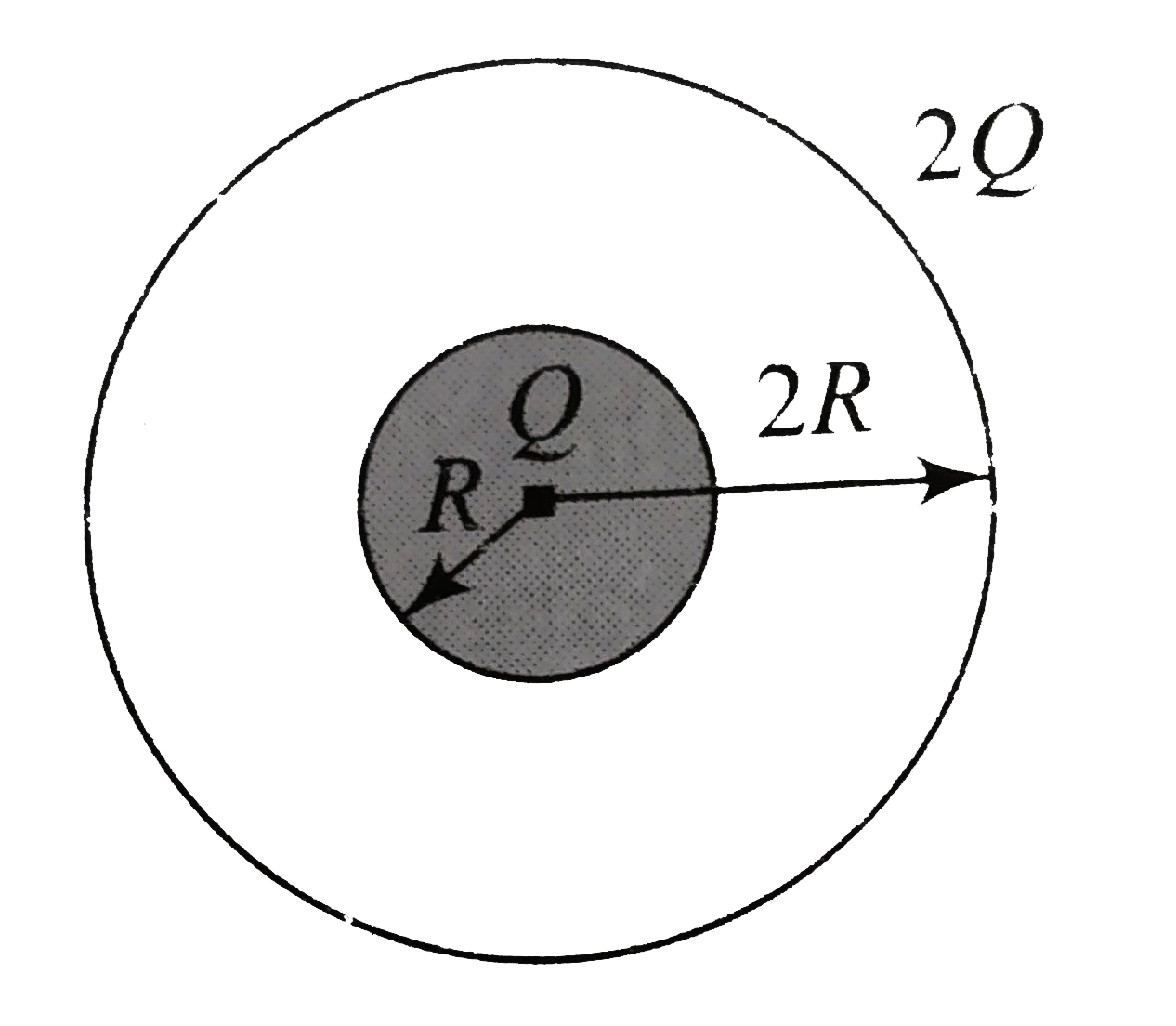
View SolutionA charge Q is uniformly distributed in a dielectric sphere of radius R (having dielectric constant unity). This dielectric sphere is enclosed by a concentric spherical shell of radius 2R and having uniformly distributed charge 2Q. Which of the following graph correctly represents variation of electric field with distance r from the common centre?
View SolutionConisder a thin spherical shell of radius R with centre at the origin, carrying uniform poistive surface charge denisty. The variation of the magnitude of the electric field ∣∣→E(r)∣∣ and the electric potential V(r) with the distance r from the centre, is best represented by which graph?
View Solution