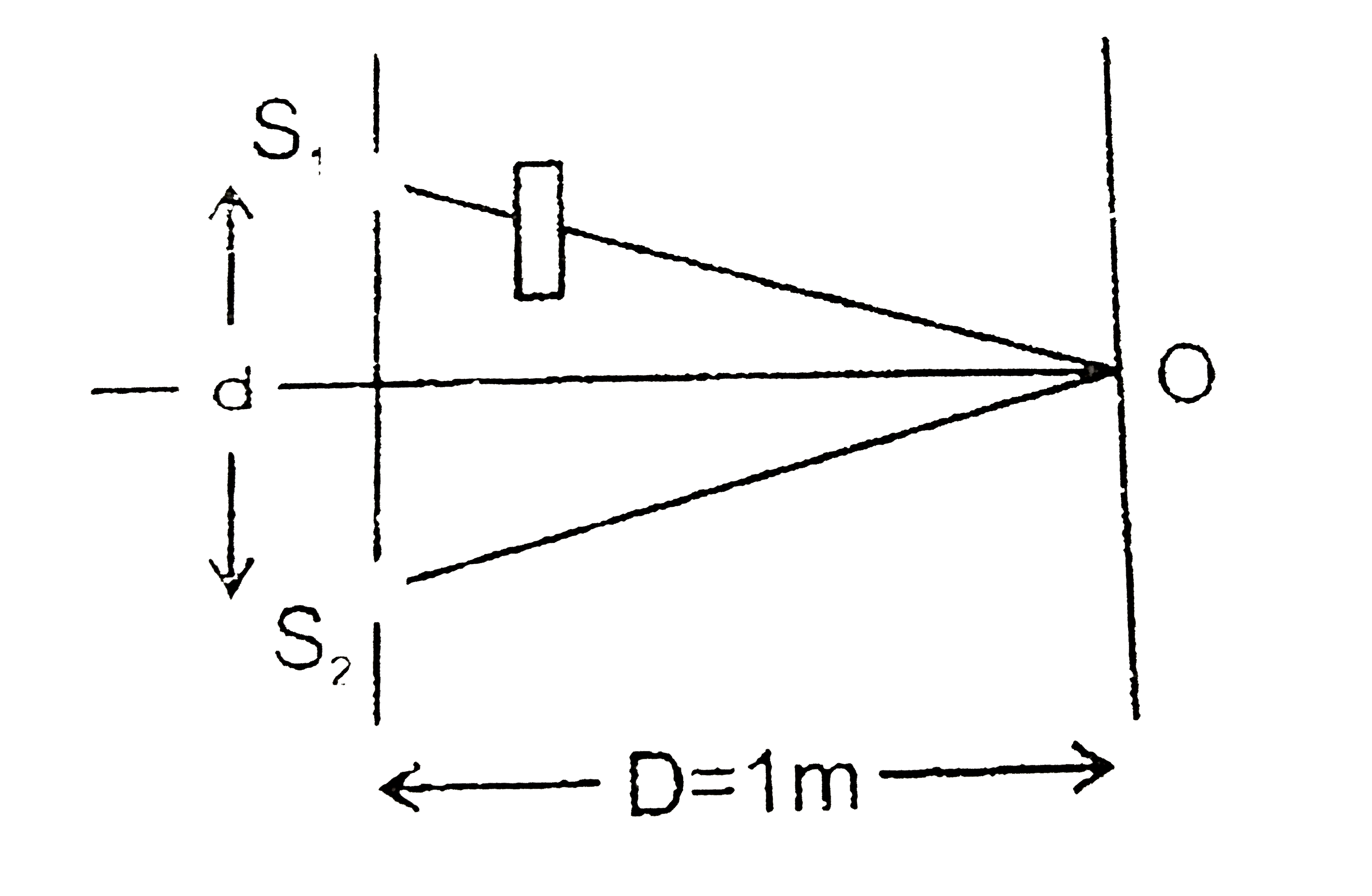
A monochromatic light of λ=500nm is incident on two identical slits separated by a distance of 5×10−4m. The interference pattern is seen on a screen placed at a distance of 1m from the plane of slits. A thin glass plate of thickness 1.5×10−6m and refractive index μ=1.5 is placed between one of the slits and the screen. Find the intensity at the center of the screen if the intensity is I0 in the absence of the plate. Also find the lateral shift of the central maxima and number of fringes crossed through center.
The correct Answer is:0,1.5mm
 After the thin plate is placed between one of the slits and the screen, an additional path difference [ΔP=(μ−1)t] is created between the rays emerging out of S1andS2. Thus
After the thin plate is placed between one of the slits and the screen, an additional path difference [ΔP=(μ−1)t] is created between the rays emerging out of S1andS2. Thus
S1O−S2O=(μ−1)t=0.75×10−6m
(μ−1)tλ=32⇒(μ−1)t=32λ
Point O would be an interference minimum. Thus intensity at O is zero.
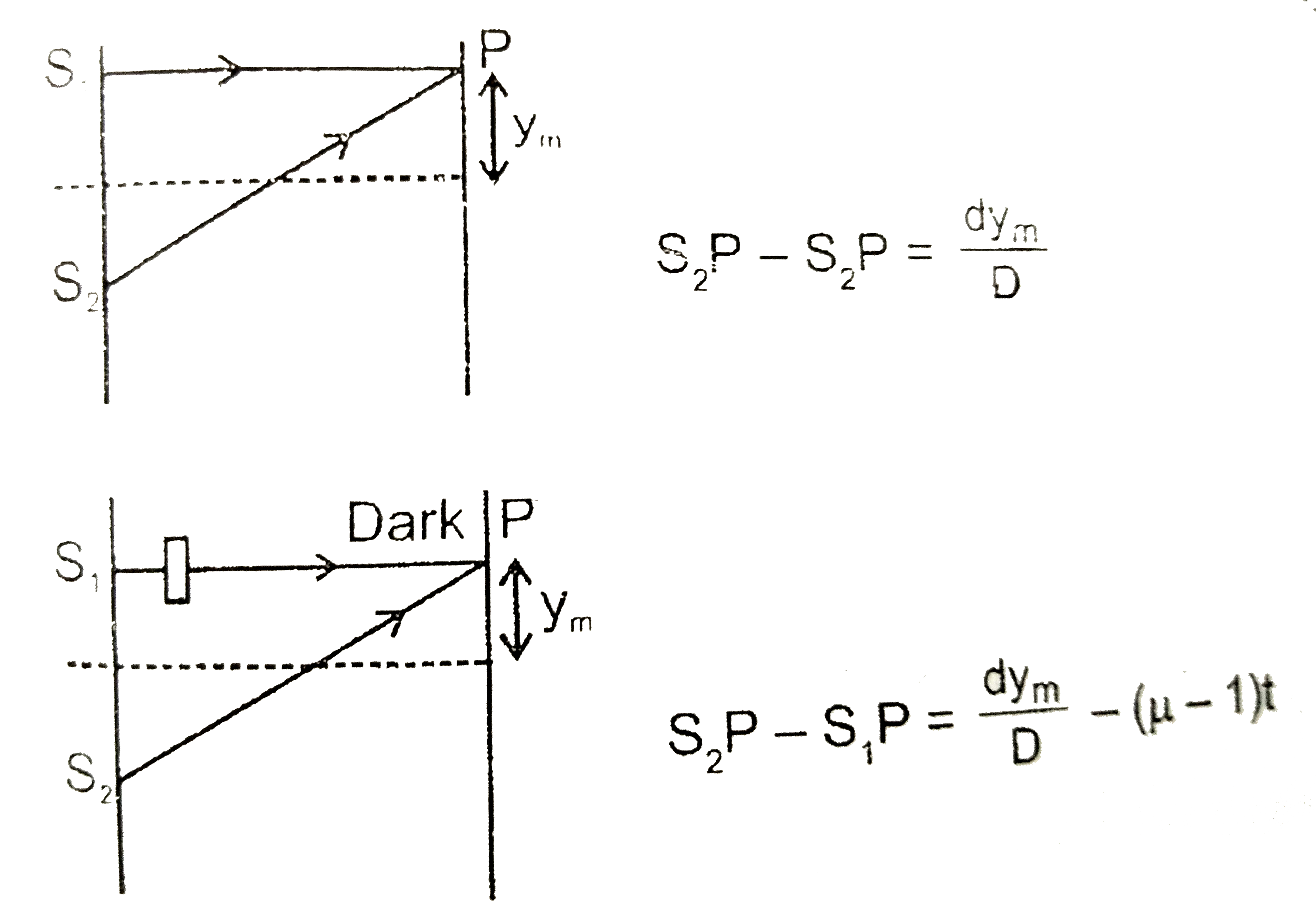
S1P−S2P=dymD
S2P−S1P=dymD−(μ−1)t
For central maxima, the path difference between the interfering beams is zero.
i.e.S2P−S1P=0
⇒dymD=(μ−1)t⇒ym=Dd(μ−1)t=15×10−4×0.75×10−6=1.5mm
Thus, the effect of putting a thin film in front of one of the slits is to shift the central maxima towards that alit.
Topper's Solved these Questions
Similar Questions
A monochromatic light of λ=5000Å is incident on two identical slits separated by a distance of 5×10−4 m. The interference pattern is seen on a screen placed at a distance of 1 m from the plane of slits. A thin glass plate of thickness 1.5×10−6 m and refractive index μ=1.5 is placed between one of the slits and the screen . Find the intensity at the center of the screen.
View SolutionA thin glass plate of thickness t and refractive index μ is inserted between screen and one of the slits in a young's experiment. If the intensity at the centre of the screen is I, what was the intensity at the same point prior to the introduction of the sheet.
View SolutionTwo slits in YDSE are placed 1 mm from each other. Interference pattern is observed on a screen placed 1m from the plane of slits. What is the angular fringe width for a light of wavelength 400 nm
View SolutionTwo slits in YDSE are placed 2 millimeter from each other. Interference pattern is observed on a screen placed 2 m from the plane of slits. What is the fringe width for a light of wavelength 400 nm?
View SolutionIn Yonung's double-slit experiment, two slits which are separated by 1.2 mm are illuminated with a monochromatic light of wavelength 6000Å The interference pattern is observed on a screen placed at a distance of 1 m from the slits. Find the number of bright fringes formed over 1 cm width on the screen.
View SolutionTwo slits at a distance of 1mm are illuminated by a light of wavelength 6.5×10−7m. The interference fringes are observed on a screen placed at a distance of 1m. The distance between third dark fringe and fifth bright fringe will be
View SolutionIn a YDSE, distance between the slits and the screen is 1m, separation between the slits is 1mm and the wavelength of the light used is 5000nm. The distance of 100th maxima from the central maxima is:
View SolutionA young's double slit apporatus is immersed in a liquid of refractive index 1.33.It has slit separation of 1 mm and interference pattern is observed on the screen at a distance 1.33 m from plane of slits.The wavelength in air is 6300Å
Calculate the fringe width.
View SolutionLight of wavelength 5000A0 is incident on a slit. The first minimum of the diffraction pattern is observed to lie at a distance of 5 mm from the central maximum on a screen placed at a distance of 3 m from the slit. Then the width of the slit is
View SolutionA plane monochromatic light wave falls normally on a diaphragm with two narrow slits separated by 2.5mm. The fringe pattern is formed on a screen 100 cm behind the diaphragm. By what distance will these fringes be displaced, when one of the slits is covered by a glass plate (μ=1.5) of thickness 10μm?
View Solution
