A biconvex lens is used to project a slide on screen. The slide is 2cm high and placed at 10cm from the lens. The image is 18cm high. What is the focal length of the lens?
9cm
18cm
4.5cm
20cm
The correct Answer is:A
To find the focal length of the biconvex lens used to project a slide, we can follow these steps:
Step 1: Identify Given Values
- Height of the object (slide), Ho=2cm
- Distance of the object from the lens, U=−10cm (the object distance is taken as negative in lens formula)
- Height of the image, Hi=18cm
Step 2: Calculate Magnification
The magnification m of the lens is given by the formula:
m=HiHo=−VU
Where V is the image distance.
Substituting the known values:
m=182=9
Since the image is real and inverted, we can write:
9=−V−10
Thus, we can solve for V:
V=9×10=90cm
Step 3: Use the Lens Formula
The lens formula relates the object distance U, image distance V, and focal length F:
1F=1V−1U
Substituting the values we found:
1F=190−1−10
This simplifies to:
1F=190+110
Converting 110 to a common denominator:
110=990
Thus:
1F=190+990=1090
Step 4: Calculate the Focal Length
Now, we can find F:
F=9010=9cm
Final Answer
The focal length of the lens is 9cm.
---
- Height of the object (slide), Ho=2cm
- Distance of the object from the lens, U=−10cm (the object distance is taken as negative in lens formula)
- Height of the image, Hi=18cm
The magnification m of the lens is given by the formula:
m=HiHo=−VU
Where V is the image distance.
m=182=9
Since the image is real and inverted, we can write:
9=−V−10
Thus, we can solve for V:
V=9×10=90cm
The lens formula relates the object distance U, image distance V, and focal length F:
1F=1V−1U
1F=190−1−10
This simplifies to:
1F=190+110
Converting 110 to a common denominator:
110=990
Thus:
1F=190+990=1090
Now, we can find F:
F=9010=9cm
The focal length of the lens is 9cm.
Topper's Solved these Questions
- GEOMATRICAL OPTICS BOOK - RESONANCE ENGLISHCHAPTER - GEOMATRICAL OPTICS EXERCISE - Exercise-2
62 Videos
- GEOMATRICAL OPTICS BOOK - RESONANCE ENGLISHCHAPTER - GEOMATRICAL OPTICS EXERCISE - Exercise-3
80 Videos
- GEOMATRICAL OPTICS BOOK - RESONANCE ENGLISHCHAPTER - GEOMATRICAL OPTICS EXERCISE - Problem
33 Videos
- EXPERIMENTAL PHYSICSBOOK - RESONANCE ENGLISHCHAPTER - EXPERIMENTAL PHYSICSEXERCISE - PART -II
10 Videos
- GRAVITATIONBOOK - RESONANCE ENGLISHCHAPTER - GRAVITATIONEXERCISE - HIGH LEVEL PROBLEMS
16 Videos
Similar Questions
A convex lens is used to obtain a magnified image of an object on a screen . The object is at a distance 10 m from the lens . If the magnification is 19. the focal length of the lens is
View SolutionA convex lens has a focal length of 20 cm. It is used to form an image of an object placed 15 cm from lens. The image is
View Solution
Knowledge Check
A convex lens of focal length 15 cm is placed on a plane mirror. An object is placed at 30 cm from the lens. The image is
Areal, at 30 cm in front of the mirrorBreal, at 30 cm behind the mirrorCreal, at 10 cm in front of the mirrorDvirtual, at 10 cm behind the mirrorA concave lens is placed in contact with a convex lens of focal length 25cm. The combination produces a real image at a distance of 80cm, when an object is at a distance of 40cm. What is the focal length of concave lens ?
A−400cmB−200cmC+400cmD+200cm
Find the distance of an object from a convex lens if image is
two times magnified. Focal length of the lens is 10cm
View SolutionFind the distance of an object from a convex lens if image is
two times magnified. Focal length of the lens is 10cm
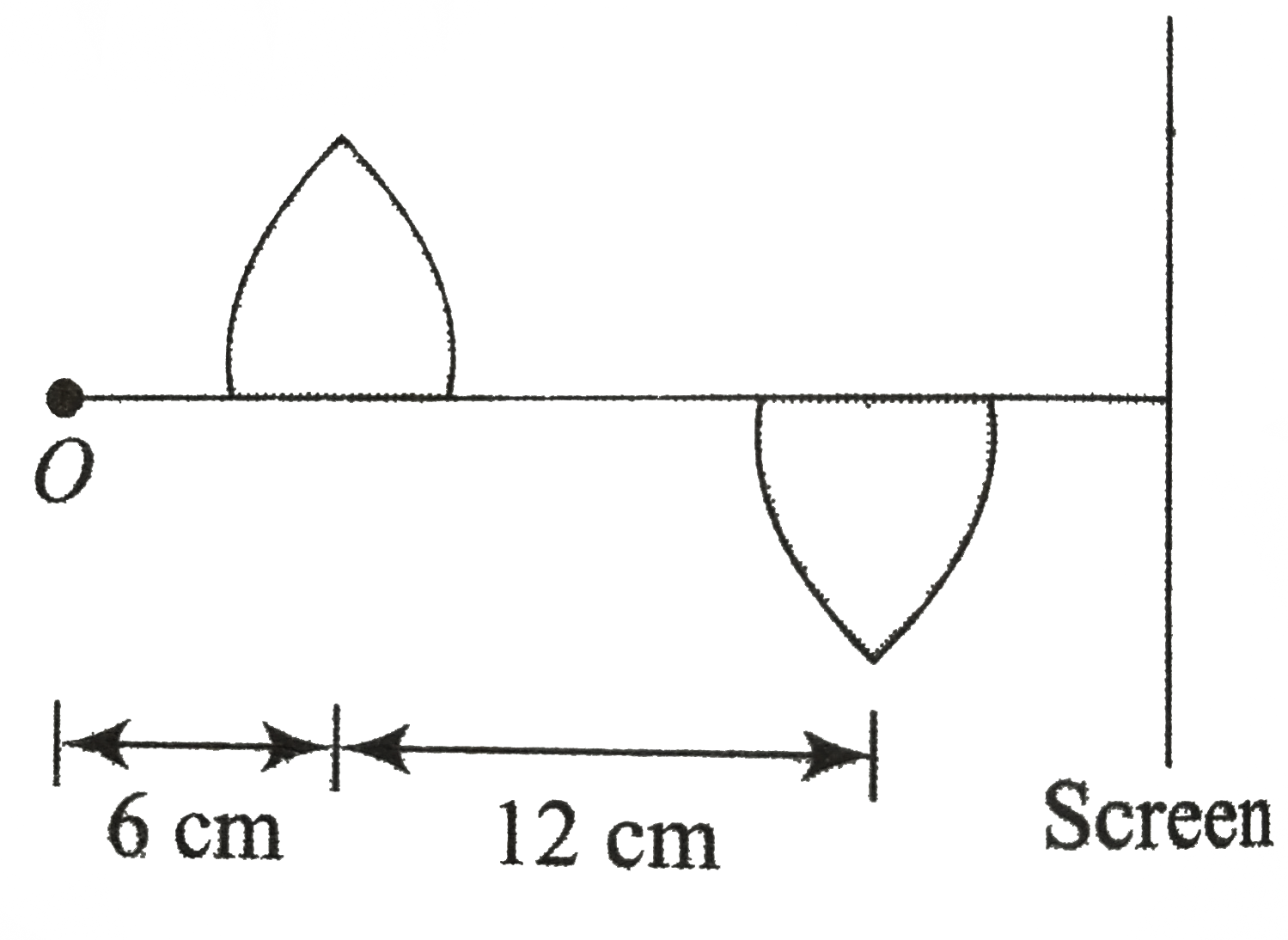
View SolutionA convex lens is cut in half along its principal axis and the two halves are separated by a distance of 12cm. An object is placed 6cm in front of the lens as shown in Figure., Two sharp images are formed on the screen placed 80cm from the object. What is the focal length of the lens?
View SolutionA convex lens is used to throw on a screen 10m from the lens, a magnified image of an object. If the magnification is to be 19, find the focal length of the lens.
View SolutionA convex lens forms an image of an object on a screen 30 cm from the lens. When the lens is moved 90 cm towards the object, then the image is again formed on the screen. Then, the focal length of the lens is
View SolutionAn object is kept at a distance of 16cm from a thin lens and the image formed is real. If the object is kept at a distance of 6cm from the lens, the image formed is virtual. If the sizes of the images formed are equal, the focal length of the lens will be
View Solution
