Compounds A and B react with a common reagent with first order kinetics in both cases. If 99% of A must react before 1% of B has reacted, what is the minimum ratio for their respective rate constants ?
916
229
500
458
The correct Answer is:D
To solve the problem, we need to determine the minimum ratio of the rate constants kA and kB for the reactions of compounds A and B with a common reagent, given that 99% of A must react before 1% of B has reacted.
1. Understanding the Reaction:
- We know that both compounds A and B react with a common reagent and that the reactions follow first-order kinetics.
2. Defining the Reacted and Remaining Fractions:
- When 99% of A has reacted, the remaining fraction of A is:
(A)=1−0.99=0.01
- At this point, only 1% of B has reacted, so the remaining fraction of B is:
(B)=1−0.01=0.99
3. Using the First-Order Kinetics Formula:
- For a first-order reaction, the rate constant k can be expressed in terms of the fraction of the reactant that has reacted:
k=1tln((A)0(A))
- For compound A, since 99% has reacted:
kA=1tAln(1001)=1tAln(100)
4. Calculating for Compound B:
- For compound B, since only 1% has reacted:
kB=1tBln(10099)
5. Finding the Ratio of Rate Constants:
- We need to find the ratio kAkB:
kAkB=1tAln(100)1tBln(10099)=tBln(100)tAln(10099)
6. Assuming Time Relation:
- Since we want the minimum ratio, we can assume tA=tB for simplicity, leading to:
kAkB=ln(100)ln(10099)
7. Calculating the Logarithms:
- We know:
ln(100)=4.605
ln(10099)=ln(100)−ln(99)≈4.605−4.595=0.01005
8. Final Calculation:
- Now substituting the values:
kAkB≈4.6050.01005≈458.7
Conclusion:
The minimum ratio of the rate constants kAkB is approximately 458.
- We know that both compounds A and B react with a common reagent and that the reactions follow first-order kinetics.
- When 99% of A has reacted, the remaining fraction of A is:
(A)=1−0.99=0.01
- At this point, only 1% of B has reacted, so the remaining fraction of B is:
(B)=1−0.01=0.99
- For a first-order reaction, the rate constant k can be expressed in terms of the fraction of the reactant that has reacted:
k=1tln((A)0(A))
- For compound A, since 99% has reacted:
kA=1tAln(1001)=1tAln(100)
- For compound B, since only 1% has reacted:
kB=1tBln(10099)
- We need to find the ratio kAkB:
kAkB=1tAln(100)1tBln(10099)=tBln(100)tAln(10099)
- Since we want the minimum ratio, we can assume tA=tB for simplicity, leading to:
kAkB=ln(100)ln(10099)
- We know:
ln(100)=4.605
ln(10099)=ln(100)−ln(99)≈4.605−4.595=0.01005
- Now substituting the values:
kAkB≈4.6050.01005≈458.7
The minimum ratio of the rate constants kAkB is approximately 458.
Topper's Solved these Questions
Similar Questions
Knowledge Check
A first order reaction is 20% complete in 10 minutes. What is the specific rate constant for the reaction?
A0.0970 −1minB0.009 min−1C0.0223 min−1D2.223min−1Compounds 'A' and 'B' react according to the following chemical equation.
A(g)→2B(g)→2C(g)
Concentration of either 'A' or 'B' were changed Keeping the concentration of one of the reactants constant and rates were measured as a function of initial concentration. Following result were obtained.
Choose the correct option for the rate equations for this reaction.
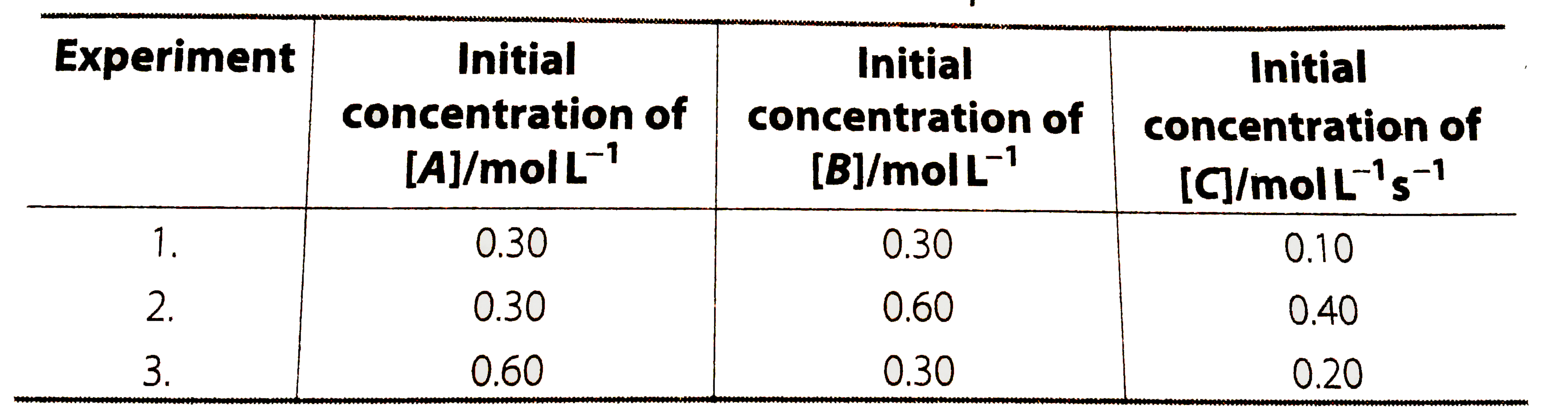 ARate =k[A]2[B]BRate =k[A][B]2CRate =k[A][B]DRate =k[A]2[B]∘
ARate =k[A]2[B]BRate =k[A][B]2CRate =k[A][B]DRate =k[A]2[B]∘Compounds 'A' and 'B' react according to the following chemical equation.
A(g)→2B(g)→2C(g)
Concentration of either 'A' or 'B' were changed Keeping the concentration of one of the reactants constant and rates were measured as a function of initial concentration. Following result were obtained.
Choose the correct option for the rate equations for this reaction.
 ARate =k[A]2[B]BRate =k[A][B]2CRate =k[A][B]DRate =k[A]2[B]∘
ARate =k[A]2[B]BRate =k[A][B]2CRate =k[A][B]DRate =k[A]2[B]∘
A first order reaction is 50% complete in 20 minutes. What is its rate constant ?
View SolutionA first order reaction is 50% complete in 20 minutes. What is its rate constant ?
View SolutionA first order reaction is 50% complete in 20 minutes. What is its rate constant ?
View SolutionCompounds 'A' and 'B' react according to the following chemical equation.
A(g)→2B(g)→2C(g)
Concentration of either 'A' or 'B' were changed Keeping the concentration of one of the reactants constant and rates were measured as a function of initial concentration. Following result were obtained.
Choose the correct option for the rate equations for this reaction.

View SolutionCompounds 'A' and 'B' react according to the following chemical equation.
A(g)→2B(g)→2C(g)
Concentration of either 'A' or 'B' were changed Keeping the concentration of one of the reactants constant and rates were measured as a function of initial concentration. Following result were obtained.
Choose the correct option for the rate equations for this reaction.

View Solution
