Consider the following case of competing 1st order reactions.
![Consider the following case of competing 1^(st) order reactions. After the start of the reaction at t=0 with only A,the [C] is equal to the [D] at all time.The time in which all three concentrations wil be equal is given by :](https://d10lpgp6xz60nq.cloudfront.net/physics_images/RES_PHY_RK_JA_C04_E01_193_Q01.png)
After the start of the reaction at t=0 with only A,the [C] is equal to the [D] at all time.The time in which all three concentrations wil be equal is given by :
t=12k1ln3
t=12k2ln3
t=13k1ln2
t=13k2ln2
The correct Answer is:A,B
k1=k2
⇒23rd of A has reacted for [A] =[C]=[D]
∴K1+k2=1tIn[A]013[A]0
⇒t=1k1+k2In3=12k1In3=12k2In3
Topper's Solved these Questions
Similar Questions
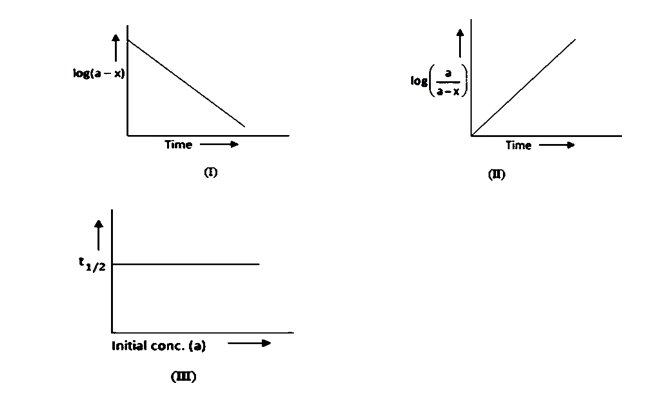
Which of the following is/are correct for the first order reaction ? (a is initial concentration of reactant, x is concentration of the reactant reacted and t is time)
View SolutionConsider the following in respect of zero order reaction.
I. t1/2 is directly proportional to the initial concentration
II. Time taken for the completion of the reaction is twice its t1/2
III Concentration of the reactant decreases linearly with time
Which of the statements given above are correct ?
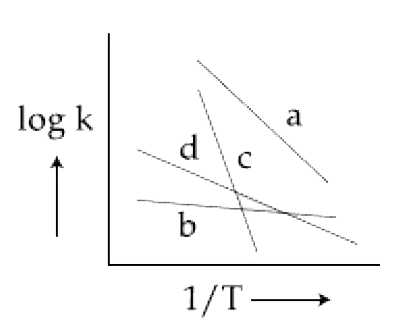
View SolutionConsider the following plots of rate constant versus 1T for four different reactions. Which of the following orders is correct for the activation energies of these reactions ?
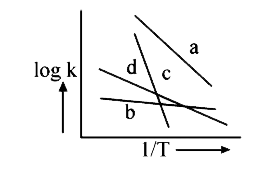
View SolutionConsider the following plots of rate constant versus 1T for four different reactions. Which of the following orders is correct for the activation energies of these reactions?
View SolutionFor the second order reaction, concentration (x) of the product at time t starting with initial concentration [A]o is :
View SolutionA : The rate constant of zero order reaction is equal to rate of reaction.
R : t1/2 for zero order reaction is directly proportional to initial concentration.
View SolutionThe rate for a first order reaction is 0.6932×10−2molL−1min−1 and the initial concentration of the reactants is 1M, T1/2 is equal to
View SolutionThe rate for a first order reaction is 0.6932×10−2molL−1min−1 and the initial concentration of the reactants is 1M, T1/2 is equal to
View SolutionIF the t1/2 for a first order reaction 0.4 min, the time of or 99.9% completion of the reaction is ............min.
View SolutionIn a first order reaction, the concentrations of the reactant, 30 minutes and 40 minutes after the start are C1 and C2( in moles / litre ) respectively . What was C0, initial concentration ?
View Solution