In the following 'I' refers to current and other symbols have their usual meaning. Chosse the option that corresponds to the dimensions of electrical conductivity:
M−1L−3T3I2
M−1L3T3I
ML−3T−3I2
M−1L−3T3I
The correct Answer is:A
To find the dimensions of electrical conductivity, we start from the relationship between resistance, resistivity, and conductivity. The electrical conductivity (σ) is defined as the inverse of resistivity (ρ), and resistivity is related to resistance (R) through the formula:
σ=1ρ
Resistance (R) can be expressed in terms of resistivity (ρ) as follows:
R=ρLA
Where:
- R = Resistance
- ρ = Resistivity
- L = Length of the conductor
- A = Cross-sectional area of the conductor
From this, we can express resistivity in terms of resistance:
ρ=RAL
Now, substituting this into the equation for conductivity:
σ=1ρ=ARL
Next, we need to find the dimensions of each term in this equation.
1. Dimensions of Resistance (R):
The formula for power in terms of current (I) and resistance (R) is given by:
P=I2R
Power (P) has dimensions of work done per unit time:
(P)=(W)(T)=ML2T−2T=ML2T−3
Rearranging the power equation gives us:
R=PI2
Thus, the dimensions of resistance can be expressed as:
(R)=(P)(I)2=ML2T−3I2
2. Substituting for Resistance:
Now substituting the dimensions of resistance into the conductivity equation:
(σ)=(A)(R)(L)=(A)(ML2T−3I2)(L)
The dimensions of area (A) are:
(A)=L2
Thus, substituting this into the conductivity equation gives:
(σ)=L2(ML2T−3I2)L=L2I2T−3ML3
3. Simplifying the Dimensions:
Simplifying this expression:
(σ)=I2ML
4. Final Dimensions of Electrical Conductivity:
Therefore, the dimensions of electrical conductivity are:
(σ)=M−1L−3T3I2
Final Answer:
The dimensions of electrical conductivity are:
M−1L−3T3I2
- R = Resistance
- ρ = Resistivity
- L = Length of the conductor
- A = Cross-sectional area of the conductor
The formula for power in terms of current (I) and resistance (R) is given by:
Now substituting the dimensions of resistance into the conductivity equation:
Simplifying this expression:
Therefore, the dimensions of electrical conductivity are:
The dimensions of electrical conductivity are:
Topper's Solved these Questions
- TEST PAPERSBOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PART - II PHYSICS
106 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Advanced Level Problems
13 Videos
- TEST SERIESBOOK - RESONANCE ENGLISHCHAPTER - TEST SERIESEXERCISE - PHYSICS
130 Videos
Similar Questions
Which of the following formula is correct ? [ Where symbols have their usual meaning ]
View SolutionSymbols have their usual meanings. Match the Column-I with Column-II:
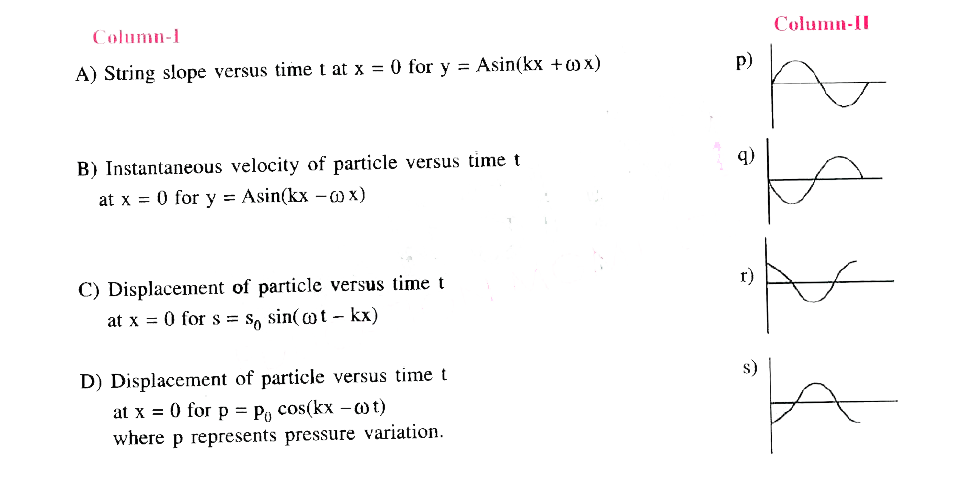
View SolutionBohr magnetonis given by (symbols have their usual meanings)
View SolutionSelect the incorrect relation. (Where symboles have their usual meanings):-
View SolutionDisplacement current can be expressed as (symbols have their usual meanings)
View Solutionthe correct form of Strokes law is (symbols have their usual meanings)
View SolutionDimensions of 1μ0∈0 , where symbols have their usual meaning are
View SolutionThe poynting vector in vaccum is represented by (where symbols have their usual meanings)
View SolutionWhich of the following is correct ? (symbols have their ususal meanings)
View SolutionWhich of the following is correct ? (symbols have their ususal meanings)
View Solution
