Starting with a sample of pure 66Cu,78 of it decays into Zn in 15 min. The corresponding half-life is
10 minute
15 minute
5 minute
712minute
The correct Answer is:C
N=N0(1−e−λt)
⇒N0−NN0=e−λt
∴18=e−λt
⇒8=eλtt ⇒3ln2=λt ⇒=3×0.69315
t1/2=0.6933×0.693×15 t1/2=5min
Topper's Solved these Questions
- NUCLEAR PHYSICSBook:RESONANCE ENGLISHChapter:NUCLEAR PHYSICSExercise:Exercise -3 Part-III CBSE PROBLEMS (LAST 10 YEARS)
Explore 34 Videos
- NUCLEAR PHYSICSBook:RESONANCE ENGLISHChapter:NUCLEAR PHYSICSExercise:Advanced level solutions
Explore 16 Videos
- NUCLEAR PHYSICSBook:RESONANCE ENGLISHChapter:NUCLEAR PHYSICSExercise:Exercise 3 Part -1 JEE (Advanced)
Explore 19 Videos
- GRAVITATIONBook:RESONANCE ENGLISHChapter:GRAVITATIONExercise:HIGH LEVEL PROBLEMS
Explore 16 Videos
- REVISION DPPBook:RESONANCE ENGLISHChapter:REVISION DPPExercise:All Questions
Explore 463 Videos
Similar Questions
Knowledge Check
Radioactive carbon dating can determine how long ago an organism lived by measuring how much of thte 14C in the sample has decayed. 14C is an isotope of carbon that has a half-life of 5,600 years.Half-life is the amount of time it takes for half of the original amount to decay. If a sample of a petrified tree contains 6.25 percent of its original 14C, how long ago did the tree die ?
A22,400 yearsB28,000 yearsC35,000 yearsD89,600 yearsA fraction f1 of a radioactive sample decays in one mean life, and a fraction f2 decays in one half life. Then
Af1>f2Bf1<f2Cf1=f2Deither of (a),(b ) or (c ) depending on the values of the mean life and half life.
8 kg of Cu66 undergones radioactive decay and after 15 minutes only 1 kg remains . The half - life , in minutes , is then
View SolutionThe half life of 2g sample of radioactive nuclide 'X' is 15 min. The half life time of 1g sample of X is
View SolutionA bone containing 200g carbon-14 has β-decay rate of 375 decay/min. Calculate the time that has elapsed since the death of the living one. Given the rate of decay for the living organism is equal to 15 decay per min per gram of carbon and half-life of carbon-14 is 5730years.
View SolutionA bone containing 200 g carbon-14 has β-decay rate of 375 decay/min. Calculate the time that has elapsed since the death of the living one. Given the rate of decay for the living organism is equal to 15 decay per min per gram of carbon and half-life of carbon-14 is 5730 years.
View SolutionThe probability that particular nucleus of 38Cl will undergo β-decay in time interval 10−3 sec (the half life of 38Cl is 37.2 min) is
View SolutionThe graph represents the decay of a newly-prepared sample of radioactive nuclide X to a stable nuclide Y. The half-life of X is t. The growth curve for Y intersects the decay curve for X after time T. What is the time T ?
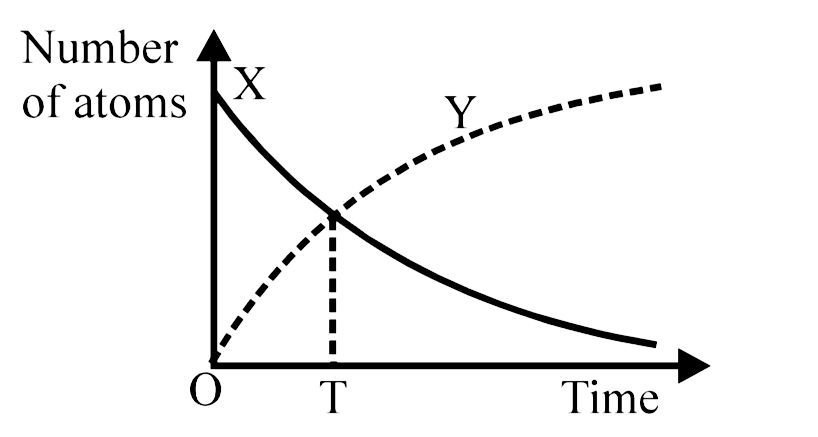
View Solution