A metre stick swinging in vertical plane about a fixed horizontal axis passing through its one end undergoes small oscillation of frequency f0. If the bottom half of the stick were cut off, then its new frequency of small oscillation will become.
f0
√2f0
2f0
2√2f0
The correct Answer is:B
f0=12π√mglI
Where, l is distance between point of suspension and centre of mass of the body.
thus, for the stick of length L and mass m,
f0=12π
⎷mgL2(mL2/12)=12π√6gL
When bottom half of the stick is cut of
f'0=12π
⎷m2.g.L4m2(L/2)212
=12π×√12gL=√2f0
Related Playlists
Similar Questions

A metre stick swinging in vertical plane about a fixed horizontal axis passing through its one end undergoes small oscillation of frequency f0. If the bottom half of the stick were but off, then its new frequency of small oscillation woul become.View SolutionA metre stick swinging about its one end oscillates with frequency f0. If the bottom half of the stick was cut off, then its new oscillation frequency will be :
View SolutionA magnetic needle vibrates in a vertical plane parallel to the magnetic meridlian about a horizontal axis passing through its centre. Its frequency is π. If the plane of oscillation is turned about a vertical axis by 90∘, the frequency of oscillation in vertical plane will be :
View SolutionA uniform disc is rotating at a constantt speed in a vertical plane about a fixed horizontal axis passing through the centre of the disc. A piece of the disc from its rim detaches itself from the disc at the instant when it is at horizontal level with the centre of the disc and moving upward. Then about the fixed axis, the angular speed of the
View SolutionAn uniform disc is rotating at a constant speed in a vertical plane about a fixed horizontal axis passing through the centre of the disc. A piece of the disc from its rim detaches itself from the disc at the instant when it is at horizontal level with the centre of the disc and moving upwards, then about the fixed axis.
STATEMENT-1 : Angular speed of the disc about the aixs of rotation will increase.
and
STATEMENT-2 : Moment of inertia of the disc is decreased about the axis of rotation.View SolutionA massless stick of length L is hinged at one end and a mass m attached to its other end. The stick is free to rotate in vertical plane about a fixed horizontal axis passing through frictionless hinge. The stick is held in a horizontal position. At what distance x from the hinge should a second mass M = m be attached to the stick, so that stick falls as fast as possible when released from rest
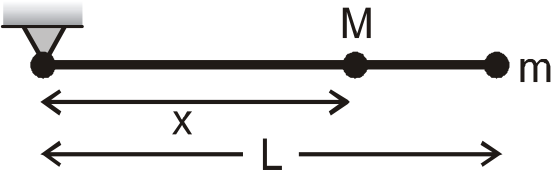 View Solution
View SolutionA uniform rod of length l oscillates about an axis passing through its end. Find the oscillation period and the reduced length of this pendulum.
View SolutionA ring of the radius r is suspended from a point on its circumference. If the ring is made to oscillate in the plane of the figure, then the angular frequency of these small oscillations is
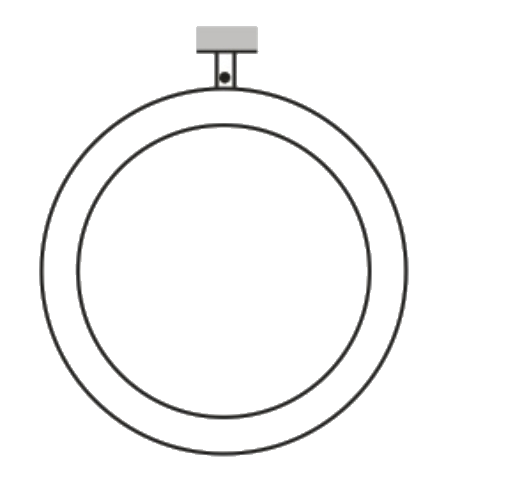 View Solution
View SolutionAn almost inertia-less rod of length l3.5 m can rotate freely around a horizontal axis passing through its top end. At the bottom end of the roa a small ball of mass m andat the mid-point another small ball of mass 3m is attached. Find the angular frequency (in SI units) of small oscillations of the system about the equilibrium position. Gravitational acceleration is g=9.8 m//s^(2).
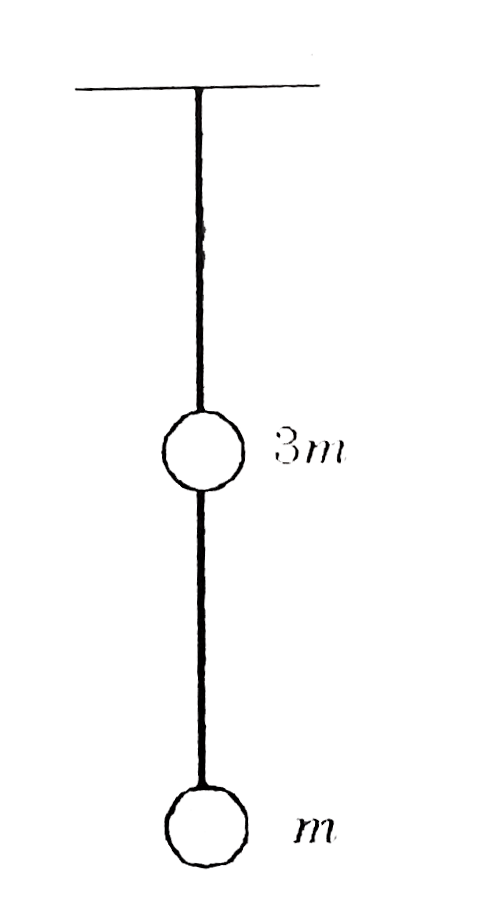 View Solution
View SolutionA uniform rod of mass m and length L is free to rotate in the vertical plane about a horizontal axis passing through its end. The rod initially in horizontal position is released. The initial angular acceleration of the rod is:
 View Solution
View Solution