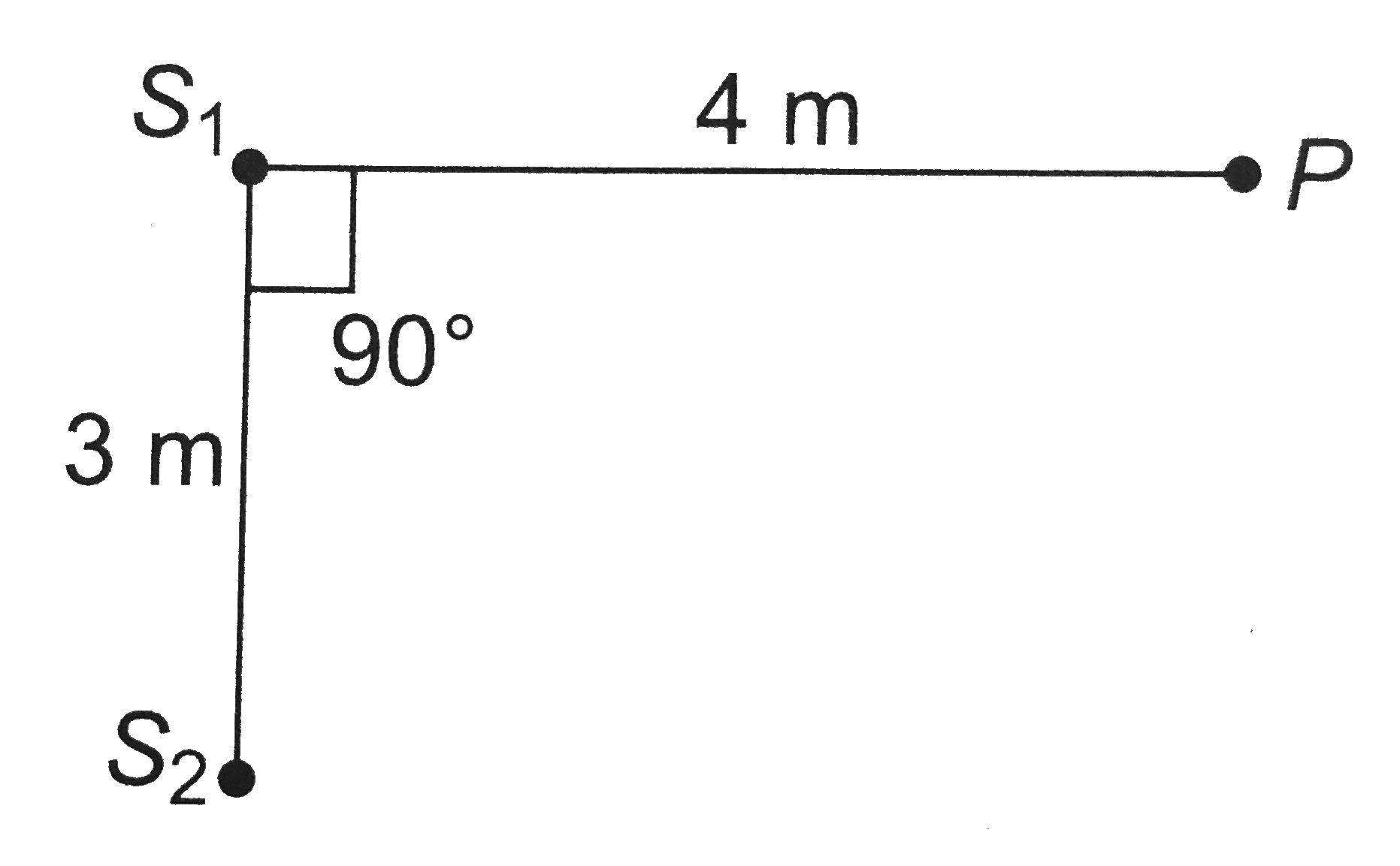
S1 and S2 are two coherent sources of sound of frequency 110Hz each they have no initial phase difference. The intensity at a point P due to S1 is I0 and due to S2 is 4I0. If the velocity of sound is 330m/s then the resultant intensity at P is
I0
9I0
3I0
8I0
The correct Answer is:C
The wavelength of sound source =330110=3 meter.
The phase difference between interferring waves at P is
=Δϕ=2πλ(S2P−S1P)=2π3(5−4)=2π3
∴ Resultant intensity at P=I0+4I0+2√I0√4I0cos.2π3=3I0
Topper's Solved these Questions
Similar Questions
S1 and S2 are two coherent sources of sound having no intial phase difference. The velocity of sound is 330m/s. No maximum will be formed on the line passing through S2 and prependicular to the line joining S1 and S2. If the frequency of both the sources is
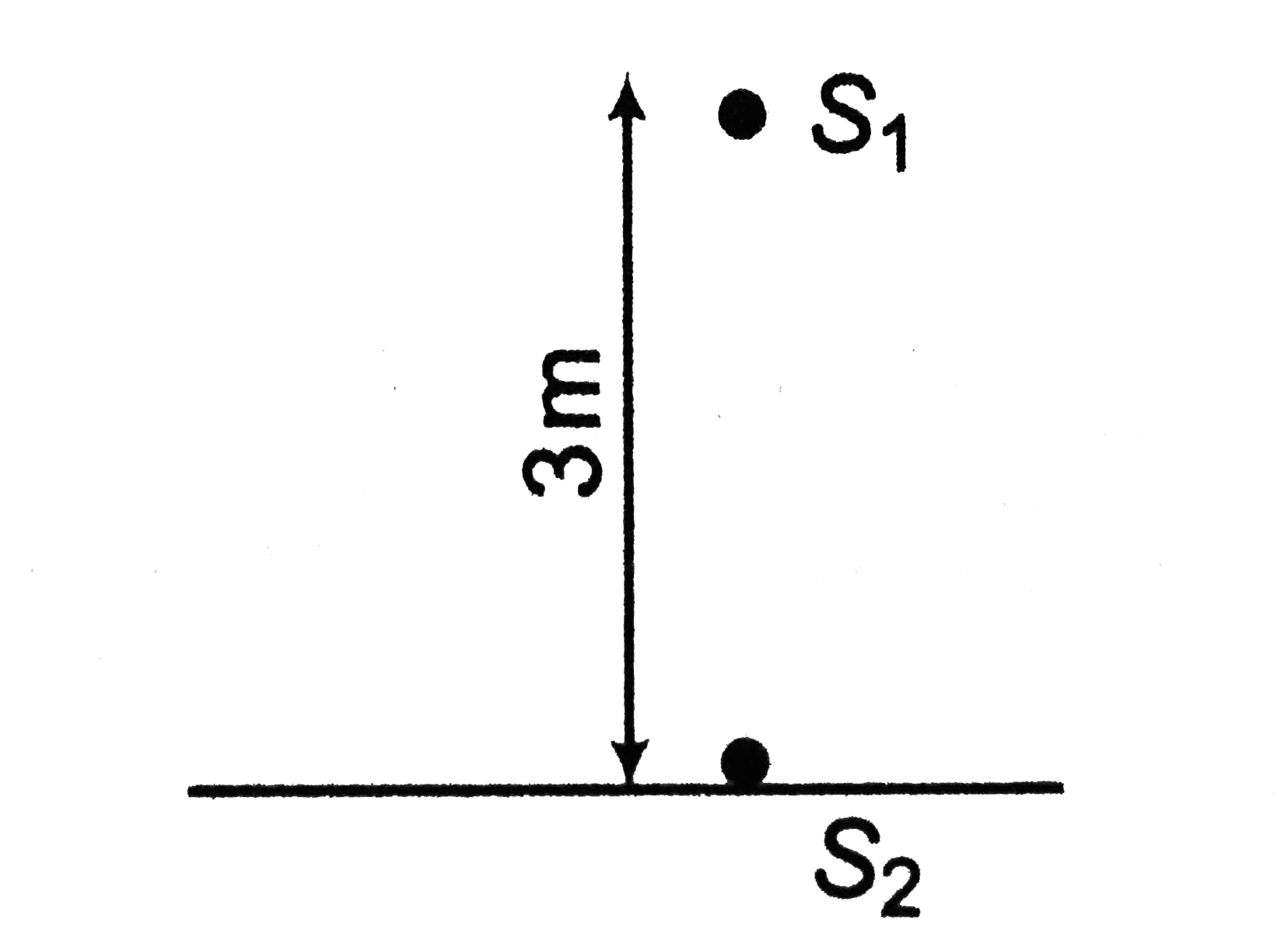
View SolutionConsider an interference arrangement similar to YDSE. Slits S1 and S2 are illuminated with coherent microwave sources each of frequency 2 MHz The sources are synchronized to have zero phase difference. The slits are separated by distance d= 75m. The intensity I(θ) is measured as a function of θ where θ is defined as shown in figure. if I0 is maximum intensity then calculated I(θ) for (i)θ=0∘,(ii)θ=π/6and(iii)θ=π/2
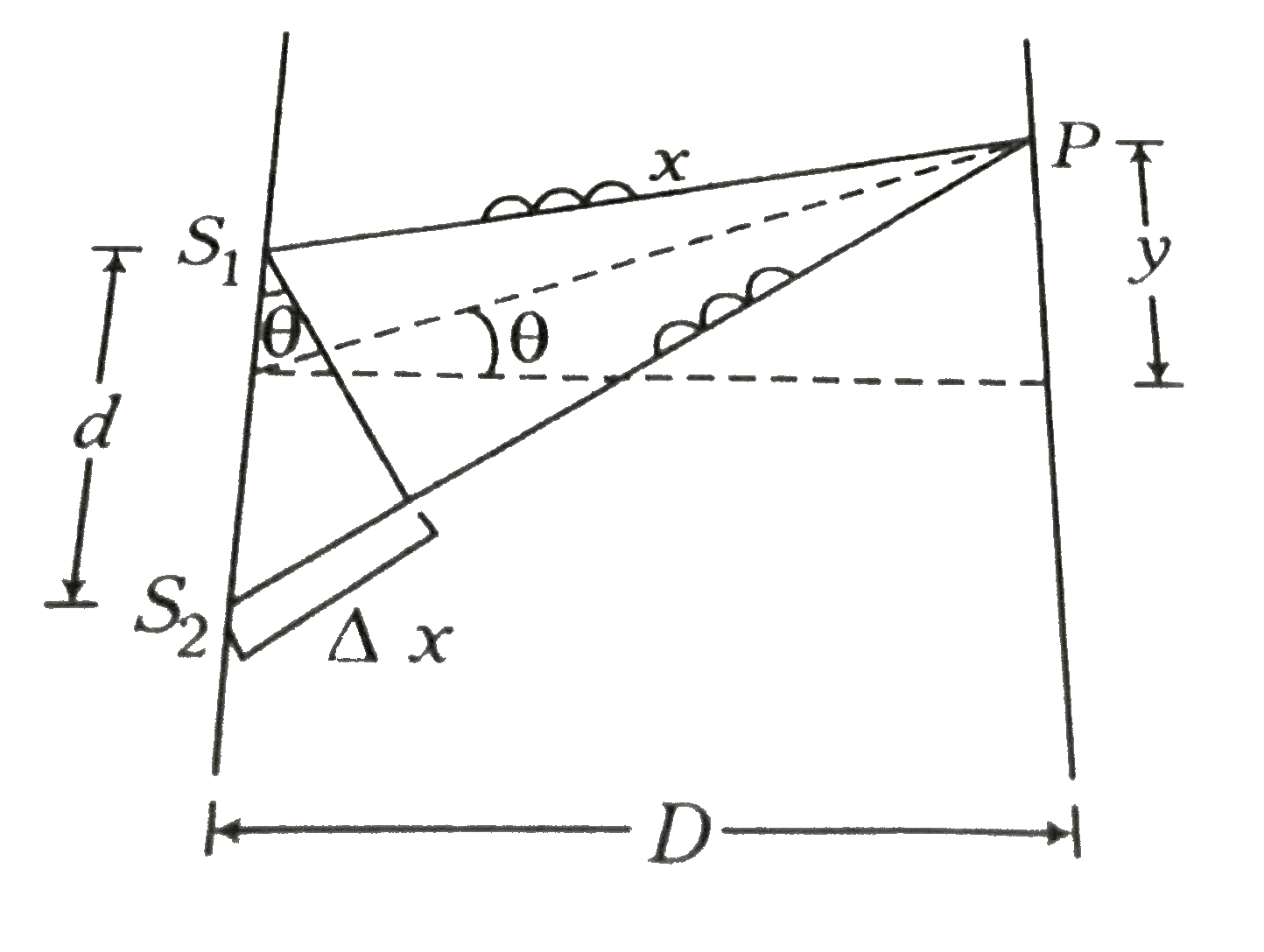
View SolutionIn the figure , the intensity of waves arriving at D from two coherent soucrces s1ands2isI0. The wavelength of the wave is λ=4m . Resultant intensity at D will be

View SolutionIn an interference arrangement similar to Young's double-slit experiment, the slits S_1 and S_2 are illuminated with coherent microwave sources, each of frequency 10^6 Hz. The sources are synchronized to have zero phase difference. The slits are separated by a distance d=150.0 m. The intensity I (theta) is measured as a function of theta, where theta is defined as shown. If I_0 is the maximum intensity, then I (theta) for 0lethetale90degree is given by

View SolutionS1 and S2 are two coherent sources of frequency f each.(θ1=θ2=0∘)Vsound=330m/s.
(i) so that constructive interfence at 'p'
(ii) so that destructive interfrece at 'p'
View SolutionS1 and S2 are two sources of sound emitting sine waves. The two sources are in phase. The sound emitted by the two sources interfers at point F. The waves of wavelength :

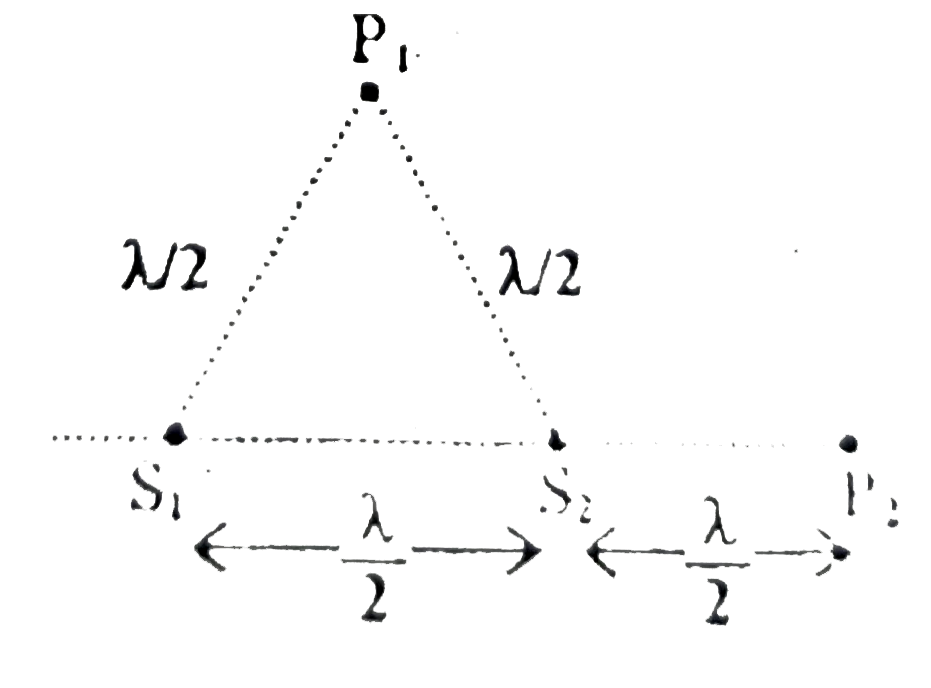
View SolutionS1 and S2 are two equally intense coherent point second sources vibrating in phase. The resultant intensity at P1 is 80SI units then resultant intensity at P2 in SI units will be
View SolutionTwo sources of sound S1 and S2 produce sound waves of same frequency 660Hz. A listener is moving from source S1 towards S2 with a constant speed um/s and he hears 10 beats/s. The velocity of sound is 330m/s. Then, u equals :
View SolutionIntensity due to line source S1 at a distance of r is I0 and intensity due to other line source S2 at a distance of r is 4I0 and frequency of both source are equal. If interference occurs at a point P as shown in the figure and wavelength of both sources is r, find the resultant intensity (in wa/m2) at P. (where I0=2watt/m2)

View SolutionConsider two coherent, monochromatic (wavelength λ) sources S1andS2, separated by a distance d. The ratio of intensities of S1 and that of S2 (which is responsible for interference at point P, where detector is located) is 4. The distance of point P from S1 is (if the resultant intensity at point P is equal to 94 times of intensity of S1) (Given : ∠S2S1P=90∘,d>0andn is a positive integer)
View Solution