A small square loop of wire of side l is placed inside a large square loop of wire of side L(L>>l). The loops are co-planer and their centres coincide. The mutual inductance of the system is proportional to
lL
l2L
Ll
L2l
The correct Answer is:B
To solve the problem of finding the mutual inductance of a small square loop placed inside a larger square loop, we will follow these steps:
Step 1: Understand the Configuration
We have two square loops:
- A small loop with side length l
- A large loop with side length L where L≫l
Both loops are coplanar and their centers coincide.
Step 2: Determine the Magnetic Flux
The magnetic flux (Φ) linked with the larger loop due to the smaller loop can be expressed as:
Φ=B⋅A
where B is the magnetic field due to the smaller loop and A is the area of the larger loop.
The area A of the larger loop is:
A=L2
Step 3: Calculate the Magnetic Field
The magnetic field B at the center of the smaller loop carrying a current I can be derived from Ampere's Law or Biot-Savart Law. For a square loop, the magnetic field at its center is given by:
B=2√2μ0Iπl
where μ0 is the permeability of free space.
Step 4: Substitute into the Flux Equation
Now substituting B into the flux equation:
Φ=(2√2μ0Iπl)⋅L2
Step 5: Calculate Mutual Inductance
The mutual inductance M12 is defined as the ratio of the magnetic flux linked with one loop to the current in the other loop:
M12=ΦI
Substituting the expression for Φ:
M12=2√2μ0L2πl
Step 6: Analyze Proportionality
From the equation M12=2√2μ0L2πl, we can see that the mutual inductance is proportional to:
M12∝l2L
Thus, the mutual inductance of the system is proportional to l2L.
Final Answer
The mutual inductance of the system is proportional to l2L.
---
We have two square loops:
- A small loop with side length l
- A large loop with side length L where L≫l
The magnetic flux (Φ) linked with the larger loop due to the smaller loop can be expressed as:
Φ=B⋅A
where B is the magnetic field due to the smaller loop and A is the area of the larger loop.
A=L2
The magnetic field B at the center of the smaller loop carrying a current I can be derived from Ampere's Law or Biot-Savart Law. For a square loop, the magnetic field at its center is given by:
B=2√2μ0Iπl
where μ0 is the permeability of free space.
Now substituting B into the flux equation:
Φ=(2√2μ0Iπl)⋅L2
The mutual inductance M12 is defined as the ratio of the magnetic flux linked with one loop to the current in the other loop:
M12=ΦI
Substituting the expression for Φ:
M12=2√2μ0L2πl
From the equation M12=2√2μ0L2πl, we can see that the mutual inductance is proportional to:
M12∝l2L
Thus, the mutual inductance of the system is proportional to l2L.
The mutual inductance of the system is proportional to l2L.
Topper's Solved these Questions
Similar Questions
A circular loop of radius r is placed at the center of current carrying conducting square loop of side a. If both loops are coplanar and a >> r, then the mutual inductance between the loops will be
View SolutionA rectangular loop of sides 'a' and 'b' is placed in xy plane. A very long wire is also placed in xy plane such that side of length 'a' of the loop is parallel to the wire. The distance between the wire and the nearest edge of the loop is 'd'. The mutual inductance of this system is proportional to :
View Solution
Knowledge Check
A small square loop of wire of side l is placed inside a large square loop of wire of side L(L>>l). The loops are coplanar and their centre coincide. What is the mutual inductance of the system ?
A2√2μ0πl2LB8√2μ0πl2LC2√2μ02πl2LD2√2μ0L2πl
A square conducting loop of side L is situated in gravity free space. A small conducting circular loop of redius r(r<<L) is placed at the center of the square loop, with its plane perpendicular to the plane of the square loop. The mutual inductance of the two coils is
View SolutionA small square loop of n1 turns and side l is placed at the centre of a large coplanar, circular loop of radius r and n2 tuns. The mutual inductacne of the combination is
View SolutionA square conducting loop of side L is situated in gravity free space. A small conducting circular loop of radius r(r<<L) is placed at the center of the square loop, with its plane perpendicular to the plane of the square loop. The mutual inductance of the two coils is
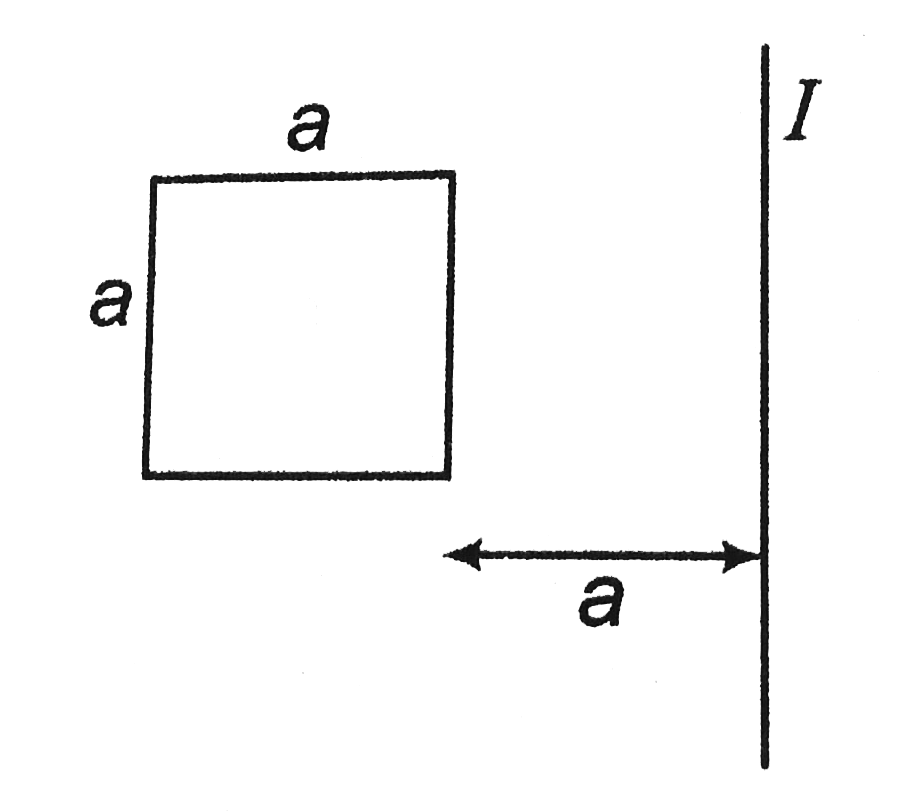
View SolutionAn infinitely long wire is placed near a square loop as shown in figure. Choose the correct options.
View SolutionA square loop of sides L is placed at centre of a circular loop of radius r (r << L) such that they are coplanar, then mutual inductance between both loop is
View SolutionA small circular loop of wire of radius a is located at the centre of a much larger circular wire loop of radius b. The two loops are in the same plane. The outer loop of radius b carries an alternating current I=I0cos(ωt). The emf induced in the smaller inner loop is nearly ?
View SolutionThe conducting circular loops of radii R1andR2 are placed in the same plane with their centres coinciding. If R1>>R2, the mutual inductance M between them will be directly proportional to
View Solution
