A metal rod of length L is placed normal to a magnetic field and rotated through one end of rod in circular path with frequency f. The potential difference between it ends will be-
πL2Bf
BL/f
πL2B/f
fBL
The correct Answer is:A
To find the potential difference between the ends of a metal rod of length L that is rotated in a magnetic field with frequency f, we can follow these steps:
1. Understand the Setup:
- A metal rod of length L is placed perpendicular (normal) to a magnetic field B.
- The rod is rotated about one of its ends in a circular path with a frequency f.
2. Identify the Formula for EMF:
- The electromotive force (emf) induced in a rotating rod in a magnetic field is given by the formula:
E=12BωL2
where E is the induced emf, B is the magnetic field strength, ω is the angular velocity, and L is the length of the rod.
3. Convert Frequency to Angular Velocity:
- The angular velocity ω can be related to the frequency f by the formula:
ω=2πf
4. Substitute ω into the EMF Formula:
- Replace ω in the emf formula:
E=12B(2πf)L2
5. Simplify the Expression:
- Simplifying the equation gives:
E=BπfL2
6. Final Result:
- Therefore, the potential difference (emf) between the ends of the rod is:
E=πL2Bf
Conclusion:
The potential difference between the ends of the rod is given by:
E=πL2Bf
- A metal rod of length L is placed perpendicular (normal) to a magnetic field B.
- The rod is rotated about one of its ends in a circular path with a frequency f.
- The electromotive force (emf) induced in a rotating rod in a magnetic field is given by the formula:
E=12BωL2
where E is the induced emf, B is the magnetic field strength, ω is the angular velocity, and L is the length of the rod.
- The angular velocity ω can be related to the frequency f by the formula:
ω=2πf
- Replace ω in the emf formula:
E=12B(2πf)L2
- Simplifying the equation gives:
E=BπfL2
- Therefore, the potential difference (emf) between the ends of the rod is:
E=πL2Bf
The potential difference between the ends of the rod is given by:
E=πL2Bf
Topper's Solved these Questions
Similar Questions
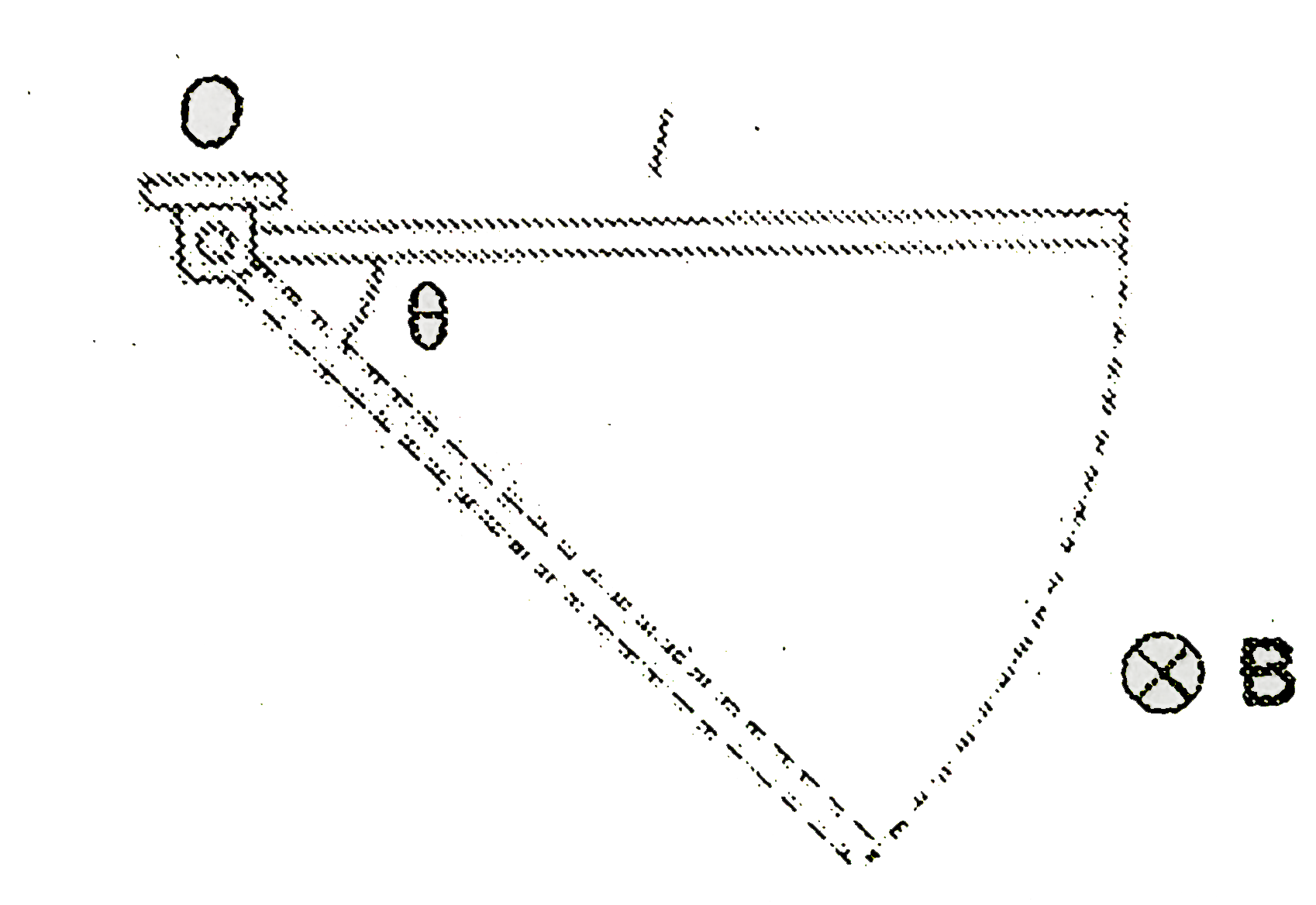
A conducting rod of length l is hinged at point O. It is a free to rotate in a verical plane. There exists a uniform magnetic field B in horizontal direction. The rod is released from the position shown. The potential difference between the two ends of the rod is proportional to
View SolutionA rod of length 20 cm is rotating with angular speed of 100 rps in a magnetic field of strength 0.5 T about it’s one end . What is the potential difference betweet two ends of the rod :
View Solution
Knowledge Check
A rod of length l rotates with a uniform angular velocity omega about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the two ends of the lrod is
ABl2ω2BzeroC(Bl2ω8)D2Bl2ω
A conducing rod of length l is falling with a velocity v perpendicular to a unifrorm horizontal magnetic field B . The potential difference between its two ends will be
View SolutionA metal rod of length 2 m is rotating with an angualr velocity of 100rads−1 in plane perpendicular to a uniform magnetic field of 0.3 T. The potential difference between the ends of the rod is
View SolutionA long horizontal metallic rod with length along the east-west direction is falling under gravity. The potential difference between its two ends will
View SolutionA conducting rod AB of lemgth 2 l moves through a uniform magnetic field B. The rod makes an angle θ with the vertical while it falls with a uniform linear velocity v. The potential difference between the two ends of the rod is Bv2l .

View SolutionA rod of length l rotates with a uniform angular velocity omega about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the two ends of the lrod is
View SolutionA rod of length l rotates with a small but uniform angular velocity ω about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the centre of the rod and an end is
View SolutionA metallic rod of length I rotates at angular velocity ω about an axis passing through one end and perpendicular to the rod. If mass of electron is m and its charge is -e then the magnitude of potential difference between its two ends is
View Solution
