In determination of young modulus of elasticity of wire, a force is applied and extension is recorded. Initial length of wire 1m . The curve between extension and stress is depicted then young modulus of wire will be:
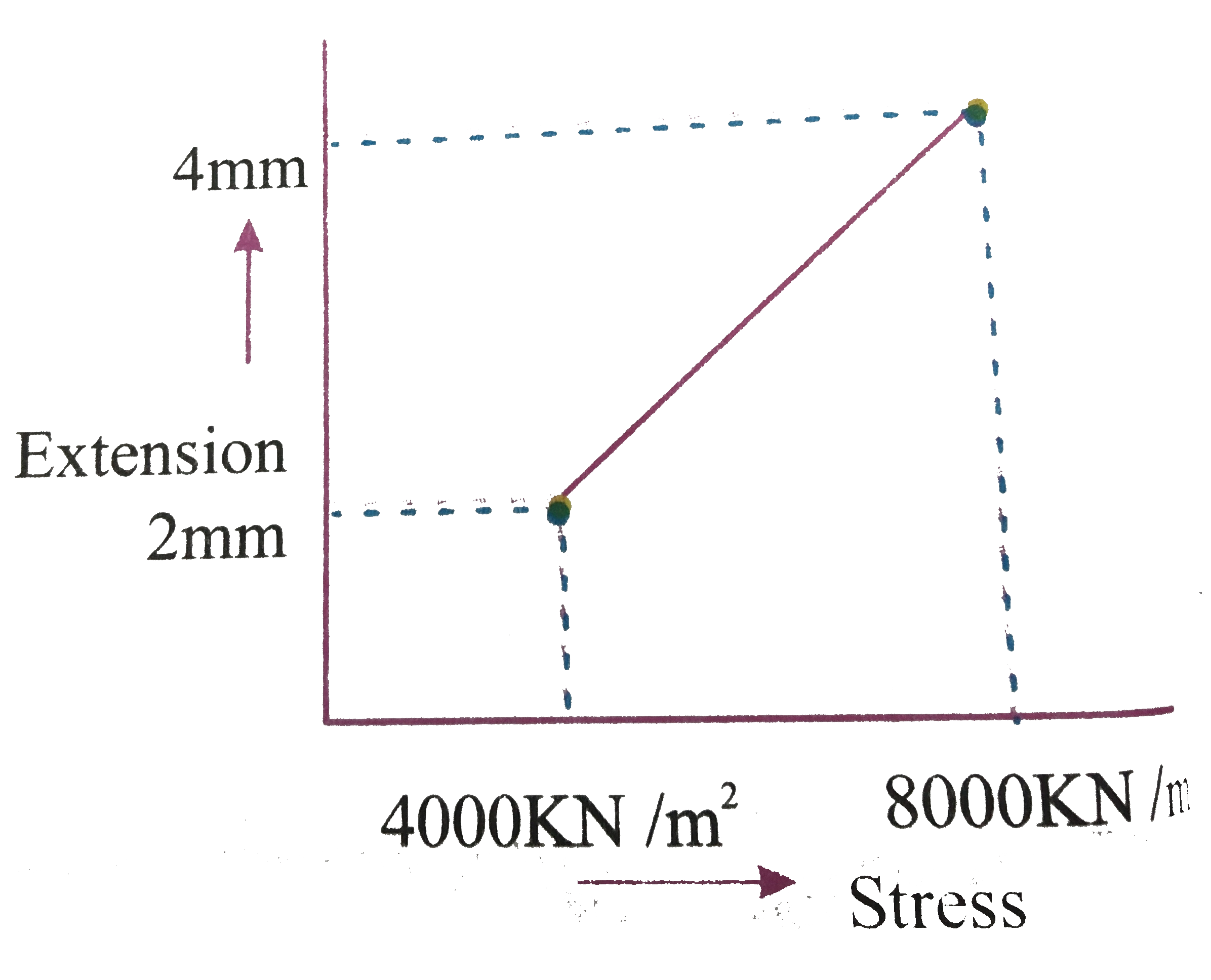
2×109N/m2
1×109N/m2
2×1010N/m2
1×1010N/m2
The correct Answer is:A
Δ1=FlAy,Δl(F/A)=ly=slope of curve
ly=(4−2)×10−3400×103
Given 1=1m→y=4000×1032×10−3
=2×109N/m2
Topper's Solved these Questions
- FLUID MECHANICSBook:RESONANCE ENGLISHChapter:FLUID MECHANICSExercise:Examples
Explore 22 Videos
- FLUID MECHANICSBook:RESONANCE ENGLISHChapter:FLUID MECHANICSExercise:Board Level Exercise
Explore 18 Videos
- ELECTROSTATICSBook:RESONANCE ENGLISHChapter:ELECTROSTATICSExercise:Exercise
Explore 52 Videos
- FULL TEST 1Book:RESONANCE ENGLISHChapter:FULL TEST 1Exercise:Exercise
Explore 30 Videos
Similar Questions
Knowledge Check
Young's modulus of a wire depends on
Adiameter of the wireBmass hanging from the wireClength of the wireDÂ material of the wireThe temperature of a wire is doubled. The Young's modulus of elasticity
Aalso doubleBbecome four timesCremain sameDdecrease.If 'S' is stress and 'Y' is young's modulus of material of a wire, the energy stored in the wire per unit volume is
AS2YB2YS2CS22YD2S2Y
The Young's modulus of a wire is numerically equal
View SolutionA force F doubles the length of wire of cross-section a The Young modulus of wire is
View SolutionThe temperature of a wire is doubled. The Young's modulus of elasticity
View SolutionFor the experimental determination of Young.s modulus of elasticity Y of the material of a given wire by using Searle.s apparatus, the spherometer reading while load increasing and decreasing are tabulated below:
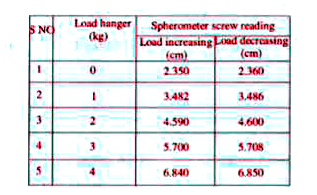
The mean extension for 2kg load is
View SolutionShow that the Young.s modulus of the material of a wire is equal to the stress required to double the initial length of the wire. Assume that Hookes law holds good throughout the process.
View Solution
