A container of cross-section area 'S' and height 'h' is filled with mercury up to the brim. Then the container is sealed airtight and a hole of small cross section area 'S/n' (where 'n' is a positive constant) is puched in its bottom. Find out the time interval upto which the mercury will come out from the bottom hole.
[Take the atmospheric pressure to be equal to h0 height of mercury column: h>h0]
The correct Answer is:14T∫0dt;t=n√2g(h−h0)
To solve the problem, we need to find the time interval during which mercury will flow out of the hole at the bottom of the sealed container. We will use principles from fluid mechanics, particularly Bernoulli's theorem and the relationship between the velocities of the fluid.
1. Understanding the System:
- We have a container with cross-sectional area S and height h filled with mercury.
- A small hole with cross-sectional area Sn is punched at the bottom.
- The container is sealed, so the pressure at the top is constant.
2. Velocity Relationship:
- Let V1 be the velocity at which the mercury level decreases (the velocity of mercury inside the container).
- Let V2 be the velocity of mercury flowing out of the hole.
- By the principle of conservation of mass, we have:
S⋅V1=Sn⋅V2⟹V2=n⋅V1
3. Applying Bernoulli's Theorem:
- We apply Bernoulli's theorem at two points:
- Point 1: At height y inside the container.
- Point 2: At the hole at the bottom.
- The equation becomes:
P1+12ρV21+ρgy=P2+12ρV22+ρg⋅0
- Since the container is sealed, the pressure at the top P1 is 0. The atmospheric pressure at the hole P2 is given as ρgh0:
0+12ρV21+ρgy=ρgh0+12ρV22
4. Simplifying the Equation:
- Rearranging the equation gives:
12ρV21+ρgy=ρgh0+12ρV22
- Canceling ρ from all terms, we have:
12V21+gy=gh0+12V22
5. Substituting for V2:
- Substitute V2=nV1:
12V21+gy=gh0+12(nV1)2
- This simplifies to:
12V21+gy=gh0+12n2V21
- Rearranging gives:
gy−gh0=12(n2−1)V21
6. Finding V1:
- Solving for V21:
V21=2g(y−h0)n2−1
- Thus,
V1=√2g(y−h0)n2−1
7. Relating V1 to Height Change:
- Since V1=−dydt:
−dydt=√2g(y−h0)n2−1
- Rearranging gives:
dt=−dy√2g(y−h0)n2−1
8. Integrating:
- Integrate from y=h to y=h0:
t=−√n2−12g∫h0hdy√y−h0
- The integral evaluates to:
t=−√n2−12g(2√y−h0)h0h
- This gives:
t=2√n2−12g(√h−h0)
9. Final Result:
- Therefore, the time interval t during which the mercury will come out from the bottom hole is:
t=2√n2−12g√h−h0
- We have a container with cross-sectional area S and height h filled with mercury.
- A small hole with cross-sectional area Sn is punched at the bottom.
- The container is sealed, so the pressure at the top is constant.
- Let V1 be the velocity at which the mercury level decreases (the velocity of mercury inside the container).
- Let V2 be the velocity of mercury flowing out of the hole.
- By the principle of conservation of mass, we have:
S⋅V1=Sn⋅V2⟹V2=n⋅V1
- We apply Bernoulli's theorem at two points:
- Point 1: At height y inside the container.
- Point 2: At the hole at the bottom.
- The equation becomes:
P1+12ρV21+ρgy=P2+12ρV22+ρg⋅0
- Since the container is sealed, the pressure at the top P1 is 0. The atmospheric pressure at the hole P2 is given as ρgh0:
0+12ρV21+ρgy=ρgh0+12ρV22
- Rearranging the equation gives:
12ρV21+ρgy=ρgh0+12ρV22
- Canceling ρ from all terms, we have:
12V21+gy=gh0+12V22
- Substitute V2=nV1:
12V21+gy=gh0+12(nV1)2
- This simplifies to:
12V21+gy=gh0+12n2V21
- Rearranging gives:
gy−gh0=12(n2−1)V21
- Solving for V21:
V21=2g(y−h0)n2−1
- Thus,
V1=√2g(y−h0)n2−1
- Since V1=−dydt:
−dydt=√2g(y−h0)n2−1
- Rearranging gives:
dt=−dy√2g(y−h0)n2−1
- Integrate from y=h to y=h0:
t=−√n2−12g∫h0hdy√y−h0
- The integral evaluates to:
t=−√n2−12g(2√y−h0)h0h
- This gives:
t=2√n2−12g(√h−h0)
- Therefore, the time interval t during which the mercury will come out from the bottom hole is:
t=2√n2−12g√h−h0
Topper's Solved these Questions
Similar Questions
Consider the barometer shown in figure. Density of mercury is ρ. A small hole is made at point S as shown. The mercury come out from this hole with speed v equal to
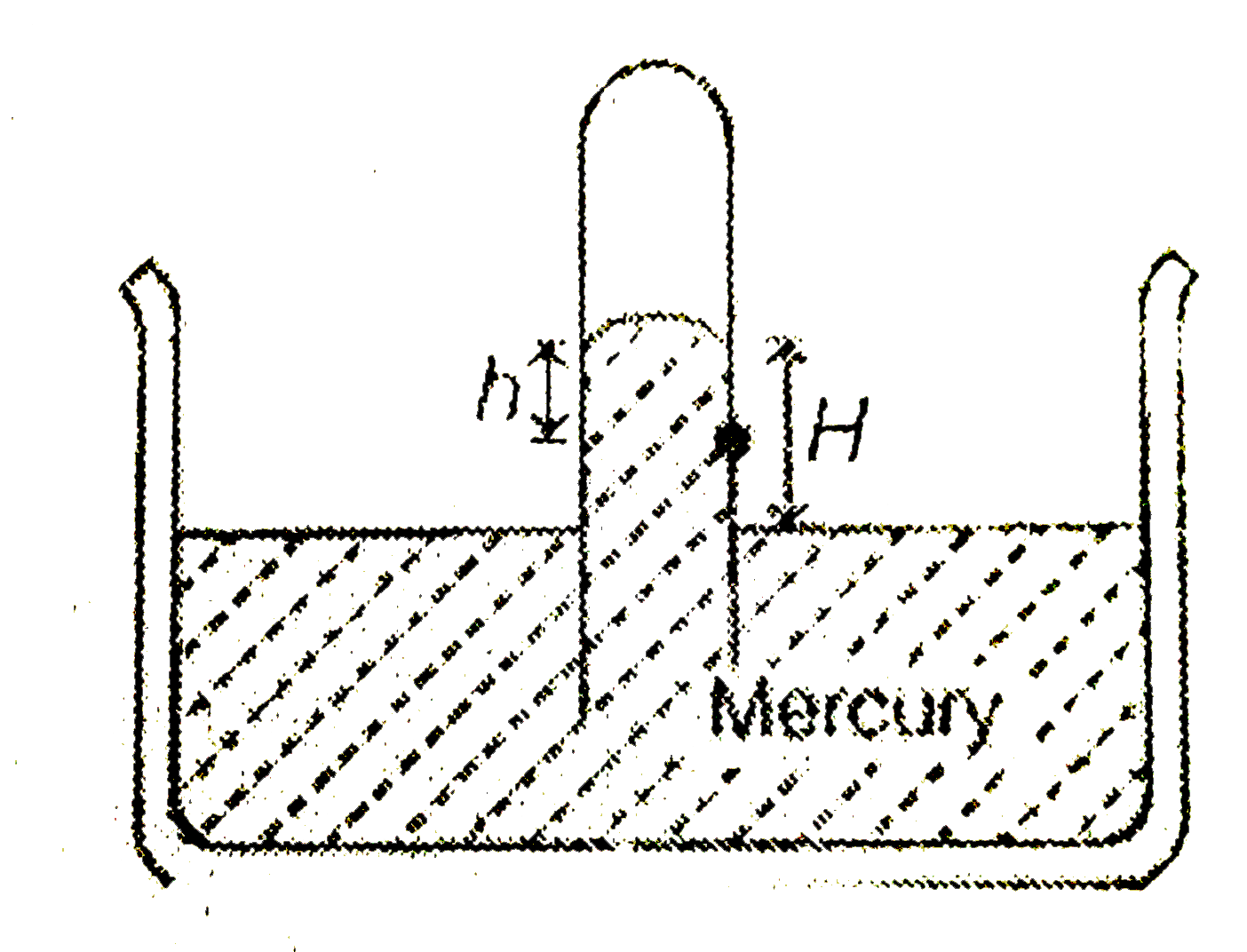
View SolutionConsider the barometer shown in figure. If a small hole is made at a point P in the barometer tube, will the mercury come out from this hole?

View Solution
Knowledge Check
A tank filled with fresh water has a hole in its bottom and water is flowing out of it. If the size of the hole is increased, then
Athe volume of water flowing out per second will decreaseBthe velocity of outflow of water remains unchangedCthe volume of water volume out per second remains zeroDBoth (b) and (c)
The vertical height of mercury in a simple barometer is dependant on the area of cross-section of barometer tube.
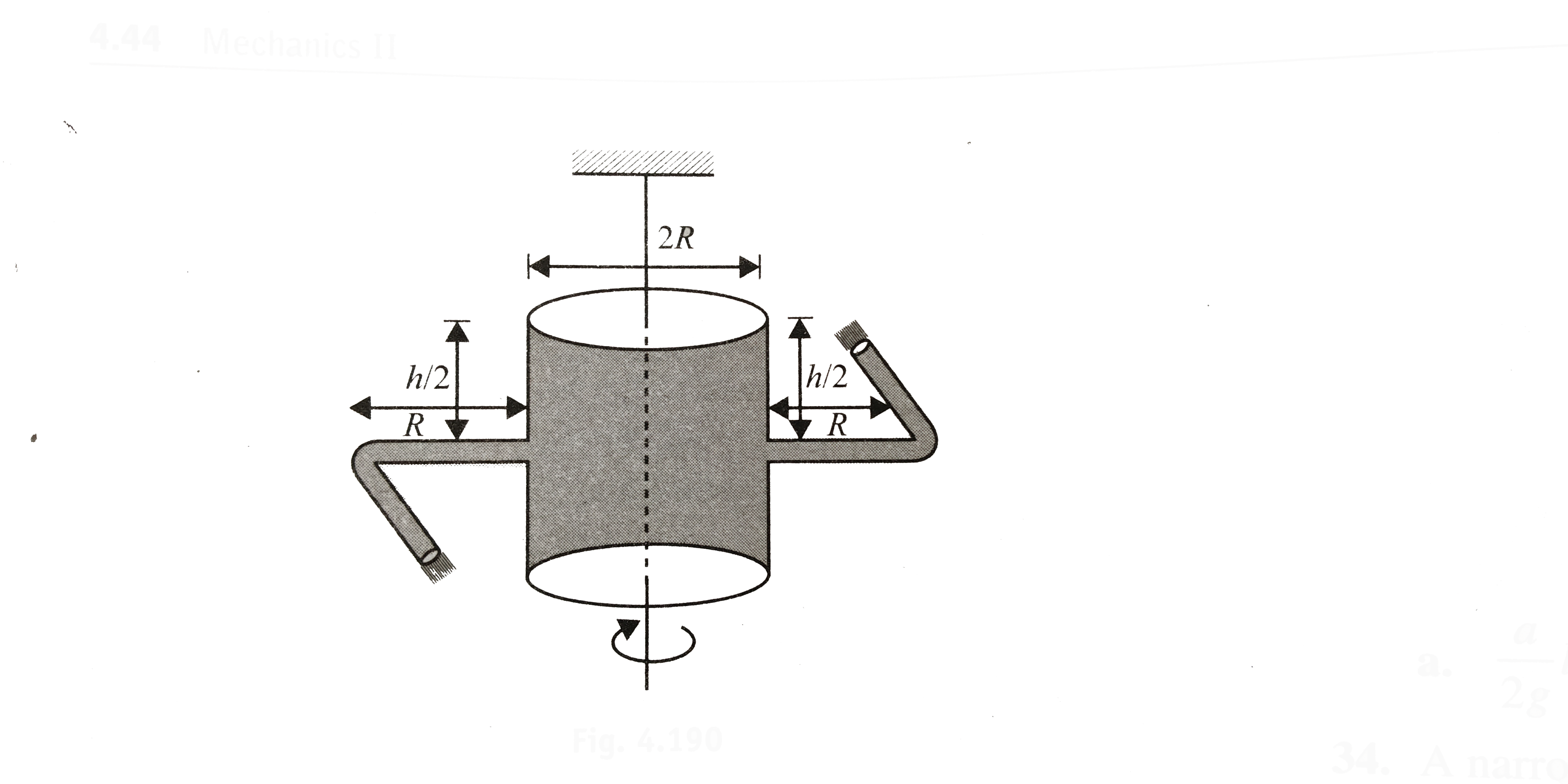
View SolutionA cylindrical container of radius 'R' and height 'h' is completely filled with a liquid. Two horizontal L-shaped pipes of small cross-sectional area 'a' are connected to the cylinder as shown in the figure. Now the two pipes are opened and fluid starts coming out of the pipes horizontally in opposite directions. Then the torque due to ejected liquid on the system is
View SolutionA cylindrical vessel contains a liquid of density ρ up to height h. The liquid is closed by a piston of mass m and area of cross section A. There is a small hole at the bottom of the vessel. The speed v with which the liquid comes out of the hole is

View SolutionA cylindrical vessel contains a liquid of density ρ up to height h. The liquid is closed by a piston of mass m and area of cross section A. There is a small hole at the bottom of the vessel. The speed v with which the liquid comes out of the hole is

View SolutionWater is filled in a container upto height 3m. A small hole of area 'a' is punched in the wall of the container at a height 52.5 cm from the bottom. The cross sectional area of the container is A. If a/A=0.1 then v2 is (where v is the velocity of water coming out of the hole)
View SolutionA beaker of circular cross-section of radius 4 cm is filled with mercury up to a height of 10 cm. Find the force exerted by the mercury on the bottom of the beaker. The atmospheric pressure =105Nm−2. Density of mercury =13600kgm−3.Takeg10ms−2
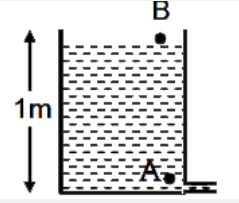
View SolutionA container of height 10 cm is filled with water. There is hole at bottom. Find the pressure difference between point A & B .
View Solution