A smooth tube of certain mass is rotated in a gravity-free Space and released. The two balls shown in Fig move towards the ends of the tube. For the whole system, which of the following quantities is not conserved.
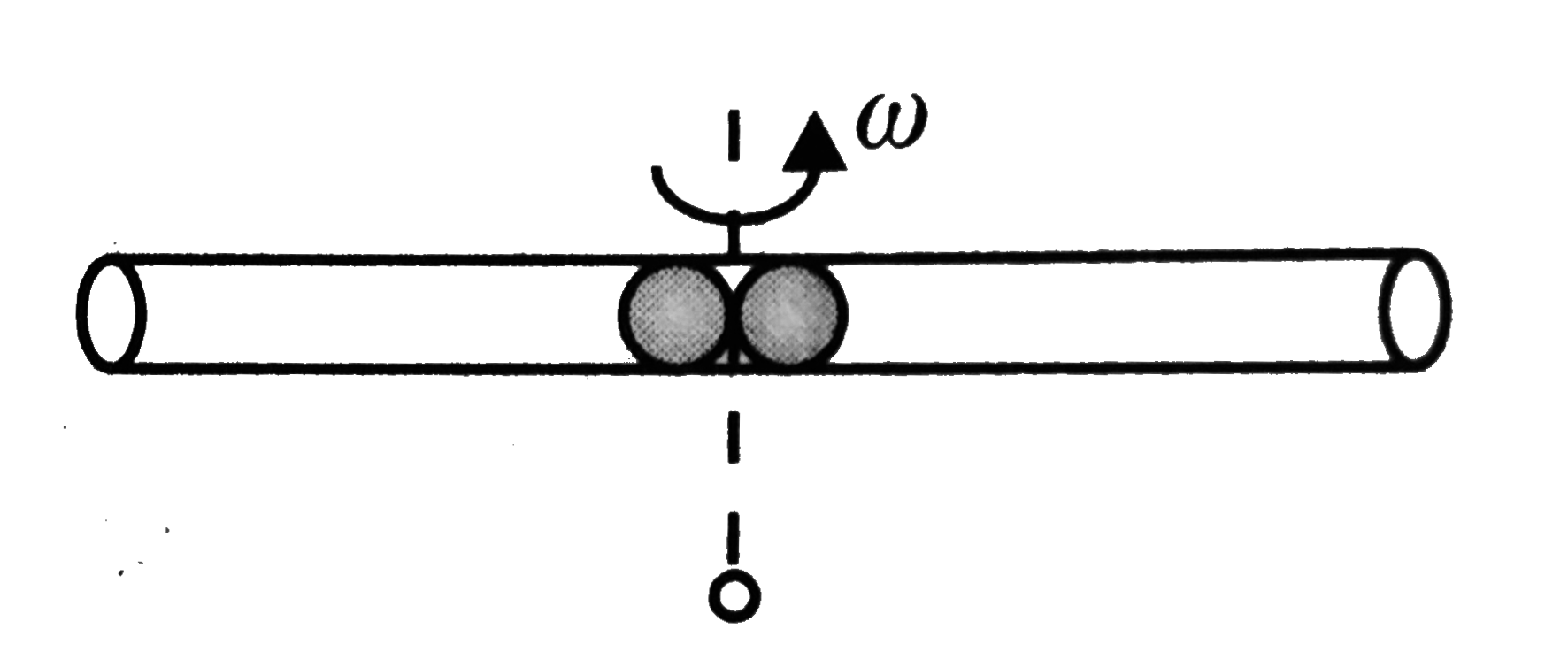
Angular momentum
Linear momentum
Kinetic energy
Angular speed
The correct Answer is:D
As Στ=0 , Angular momentum, linear momentum remains conserved.
As the two balls will move radially out, I changes. In order to keep to the angular momentum (L=Iω) conserved, angular speed (ω) should change. Hence.(D)
Topper's Solved these Questions
Similar Questions
Two point masses m1 and m2 at rest in gravity free space are released from distance d. Find the velocity of the CM of the system at the time of collision of particles
View SolutionA ball of mass m is attached to a string whose other end is fixed. The system is free to rotate in vertical plane. When the ball swings, no work is done on the ball by the tension in the string. Which of the following statement is the correct explanation?
View SolutionA particle moves in a gravity free space where an electric field of strength E and a magnetic field of induction B exit. Which of the following statement is are correct?
View SolutionA block of mass m slides down a wedge of mass M as shown . The whole system is at rest , when the height of the block is h above the ground. The wedge surface is smooth and gradually flattens. There is no friction between wedge and ground.
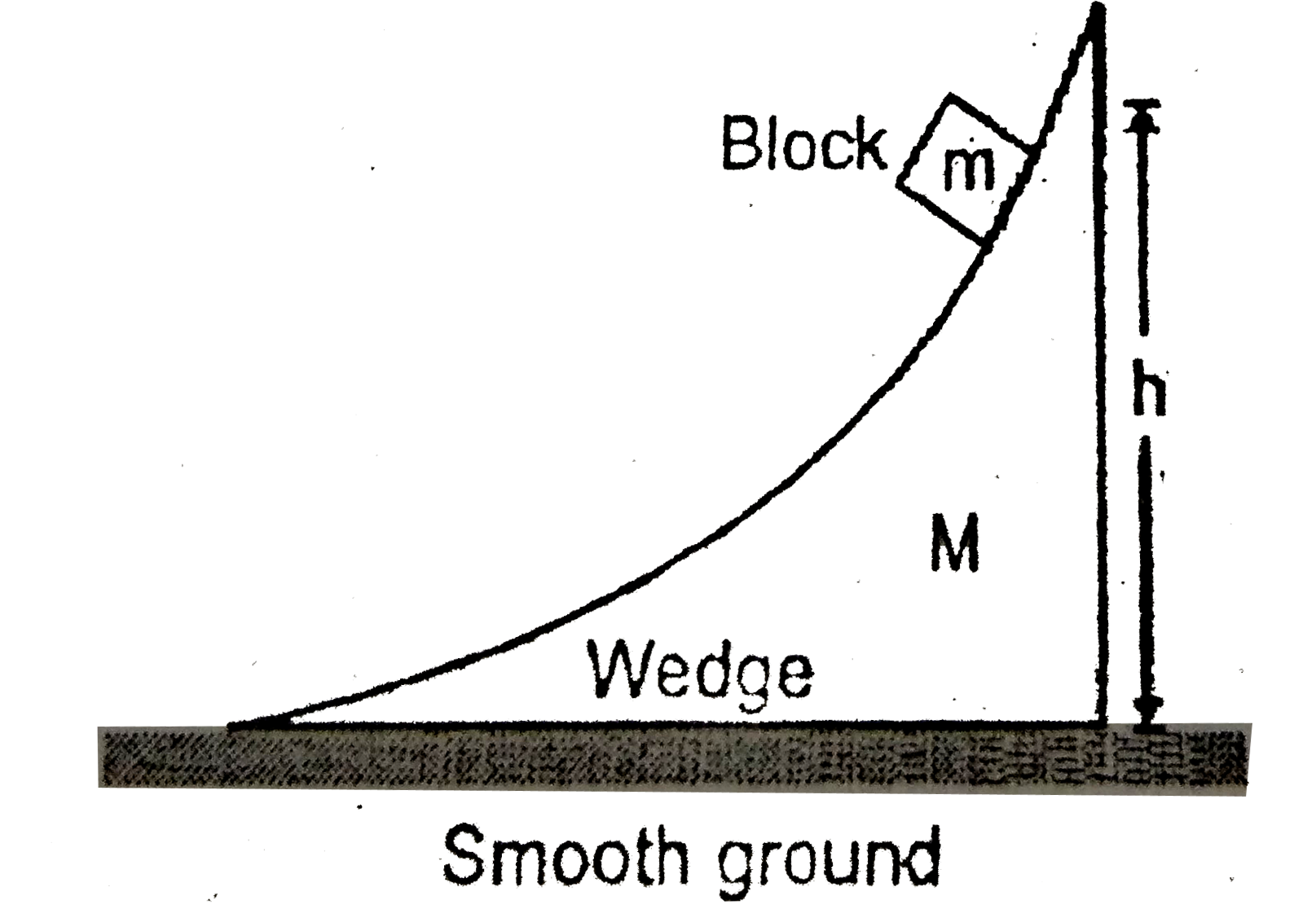
If there would have been friction wedge and block , which of the following quantities would still remains conserved ?
View SolutionA block of mass m slides down a wedge of mass M as shown . The whole system is at rest , when the height of the block is h above the ground. The wedge surface is smooth and gradually flattens. There is no friction between wedge and ground.
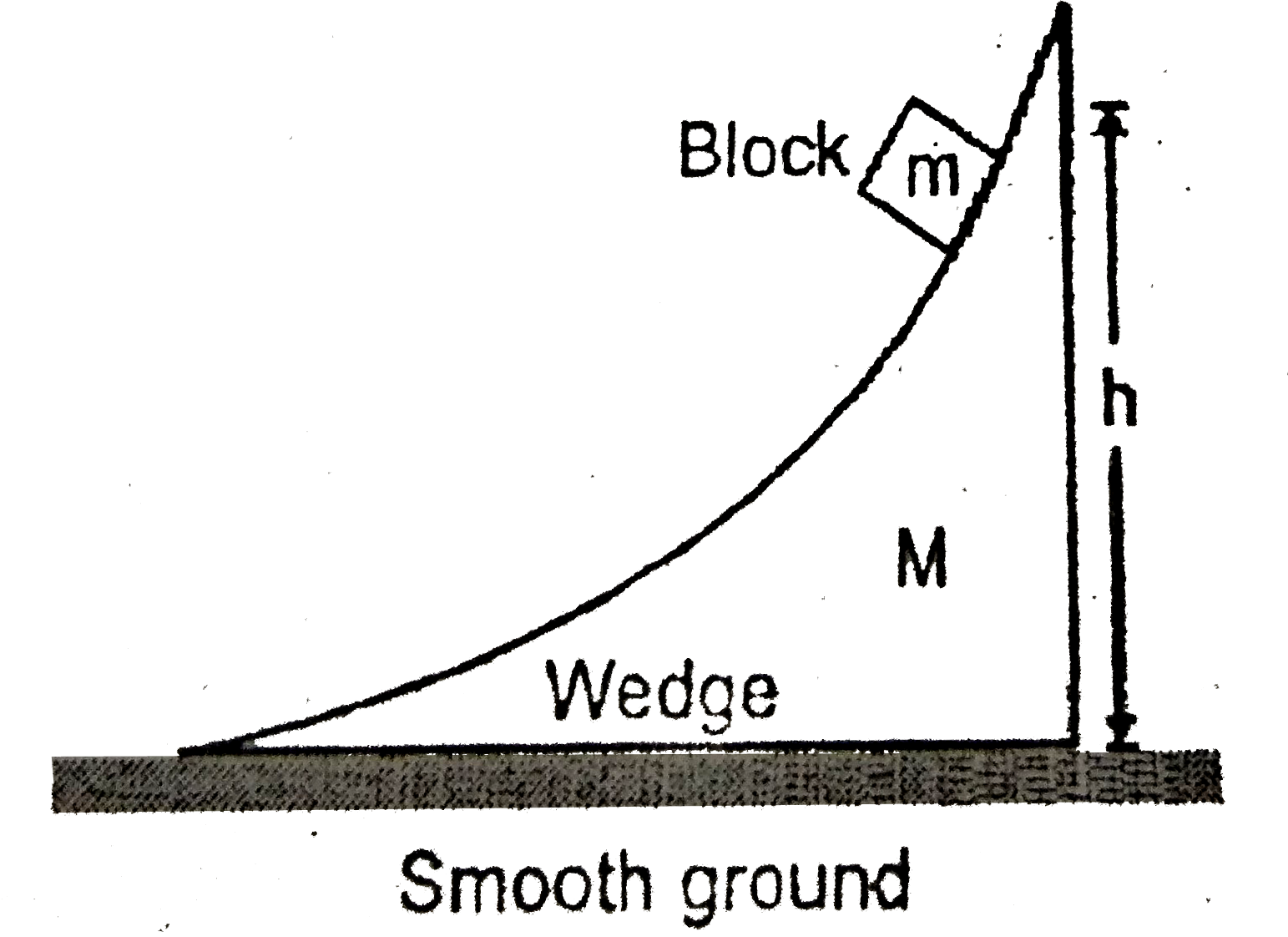
As the block slides down, which of the following quantities associated with the system remains conserved ?
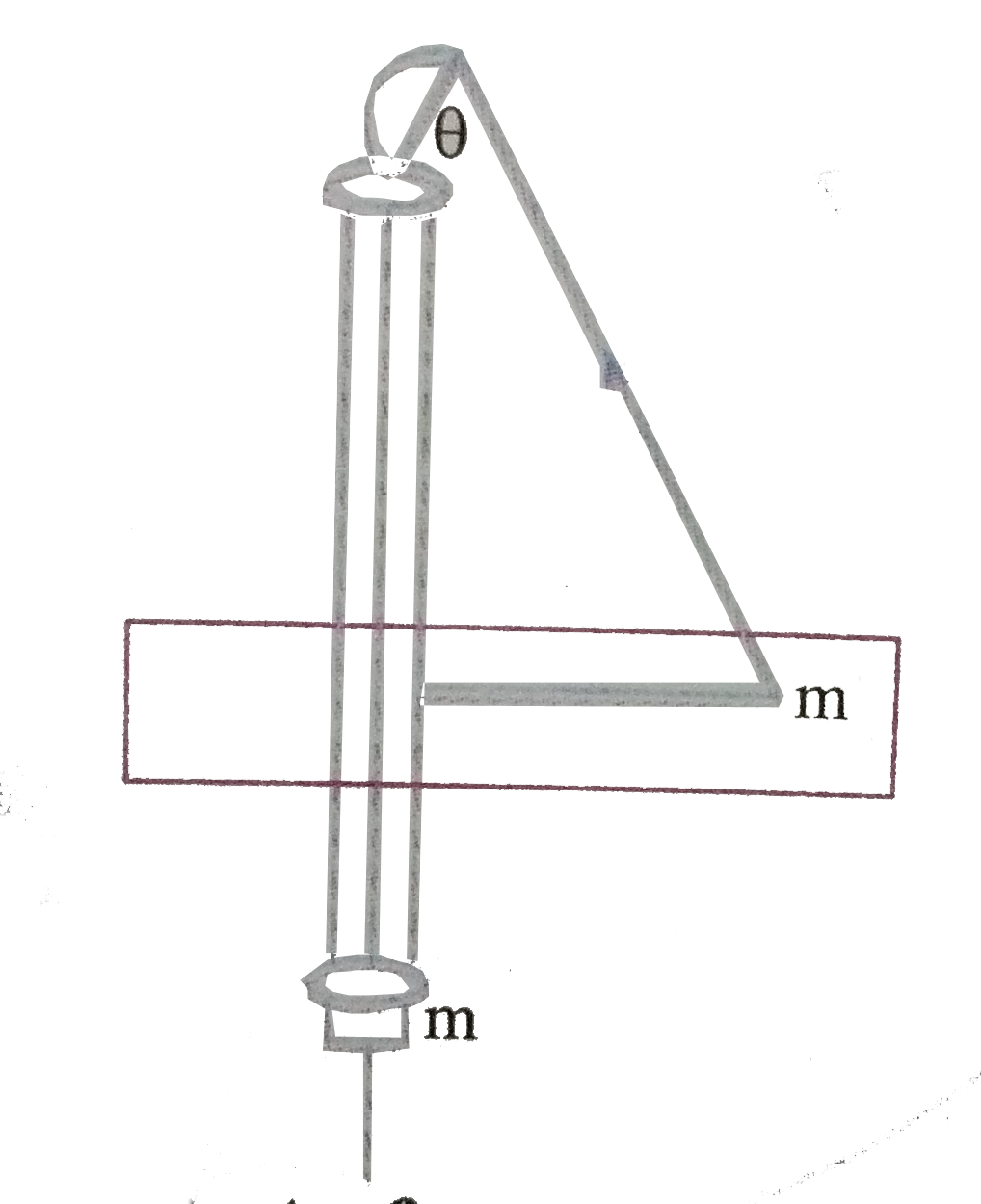
View SolutionA large mass M and a small mass m hang at the two ends of string that passes through a smooth tube as shown in fig. The mass m moves around in a circular path which lies in a horizantal plane. The length of the string from the mass m to the top of the tube is of length l and θ is the angle this length makes with the vertical, what should be the frequency of rotation of the mass m so that M remains stationary if M=16kg,m=4kg,l=1m and g=π2m/s2
View SolutionIn Fig., a hollow tube of mass M is free in horizontal direction. The system is released from rest. There is no friction present. The tube and blocks are taken as system.
i. Momentum of the system is conserved in x-direction.
ii. Speed of A w.r.t. M= speed of B w.r.t. M.
iii. Trajectory of centre of mass is X-constant. iv. Centre of mass has finite acceleration.
Evaluate the above statements and choose the correct option from the following:
Statements i, ii are true and iii, iv are false.
Statements i, ii are false and iii, iv are true.
All statements are true.
All statements are false.

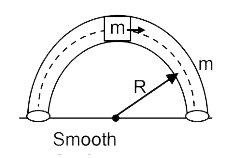
View SolutionIn a vertical smooth hollow thin tube, a block of same mass as that of tube is released as shown. When it is slightly disturbed it moves towards right. By the time the block reaches the right end of the tube, the displacement of the tube will be (where ‘R’ is the mean radius of tube, assume that the tube remains in vertical plane).
View SolutionWhich one of the following is correctly matched ?
View SolutionA small ball of mass m is released in a smooth circular tube as shown in figure. The minimum height h such that bead can complete the circle is
View Solution
