S1: Net torque on a system due to all internal force about any point is zero.
S2: For rigid body undergoing fixed axis rotation, the direction of its angular acceleration and angular velocity
S3: if net torque on a rigid body about its centre of mass is zero, the angular speed of the rigid body is always zero.
S4: For a rigid body undergoing fixed axis rotation abouts its centre of mass, then net torque on rigid body about any point is same.
TFFF
TTFT
FFFF
TFFT
The correct Answer is:D
S1: The line of action of each action reaction pair is same. Therefore magnitude of couple of each such pair is zero. Hence net torque of all internal force is zero.
S2: if net torque on a rigid body is zero, its angular velocity will be constant. the constant may necessary down.
S3: If net torque on a rigid body is zero, its angular velocity will be constant. the constant may necessary not zero.
S4: since the centre of mass is fixed, that is, at rest, hence net force on rigid body is zero. therefore torque on this rigid body any point is same.
Topper's Solved these Questions
Similar Questions
Knowledge Check
Total angular momentum of a rotating body remains constant, if the net torque acting on the body is
AzeroBmaximumCminimumDunity
In net force on a rigid body is zero. Then, match the following table.
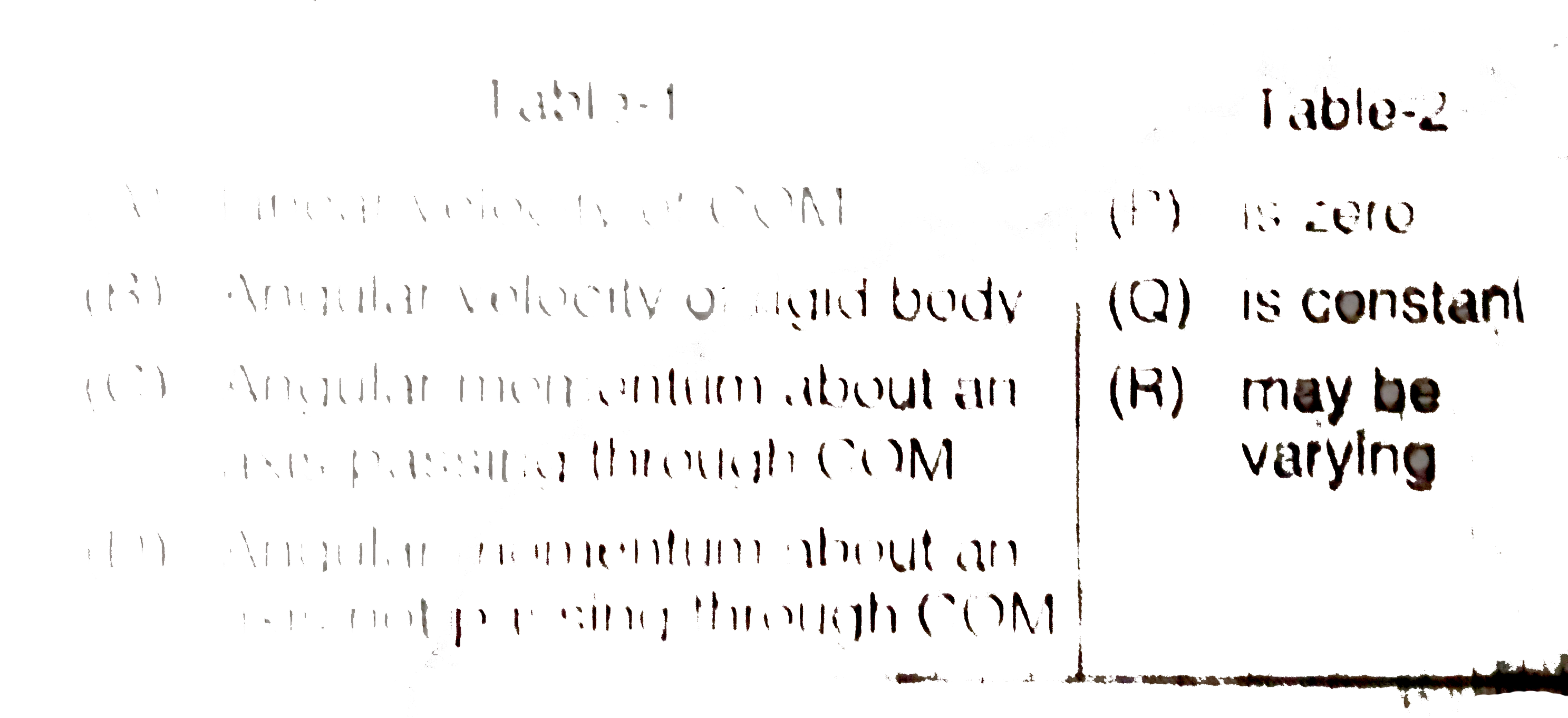
View SolutionA rigid body is in pure rotation, that is, undergoing fixed axis rotation. Then which of the following statement(s) are true?
View SolutionA rigid body is free to rotate about an axis. Can the body have non-zero angulalr acceleration of an instant when its angular velocity is zero?
View SolutionCan you calculate the torque acting on a rigid body without specifying a centre of rotation ?
View SolutionA : For a body to be in rotational equilibrium the net torque acting on the body about any point is zero.
R : For net torque to be zero, net force should also be zero.
View SolutionThe degree of freedom of a stationary rigid body about its axis will be :
View SolutionA : When a rigid body rotates about any fixed axis, then all the particles of it move in circles of different radii but with same angular velocity.
R : In rigid body relative position of particles are fixed.
View Solution